Hostname: page-component-669899f699-rg895 Total loading time: 0 Render date: 2025-04-24T20:47:43.835Z Has data issue: false hasContentIssue false
  • English
  • Français

Convective flows near a solid embedded in a fluid-saturated porous medium heated from above

Published online by Cambridge University Press:  23 December 2024

T.P. Lyubimova Open the ORCID record for T.P. Lyubimova [Opens in a new window] ,
D.V. Lyubimov  and
E.S. Sadilov
T.P. Lyubimova*
Affiliation:
Institute of Continuous Media Mechanics UB RAS, 1, Koroleva Street, Perm 614013, Russia
D.V. Lyubimov
Affiliation:
Perm State University, Bukireva Street, Perm 614068, Russia
E.S. Sadilov
Affiliation:
Institute of Continuous Media Mechanics UB RAS, 1, Koroleva Street, Perm 614013, Russia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

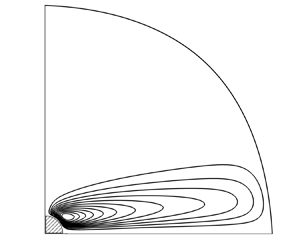
Convective flows near a cylindrical solid inclusion in a fluid-saturated porous medium heated from above are studied using the Darcy–Boussinesq equations. The impermeability condition is imposed on the inclusion surface. Two-dimensional convective flows uniform in the direction of the cylinder axis are considered. An Oseen-like approximation is implemented, however, different from the conventional Oseen approximation, quasilinearization is applied to the nonlinear terms in the energy equation and not in the momentum equation. It is shown that, when the thermal conductivity of the inclusion is higher than that of the fluid, the convective flow at a distance from the inclusion larger than the inclusion size takes the form of horizontal vortices directed away from the inclusion. In the case of low thermal conductivity of the inclusion, the direction of convective circulation is opposite: in the horizontal plane passing through the cylinder axis, the fluid is leaking to the inclusion.

JFM classification

Convection: Convection in porous media Wakes/Jets: Buoyant jets
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 1002 , 10 January 2025 , A5
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Baidulov, V.G., Matyushin, P.V. & Chashechkin, Y.D. 2007 Evolution of the diffusion-induced flow over a sphere submerged in a continuously stratified fluid. Fluid Dyn. 42, 255–267.CrossRefGoogle Scholar
Balasubramaniam, R. & Dill, H. 1991 Thermocapillary bubble migration – an Oseen-like analysis of the energy equation. Microgravity Fluid Mechanics. IUTAM Symposium, Bremen 1991, pp. 307–314. Springer.CrossRefGoogle Scholar
Chen, C.F., Briggs, D.G. & Wirtz, R.A. 1971 Stability of thermal convection in a salinity gradient due to lateral heating. Intl J. Heat Mass Transfer 14, 57–65.CrossRefGoogle Scholar
Dyke, M.V. 1964 Perturbation Methods in Fluid Mechanics. Academic Press.Google Scholar
Eichhorn, R., Lienhard, J.H. & Chen, C.C. 1974 Natural convection from isothermal spheres and cylinders immersed in a stratified fluid. Proceedings of the 5th International Heat Transfer Conference, Tokyo. A.I.Ch.E..CrossRefGoogle Scholar
Gershuni, G.Z. & Zhukhovitsky, E.M. 1976 Convective Stability of Incompressible Fluid. Keter.Google Scholar
Kamakura, K. 1993 Experimental and numerical analyses of double diffusive natural convection heated and cooled from opposing vertical walls with an initial condition of a vertically linear concentration gradient. Intl J. Heat Mass Transfer 36 (8), 2125–2134.CrossRefGoogle Scholar
Khlybov, O.A. 2022 Application of the automatic code generation and symbolic computation techniques to numerical solution of PDE problems. J. Phys.: Conf. Ser. 2317, 012010.Google Scholar
Kodešová, R., et al. 2023 Assessment of potential mobility of selected micropollutants in agricultural soils the Czech Republic using their sorption predicted from soil properties. Sci. Total Environ. 865, 16174.CrossRefGoogle ScholarPubMed
Kodešová, R., et al. 2024 Contamination of water, soil, and plants by micropollutants from reclaimed wastewater and sludge from a wastewater treatment plant. Sci. Total Environ. 927, 167965.CrossRefGoogle Scholar
Lee, J.W. & Hyun, J.M. 1991 Time-dependent double diffusion in a stably stratified fluid under lateral heating. Intl J. Heat Mass Transfer 34 (9), 2409–2421.Google Scholar
Magnani, M., Musacchio, S., Provenzale, A. & Boffetta, G. 2024 Convection in the active layer speeds up permafrost thaw in coarse grained soils. Phys. Rev. Fluids 9, L081501.CrossRefGoogle Scholar
Nield, D.A. & Bejan, A. 2006 Convection in Porous Media. Springer.Google Scholar
Phillips, O.M. 1970 On flows induced by diffusion in a stably stratified fluid. Deep-Sea Res. 17, 435–443.Google Scholar
Prandtl, L. 1952 The Essencials of Fluid Dynamics. Blackie.Google Scholar
Rees, D.A.S. & Nield, D.A. 2016 The effect of an embedded solid block on the onset of convection in a porous cavity. Intl J. Numer. Meth. Heat Fluid Flow 26 (3/4), 950–976.CrossRefGoogle Scholar
Shapiro, A. & Burkholder, F.E. 2012 Analytical and numerical investigation of two-dimensional katabatic flow resulting from local surface cooling. Boundary-Layer Meteorol. 145, 249–272.CrossRefGoogle Scholar
Singh, S.N. 1977 Free convection from a sphere in a slightly-thermally stratified fluid. Intl J. Heat Mass Transfer 20, 1155–1160.CrossRefGoogle Scholar
Sorokin, V.S. 1954 On the stationary motions of a liquid heated from below (in Russian). PMM 18 (2), 197.Google Scholar
Wunsch, C. 1970 On oceanic boundary mixing. Deep-Sea Res. 17, 293–301.Google Scholar