Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-30T20:12:18.814Z Has data issue: false hasContentIssue false
  • English
  • Français

Conditioning of cross-flow instability modes using dielectric barrier discharge plasma actuators

Published online by Cambridge University Press:  02 November 2017

Jacopo Serpieri Open the ORCID record for Jacopo Serpieri [Opens in a new window] ,
Srikar Yadala Venkata Open the ORCID record for Srikar Yadala Venkata [Opens in a new window]  and
Marios Kotsonis
Jacopo Serpieri
Affiliation:
AWEP Department, Section of Aerodynamics Delft University of Technology, Kluyverweg 1, 2629HS Delft, The Netherlands
Srikar Yadala Venkata
Affiliation:
AWEP Department, Section of Aerodynamics Delft University of Technology, Kluyverweg 1, 2629HS Delft, The Netherlands Institut PPRIME, Université de Poitiers (CNRS UPR 3346, ISAE-ENSMA), Boulevard Marie et Pierre Curie, BP 30179, 86962 Futuroscope, France
Marios Kotsonis
Affiliation:
AWEP Department, Section of Aerodynamics Delft University of Technology, Kluyverweg 1, 2629HS Delft, The Netherlands
Get access Rights & Permissions [Opens in a new window]

Abstract

In the current study, selective forcing of cross-flow instability modes evolving on a 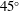$45^{\circ }$ swept wing at 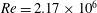$Re=2.17\times 10^{6}$ is achieved by means of spanwise-modulated plasma actuators, positioned near the leading edge. In the perspective of laminar flow control, the followed methodology holds on the discrete roughness elements/upstream flow deformation (DRE/UFD) approach, thoroughly investigated by e.g. Saric et al. (AIAA Paper 1998-781, 1998), Malik et al. (J. Fluid Mech., vol. 399, 1999, pp. 85–115) and Wassermann & Kloker (J. Fluid Mech., vol. 456, 2002, pp. 49–84). The possibility of using active devices for UFD provides several advantages over passive means, allowing for a wider range of operating 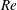$Re$ numbers and pressure distributions. In the present work, customised alternating current dielectric barrier discharge plasma actuators have been designed, manufactured and characterised. The authority of the actuators in forcing monochromatic stationary cross-flow modes at different spanwise wavelengths is assessed by means of infrared thermography. Moreover, quantitative spatio-temporal measurements of the boundary layer velocity field are performed using time-resolved particle image velocimetry. The results reveal distinct steady and unsteady forcing contributions of the plasma actuator on the boundary layer. It is shown that the actuators introduce unsteady fluctuations in the boundary layer, amplifying at frequencies significantly lower than the actuation frequency. In line with the DRE/UFD strategy, forcing a sub-critical stationary mode, with a shorter wavelength compared to the naturally selected mode, results in less amplified primary vortices and related fluctuations, compared to the critical forcing case. The effect of the forcing on the flow stability is further inspected by combining the measured actuators body force with the numerical solution of the laminar boundary layer and linear stability theory. The simplified methodology yields fast and computationally cheap estimates on the effect of steady forcing (magnitude and direction) on the boundary layer stability.

JFM classification

Boundary Layers: Boundary layer control Boundary Layers: Boundary layer stability Flow Control: Instability control
Type
Papers
Information
Journal of Fluid Mechanics , Volume 833 , 25 December 2017 , pp. 164 - 205
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Arnal, D., Gasparian, G. & Salinas, H.1998 Recent advances in theoretical methods for laminar–turbulent transition prediction. AIAA Paper 1998-0223.CrossRefGoogle Scholar
Benard, N. & Moreau, E. 2014 Electrical and mechanical characteristics of surface AC dielectric barrier discharge plasma actuators applied to airflow control. Exp. Fluids 55 (11), 1–43.CrossRefGoogle Scholar
Bippes, H. 1999 Basic experiments on transition in three-dimensional boundary layers dominated by crossflow instability. Prog. Aerosp. Sci. 35 (4), 363–412.CrossRefGoogle Scholar
Bonfigli, G. & Kloker, M. 2007 Secondary instability of crossflow vortices: validation of the stability theory by direct numerical simulation. J. Fluid Mech. 583, 229–272.CrossRefGoogle Scholar
Bridges, T. J. & Morris, P. J. 1984 Differential eigenvalue problems in which the parameter appears nonlinearly. J. Comput. Phys. 55 (3), 437–460.CrossRefGoogle Scholar
Chernyshev, S., Kuryachii, A., Manuilovich, S., Rusyanov, D. & Skvortsov, V.2013 Attenuation of cross-flow-type instability in compressible boundary layer by means of plasma actuators. AIAA Paper 2013-321.CrossRefGoogle Scholar
Corke, T. C., Enloe, C. L. & Wilkinson, S. P. 2010 Dielectric barrier discharge plasma actuators for flow control. Annu. Rev. Fluid Mech. 42, 505–529.CrossRefGoogle Scholar
Deyhle, H. & Bippes, H. 1996 Disturbance growth in an unstable three-dimensional boundary layer and its dependence on environmental conditions. J. Fluid Mech. 316, 73–113.CrossRefGoogle Scholar
Dörr, P. C. & Kloker, M. J. 2015a Numerical investigation of plasma-actuator force-term estimations from flow experiments. J. Phys. D: Appl. Phys. 48 (39), 395203.CrossRefGoogle Scholar
Dörr, P. C. & Kloker, M. J. 2015b Stabilisation of a three-dimensional boundary layer by base-flow manipulation using plasma actuators. J. Phys. D: Appl. Phys. 48, 285205.CrossRefGoogle Scholar
Dörr, P. C. & Kloker, M. J. 2016 Transition control in a three-dimensional boundary layer by direct attenuation of nonlinear crossflow vortices using plasma actuators. Intl J. Heat Fluid Flow 449–465.CrossRefGoogle Scholar
Dörr, P. C. & Kloker, M. J. 2017 Crossflow transition control by upstream flow deformation using plasma actuators. J. Appl. Phys. 121 (6), 063303.CrossRefGoogle Scholar
Dörr, P. C., Kloker, M. J. & Hanifi, A.2017 Effect of upstream flow deformation using plasma actuators on crossflow transition induced by unsteady vortical free-stream disturbances. AIAA Paper 2017-3114.CrossRefGoogle Scholar
Downs, R. S. III & White, E. B. 2013 Free-stream turbulence and the development of cross-flow disturbances. J. Fluid Mech. 735, 347–380.CrossRefGoogle Scholar
Fischer, T. M. & Dallmann, U. 1991 Primary and secondary stability analysis of a three-dimensional boundary-layer flow. Phys. Fluids A 3 (10), 2378–2391.CrossRefGoogle Scholar
Friederich, T. & Kloker, M. J. 2012 Control of the secondary cross-flow instability using localized suction. J. Fluid Mech. 706, 470–495.CrossRefGoogle Scholar
Grundmann, S. & Tropea, C. 2008 Active cancellation of artificially introduced Tollmien–Schlichting waves using plasma actuators. Exp. Fluids 44 (5), 795–806.CrossRefGoogle Scholar
Haynes, T. S. & Reed, H. L. 2000 Simulation of swept-wing vortices using nonlinear parabolized stability equations. J. Fluid Mech. 405, 325–349.CrossRefGoogle Scholar
Högberg, M. & Henningson, D. 1998 Secondary instability of cross-flow vortices in Falkner–Skan–Cooke boundary layers. J. Fluid Mech. 368, 339–357.CrossRefGoogle Scholar
Hosseini, S. M., Tempelmann, D., Hanifi, A. & Henningson, D. S. 2013 Stabilization of a swept-wing boundary layer by distributed roughness elements. J. Fluid Mech. 718, R1-1–R1-11.CrossRefGoogle Scholar
Joslin, R. D. 1998 Aircraft laminar flow control. Annu. Rev. Fluid Mech. 30 (1), 1–29.CrossRefGoogle Scholar
Jukes, T. N. & Choi, K.-S. 2013 On the formation of streamwise vortices by plasma vortex generators. J. Fluid Mech. 733, 370–393.CrossRefGoogle Scholar
Kawakami, M., Kohama, Y. & Okutsu, M.1999 Stability characteristics of stationary crossflow vortices in three-dimensional boundary layer. AIAA Paper 1998-811.CrossRefGoogle Scholar
Kotsonis, M. 2015 Diagnostics for characterisation of plasma actuators. Meas. Sci. Technol. 26 (9), 092001.CrossRefGoogle Scholar
Kotsonis, M., Ghaemi, S., Veldhuis, L. & Scarano, F. 2011 Measurement of the body force field of plasma actuators. J. Phys. D: Appl. Phys. 44 (4), 045204.CrossRefGoogle Scholar
Kotsonis, M., Giepman, R., Hulshoff, S. & Veldhuis, L. 2013 Numerical study of the control of Tollmien–Schlichting waves using plasma actuators. AIAA J. 51 (10), 2353–2364.CrossRefGoogle Scholar
Kotsonis, M., Shukla, R. K. & Pröbsting, S. 2015 Control of natural Tollmien–Schlichting waves using dielectric barrier discharge plasma actuators. Intl J. Flow Control 7 (1–2), 37–54.CrossRefGoogle Scholar
Kurz, H. B. E. & Kloker, M. J. 2014 Receptivity of a swept-wing boundary layer to micron-sized discrete roughness elements. J. Fluid Mech. 755, 62–82.CrossRefGoogle Scholar
Lohse, J., Barth, H. P. & Nitsche, W. 2016 Active control of crossflow-induced transition by means of in-line pneumatic actuator orifices. Exp. Fluids 57 (8), 1–10.CrossRefGoogle Scholar
Mack, L. M.1984 Boundary-layer linear stability theory. AGARD Rep. 709.Google Scholar
Malik, M. R., Li, F., Choudhari, M. & Chang, C.-L. 1999 Secondary instability of crossflow vortices and swept-wing boundary-layer transition. J. Fluid Mech. 399, 85–115.CrossRefGoogle Scholar
Messing, R. & Kloker, M. J. 2010 Investigation of suction for laminar flow control of three-dimensional boundary layers. J. Fluid Mech. 658, 117–147.CrossRefGoogle Scholar
Pereira, R., Kotsonis, M., De Oliveira, G. & Ragni, D. 2015 Analysis of local frequency response of flow to actuation: application to the dielectric barrier discharge plasma actuator. J. Appl. Phys. 118 (15), 103301-1–103301-10.CrossRefGoogle Scholar
Pereira, R., Ragni, D. & Kotsonis, M. 2014 Effect of external flow velocity on momentum transfer of dielectric barrier discharge plasma actuators. J. Appl. Phys. 116 (10), 153301-1–153301-9.CrossRefGoogle Scholar
Radeztsky, R. H., Reibert, M. S. & Saric, W. S. 1999 Effect of isolated micron-sized roughness on transition in swept-wing flows. AIAA J. 37 (11), 1370–1377.CrossRefGoogle Scholar
Raffel, M., Willert, C. E., Wereley, S. T. & Kompenhans, J. 2007 Particle Image Velocimetry. Springer.CrossRefGoogle Scholar
Reibert, M. S., Saric, W. S., Carrillo, R. B. Jr. & Chapman, K.1996 Experiments in nonlinear saturation of stationary crossflow vortices in a swept-wing boundary layer. AIAA Paper 1996-0184.CrossRefGoogle Scholar
Saric, W., Reed, H. & Banks, D.2004 Flight testing of laminar flow control in high-speed boundary layers. NATO-RTO-MP-AVT-111.Google Scholar
Saric, W., Carrillo, R. Jr. & Reibert, M. 1998 Leading-edge roughness as a transition control mechanism. AIAA Paper 1998-781.CrossRefGoogle Scholar
Saric, W. & Reed, H.2002 Supersonic laminar flow control on swept wings using distributed roughness. AIAA Paper 2002-147.Google Scholar
Saric, W. S., Carpenter, A. L. & Reed, H. L. 2011 Passive control of transition in three-dimensional boundary layers, with emphasis on discrete roughness elements. Phil. Trans. R. Soc. Lond A 369 (1940), 1352–1364.Google ScholarPubMed
Saric, W. S., Reed, H. L. & White, E. B. 2003 Stability and transition of three-dimensional boundary layers. Annu. Rev. Fluid Mech. 35 (1), 413–440.CrossRefGoogle Scholar
Scarano, F. 2002 Iterative image deformation methods in PIV. Meas. Sci. Technol. 13 (1), R1.CrossRefGoogle Scholar
Schlichting, H. & Gersten, K. 2000 Boundary Layer Theory. Cambridge University Press.CrossRefGoogle Scholar
Schrijer, F. F. J. & Scarano, F. 2008 Effect of predictor–corrector filtering on the stability and spatial resolution of iterative PIV interrogation. Exp. Fluids 45 (5), 927–941.CrossRefGoogle Scholar
Schuele, C. Y., Corke, T. C. & Matlis, E. 2013 Control of stationary cross-flow modes in a mach 3.5 boundary layer using patterned passive and active roughness. J. Fluid Mech. 718, 5–38.CrossRefGoogle Scholar
Serpieri, J. & Kotsonis, M. 2016 Three-dimensional organisation of primary and secondary crossflow instability. J. Fluid Mech. 799, 200–245.CrossRefGoogle Scholar
Serpieri, J. & Kotsonis, M. 2017 Conditioning of unsteady cross-flow instability modes using AC-DBD plasma actuators. Exp. Therm. Fluid Sci. (under review).Google Scholar
Serpieri, J., Yadala Venkata, S. & Kotsonis, M.2017 Towards laminar flow control on swept wings with AC-DBD plasma actuators as active roughness. AIAA Paper 2017-1459.CrossRefGoogle Scholar
Shahriari, N.2016 On stability and receptivity of boundary-layer flows. PhD Thesis, KTH Royal Institute of Technology, Stockholm, Sweden.Google Scholar
Sirovich, L. 1987 Turbulence and the dynamics of coherent structures. Part i: coherent structures. Q. Appl. Maths 45 (3), 561–571.CrossRefGoogle Scholar
Tucker, A. A., Saric, W. S. & Reed, H. L.2014 Laminar flow control flight experiment design and execution. AIAA Paper 2014-909.CrossRefGoogle Scholar
Wassermann, P. & Kloker, M. 2002 Mechanisms and passive control of crossflow-vortex-induced transition in a three-dimensional boundary layer. J. Fluid Mech. 456, 49–84.CrossRefGoogle Scholar
Wassermann, P. & Kloker, M. 2003 Transition mechanisms induced by travelling crossflow vortices in a three-dimensional boundary layer. J. Fluid Mech. 483, 67–89.CrossRefGoogle Scholar
Welch, P. D. 1967 The use of fast fourier transform for the estimation of power spectra: a method based on time averaging over short, modified periodograms. IEEE Trans. Audio Electroacoust. 15 (2), 70–73.CrossRefGoogle Scholar
White, E. & Saric, W.2000 Application of variable leading-edge roughness for transition control on swept wings. AIAA Paper 2000-283.CrossRefGoogle Scholar
White, E. B. & Saric, W. S. 2005 Secondary instability of crossflow vortices. J. Fluid Mech. 525, 275–308.CrossRefGoogle Scholar