Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-08T05:36:22.921Z Has data issue: false hasContentIssue false
  • English
  • Français

Classical scaling and intermittency in strongly stratified Boussinesq turbulence

Published online by Cambridge University Press:  25 June 2015

Stephen M. de Bruyn Kops Open the ORCID record for Stephen M. de Bruyn Kops [Opens in a new window]
Stephen M. de Bruyn Kops*
Affiliation:
Department of Mechanical and Industrial Engineering, University of Massachusetts Amherst, Amherst, MA 01003-9284, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

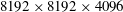Classical scaling arguments of Kolmogorov, Oboukhov and Corrsin (KOC) are evaluated for turbulence strongly influenced by stable stratification. The simulations are of forced homogeneous stratified turbulence resolved on up to $8192\times 8192\times 4096$ grid points with buoyancy Reynolds numbers of 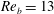$\mathit{Re}_{b}=13$, 48 and 220. A simulation of isotropic homogeneous turbulence with a mean scalar gradient resolved on 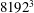$8192^{3}$ grid points is used as a benchmark. The Prandtl number is unity. The stratified flows exhibit KOC scaling only for second-order statistics when 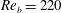$\mathit{Re}_{b}=220$; the 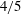$4/5$ law is not observed. At lower $\mathit{Re}_{b}$, the $-5/3$ slope in the spectra occurs at wavenumbers where the bottleneck effect occurs in unstratified cases, and KOC scaling is not observed in any of the structure functions. For the probability density functions (p.d.f.s) of the scalar and kinetic energy dissipation rates, the lognormal model works as well for the stratified cases with $\mathit{Re}_{b}=48$ and 220 as it does for the unstratified case. For lower 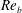$\mathit{Re}_{b}$, the dominance of the vertical derivatives results in the p.d.f.s of the dissipation rates tending towards bimodal. The p.d.f.s of the dissipation rates locally averaged over spheres with radius in the inertial range tend towards bimodal regardless of 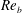$\mathit{Re}_{b}$. 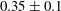There is no broad scaling range, but the intermittency exponents at length scales near the Taylor length are in the range of $0.25\pm 0.05$ and $0.35\pm 0.1$ for the velocity and scalar respectively.

JFM classification

Turbulent Flows: Homogeneous turbulence Turbulent Flows: Stratified turbulence Turbulent Flows: Turbulence simulation
Type
Papers
Information
Journal of Fluid Mechanics , Volume 775 , 25 July 2015 , pp. 436 - 463
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Almalkie, S. & de Bruyn Kops, S. M. 2012a Energy dissipation rate surrogates in incompressible Navier–Stokes turbulence. J. Fluid Mech. 697, 204–236.Google Scholar
Almalkie, S. & de Bruyn Kops, S. M. 2012b Kinetic energy dynamics in forced, homogeneous, and axisymmetric stably stratified turbulence. J. Turbul. 13 (29), 1–29.Google Scholar
Antonia, R. A. & Burattini, P. 2006 Approach to the 4/5 law in homogeneous isotropic turbulence. J. Fluid Mech. 550, 175–184.CrossRefGoogle Scholar
Antonia, R. A. & Sreenivasan, K. R. 1977 Lognormality of temperature dissipation in a turbulent boundary layer. Phys. Fluids 20 (11), 1800–1804.CrossRefGoogle Scholar
Augier, P., Sébastien, G. & Billant, P. 2012 Kolmogorov laws for stratified turbulence. J. Fluid Mech. 709, 659–670.Google Scholar
Bartello, P. & Tobias, S. M. 2013 Sensitivity of stratified turbulence to buoyancy Reynolds number. J. Fluid Mech. 725, 1–22.CrossRefGoogle Scholar
Billant, P. & Chomaz, J.-M. 2001 Self-similarity of strongly stratified inviscid flows. Phys. Fluids 13, 1645–1651.CrossRefGoogle Scholar
Brethouwer, G., Billant, P., Lindborg, E. & Chomaz, J.-M. 2007 Scaling analysis and simulation of strongly stratified turbulent flows. J. Fluid Mech. 585, 343–368.CrossRefGoogle Scholar
Corrsin, S. 1951 On the spectrum of isotropic temperature fluctuations in an isotropic turbulence. J. Appl. Phys. 22, 469–472.Google Scholar
Davidson, P. A. & Pearson, B. R. 2005 The structure of turbulent shear flows. Phys. Rev. Lett. 95, 214501.Google Scholar
Donzis, D. A. & Sreenivasan, K. R. 2010 The bottleneck effect and the Kolmogorov constant in isotropic turbulence. J. Fluid Mech. 657, 171–188.CrossRefGoogle Scholar
Donzis, D. A. & Yeung, P. K. 2010 Resolution effects and scaling in numerical simulations in turbulence. Physica D 239, 1278–1287.Google Scholar
Donzis, D. A., Yeung, P. K. & Sreenivasan, K. R. 2008 Dissipation and enstrophy in isotropic turbulence: resolution effects and scaling in direct numerical simulations. Phys. Fluids 20 (4), 045108.Google Scholar
Durbin, P. A. & Pettersson Reif, B. A. 2010 Statistical Theory and Modeling for Turbulent Flows, 2nd edn. Wiley.CrossRefGoogle Scholar
Gibson, C. H. 1980 Fossil turbulence, salinity, and vorticity turbulence in the ocean. In Marine Turbulence (ed. Nihous, J. C.), pp. 221–257. Elsevier.Google Scholar
Gibson, C. H., Stegen, G. R. & McConnell, S. 1970 Measurements of the universal constant in Kolmogorov’s third hypothesis for high Reynolds number turbulence. Phys. Fluids 13, 2448–2451.CrossRefGoogle Scholar
Godeferd, F. S. & Staquet, C. 2003 Statistical modelling and direct numerical simulations of decaying stably stratified turbulence. Part 2. Large-scale and small-scale anisotropy. J. Fluid Mech. 486, 115–159.Google Scholar
Gotoh, T., Fukayama, D. & Nakano, T. 2002 Velocity field statistics in homogeneous steady turbulence obtained using a high-resolution direct numerical simulation. Phys. Fluids 14 (3), 1065–1081.CrossRefGoogle Scholar
Gregg, M. C. 1987 Diapycnal mixing in the thermocline – a review. J. Geophys. Res. 92, 5249–5286.Google Scholar
Hebert, D. A. & de Bruyn Kops, S. M. 2006a Predicting turbulence in flows with strong stable stratification. Phys. Fluids 18 (6), 1–10.CrossRefGoogle Scholar
Hebert, D. A. & de Bruyn Kops, S. M. 2006b Relationship between vertical shear rate and kinetic energy dissipation rate in stably stratified flows. Geophys. Res. Lett. 33, L06602.CrossRefGoogle Scholar
Hierro, J. & Dopazo, C. 2003 Fourth-order statistical moments of the velocity gradient tensor in homogeneous, isotropic turbulence. Phys. Fluids 15 (11), 3434–3442.Google Scholar
Ishihara, T., Kaneda, Y., Yokokawa, M., Itakura, K. & Uno, A. 2007 Small-scale statistics in high-resolution direct numerical simulation of turbulence: Reynolds number dependence of one-point velocity gradient statistics. J. Fluid Mech. 592, 335–366.Google Scholar
Kaneda, Y., Ishihara, T., Yokokawa, M., Itakura, K. & Uno, A. 2003 Energy dissipation rate and energy spectrum in high resolution direct numerical simulations of turbulence in a periodic box. Phys. Fluids 15 (2), L21–L24.Google Scholar
Kholmyansky, M. & Tsinober, A. 2008 Kolmogorov 4/5 law, nonlocality, and sweeping decorrelation hypothesis. Phys. Fluids 20, 041704.Google Scholar
Kimura, Y. & Herring, J. R. 2012 Energy spectra of stably stratified turbulence. J. Fluid Mech. 698, 19–50.Google Scholar
Kolmogorov, A. N. 1941 Local structure of turbulence in an incompressible fluid at very high Reynolds numbers. Dokl. Akad. Nauk SSSR 30, 299–303.Google Scholar
Kolmogorov, A. N. 1962 A refinement of previous hypotheses concerning the local structure of turbulence in a viscous incompressible fluid at high Reynolds number. J. Fluid Mech. 13, 82–85.Google Scholar
Lin, J.-T. & Pao, Y.-H. 1979 Wakes in stratified fluids: a review. Annu. Rev. Fluid Mech. 11, 317–338.Google Scholar
Lindborg, E. 2006 The energy cascade in a strongly stratified fluid. J. Fluid Mech. 550, 207–242.Google Scholar
Meyers, J. & Meneveau, C. 2008 A functional form for the energy spectrum parametrizing bottleneck and intermittency effects. Phys. Fluids 20 (0), 065109.CrossRefGoogle Scholar
Monin, A. S. & Yaglom, A. M. 1971 Statistical Fluid Mechanics: Mechanics of Turbulence – Volume 1. MIT Press.Google Scholar
Muschinski, A. 2004 Local and global statistics of clear-air Doppler radar signals. Radio Sci. 39, RS1008.CrossRefGoogle Scholar
Mydlarski, L. & Warhaft, Z. 1998 Passive scalar statistics in high Péclet number grid turbulence. J. Fluid Mech. 358, 135–175.Google Scholar
Nichols-Pagel, G. A., Percival, D. B., Reinhall, P. G. & Riley, J. J. 2008 Should structure functions be used to estimate power laws in turbulence? A comparative study. Physica D 237, 665–677.Google Scholar
Novikov, E. A. & Stewart, R. W. 1964 The intermittency of turbulence and the spectrum of energy dissipation. Izv. Akad. Nauk SSSR Geogr. Geoffiz. 3, 408–413.Google Scholar
Oboukhov, A. M. 1941a Spectral energy distribution in a turbulent flow. Dokl. Akad. Nauk SSSR 32, 22–24.Google Scholar
Oboukhov, A. M. 1941b Spectral energy distribution in a turbulent flow. Izv. Akad. Nauk SSSR Geogr. Geofiz 5, 453–466.Google Scholar
Oboukhov, A. M. 1949 Structure of temperature field in a turbulent flow. Izv. Akad. Nauk SSSR Geogr. Geofiz 13, 58.Google Scholar
Oboukhov, A. M. 1962 Some specific features of atmospheric turbulence. J. Fluid Mech. 13, 77–81.Google Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.Google Scholar
Prasad, R., Meneveau, C. & Sreenivasan, K. R. 1988 The multifractal nature of the dissipation of passive scalars in fully turbulent flows. Phys. Rev. Lett. 61, 74–77.Google Scholar
Praskovsky, A. & Oncley, S. 1997 Comprehensive measurements of the intermittency exponent in high Reynolds number turbulent flows. Fluid Dyn. Res. 21 (5), 331–358.Google Scholar
Praud, O., Fincham, A. M. & Sommeria, J. 2005 Decaying grid turbulence in a strongly stratified fluid. J. Fluid Mech. 522, 1–33.Google Scholar
Rao, K. J. & de Bruyn Kops, S. M. 2011 A mathematical framework for forcing turbulence applied to horizontally homogeneous stratified flow. Phys. Fluids 23, 065110.CrossRefGoogle Scholar
Riley, J. J. & de Bruyn Kops, S. M. 2003 Dynamics of turbulence strongly influenced by buoyancy. Phys. Fluids 15 (7), 2047–2059.CrossRefGoogle Scholar
Riley, J. J. & Lindborg, E. 2008 Stratified turbulence: a possible interpretation of some geophysical turbulence measurements. J. Atmos. Sci. 65 (7), 2416–2424.Google Scholar
Rotta, J. C. 1972 Turbulente Strömungen. Teubner.Google Scholar
Saddoughi, S. G. & Veeravalli, S. V. 1994 Local isotropy in turbulence boundary layers at high Reynolds number. J. Fluid Mech. 268, 333–372.CrossRefGoogle Scholar
Shih, L. H., Koseff, J. R., Ivey, G. N. & Ferziger, J. H. 2005 Parameterization of turbulent fluxes and scales using homogeneous sheared stably stratified turbulence simulations. J. Fluid Mech. 525, 193–214.Google Scholar
Siggia, E. D. 1981 Numerical study of small-scale intermittency in three-dimensional turbulence. J. Fluid Mech. 107, 375–406.Google Scholar
Smyth, W. D. & Moum, J. N. 2000 Anisotropy of turbulence in stably stratified mixing layers. Phys. Fluids 12, 1343–1362.Google Scholar
Sreenivasan, K. R. 1984 On the scaling of the turbulence energy-dissipation rate. Phys. Fluids 27 (5), 1048–1051.Google Scholar
Sreenivasan, K. R. 1998 An update on the energy dissipation rate in isotropic turbulence. Phys. Fluids 10 (2), 528–529.Google Scholar
Sreenivasan, K. R. & Antonia, R. A. 1997 The phenomenology of small-scale turbulence. Annu. Rev. Fluid Mech. 29, 435–472.Google Scholar
Sreenivasan, K. R., Antonia, R. A. & Danh, H. Q. 1977 Temperature dissipation fluctuations in a turbulent boundary layer. Phys. Fluids 20 (8), 1238–1249.Google Scholar
Sreenivasan, K. R. & Kailasnath, P. 1993 An update on the intermittency exponent in turbulence. Phys. Fluids 5, 512–514.Google Scholar
Sreenivasan, K. R. & Kailasnath, P. 1996 The passive scalar spectrum and the Obukhov–Corrsin constant. Phys. Fluids 8, 189–196.Google Scholar
Stillinger, D. C., Helland, K. N. & Atta, C. W. V. 1983 Experiments on the transition of homogeneous turbulence to internal waves in a stratified fluid. J. Fluid Mech. 131, 91–122.Google Scholar
Taylor, G. I. 1938 Production and dissipation of vorticity in a turbulent fluid. Proc. R. Soc. Lond. A 164 (919), 15–23.Google Scholar
Tennekes, H. & Lumley, J. L. 1972 A First Course in Turbulence. MIT Press.Google Scholar
Townsend, A. A. 1956 The Structure of Turbulent Shear Flows. Cambridge University Press.Google Scholar
Van Atta, C. W. 1971 Influence of fluctuations in local dissipation rates on turbulent scalar characteristics in the inertial subrange. Phys. Fluids 14, 1803–1804.Google Scholar
Waite, M. L. 2011 Stratified turbulence at the buoyancy scale. Phys. Fluids 23 (6), 066602.Google Scholar
Warhaft, Z. 2000 Passive scalar in turbulent flows. Annu. Rev. Fluid Mech. 32, 203–240.Google Scholar
Yaglom, A. M. 1949 On the local structure of a temperature field in a turbulent flow. Dokl. Akad. Nauk SSSR 69, 743–746.Google Scholar
Yeung, P. K., Donzis, D. A. & Sreenivasan, K. R. 2005 High-Reynolds-number simulation of turbulent mixing. Phys. Fluids 17, 081703.Google Scholar
Yeung, P. K., Xu, S. & Sreenivasan, K. R. 2002 Schmidt number effects on turbulent transport with uniform mean scalar gradient. Phys. Fluids 14 (12), 4178–4191.Google Scholar
Yeung, P. K. & Zhou, Y. 1997 Universality of the Kolmogorov constant in numerical simulations of turbulence. Phys. Rev. E 52 (2), 1746–1752.Google Scholar
Zhou, Q. & Xia, K.-Q. 2010 Universality of local dissipation scales in buoyancy-driven turbulence. Phys. Rev. Lett. 104, 124301.Google Scholar