Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-27T21:04:38.178Z Has data issue: false hasContentIssue false
  • English
  • Français

Characteristics of the turbulent non-turbulent interface in a spatially evolving turbulent mixing layer

Published online by Cambridge University Press:  28 April 2020

G. Balamurugan Open the ORCID record for G. Balamurugan [Opens in a new window] ,
A. Rodda ,
J. Philip  and
A. C. Mandal Open the ORCID record for A. C. Mandal [Opens in a new window]
G. Balamurugan*
Affiliation:
Department of Aerospace Engineering, Indian Institute of Technology, Kanpur208016, India
A. Rodda
Affiliation:
Department of Aerospace Engineering, Indian Institute of Technology, Kanpur208016, India
J. Philip
Affiliation:
Department of Mechanical Engineering, The University of Melbourne, Parkville, VIC 3010, Australia
A. C. Mandal
Affiliation:
Department of Aerospace Engineering, Indian Institute of Technology, Kanpur208016, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

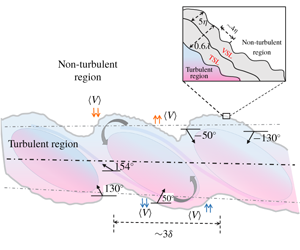
The highly convoluted interface separating the turbulent and non-turbulent regions in a turbulent mixing layer is experimentally investigated using the particle image velocimetry (PIV) technique. The mixing layer was generated using a fine screen/mesh in one half of the test section of a low-speed wind tunnel. The PIV data, which were acquired with high spatial resolution in the self-similar regime of the flow, allow us to identify the turbulent/non-turbulent interface (TNTI) using a suitable threshold value of the absolute spanwise vorticity, $|\unicode[STIX]{x1D714}_{z}|$. The threshold values for the top and bottom interfaces of the mixing layer are found to be different, and the probability density function (PDF) of the interface position for both the interfaces is found to follow the Gaussian distribution. Interestingly, the PDF of the interface orientation reveals two clear peaks, and this is attributed to the sustained large-scale motions in a mixing layer, compared to the other free-shear flows, as is also substantiated by further analyses such as the linear stochastic estimation and the conditional analysis of the transverse velocity profile. The linear stochastic analysis also shows the presence of large vorticity structures of the order of the Taylor microscale at the mean TNTI location in a mixing layer. Furthermore, the present work reveals that, using the spanwise component of vorticity alone, we can experimentally identify and estimate the thickness of the viscous superlayer from the conditional profiles of the diffusion term and the correlation coefficient of the dissipation and the diffusion terms in the enstropy transport equation. The present value of the viscous superlayer thickness of $5\unicode[STIX]{x1D702}$–$6\unicode[STIX]{x1D702}$ (where $\unicode[STIX]{x1D702}$ is the Kolmogorov length scale) compares well with the values reported in the literature for other shear flows. Although both the interfaces are found to behave like a fractal with a dimension of 1.3 in two dimensions, one can find dominant length scales of the order of the thickness of the viscous superlayer, the thickness of the TNTI and the width of the mixing layer from the pre-multiplied power spectra of the autocorrelation functions of the interface curvature, the normal velocity and the interface position, along the TNTI, respectively. In addition, we find that the TNTI characteristics do not show significant dependence on the velocity ratios and $Re_{\unicode[STIX]{x1D706}}$ considered in the present study. Furthermore, the conditional transverse velocity profiles indicate that the entrainment characteristics for the upper and lower TNTIs may be asymmetric in nature.

JFM classification

Turbulent Flows: Shear layer turbulence Mixing: Turbulent mixing Wakes/Jets: Shear layers
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 894 , 10 July 2020 , A4
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adrian, R. J. 2007 Conditional Averages and Stochastic Estimation. In Handbook of Experimental Fluid Mechanics (ed. Tropea, C., Yarin, A. L. & Foss, J. F.), pp. 1370–1378. Springer.Google Scholar
Adrian, R. J., Jones, B. G., Chung, M. K., Hassan, Y., Nithianandan, C. K. & Tung, A. T. C. 1989 Approximation of turbulent conditional averages by stochastic estimation. Phys. Fluids 1 (6), 992–998.CrossRefGoogle Scholar
Anderson, P., LaRue, J. C. & Libby, P. A. 1979 Preferential entrainment in a two-dimensional turbulent jet in a moving stream. Phys. Fluids 22 (10), 1857–1861.CrossRefGoogle Scholar
Attili, A., Cristancho, J. C. & Bisetti, F. 2014 Statistics of the turbulent/non-turbulent interface in a spatially developing mixing layer. J. Turbul. 15 (9), 555–568.CrossRefGoogle Scholar
Balamurugan, G. & Mandal, A. C. 2017 Experiments on localized secondary instability in bypass boundary layer transition. J. Fluid Mech. 817, 217–263.CrossRefGoogle Scholar
Balamurugan, G., Rodda, A., Philip, J. & Mandal, A. C. 2018 Experimental study of turbulent non-turbulent interface in a planar mixing layer using kinetic energy criteria. In 7th International and 45th National Conference on Fluid Mechanics and Fluid Power, IIT Bombay, Mumbai, India.Google Scholar
Bell, J. & Mehta, R. 1990 Development of a two-stream mixing layer from tripped and untripped boundary layers. AIAA J. 28 (12), 2034–2042.CrossRefGoogle Scholar
Bisset, D. K., Hunt, J. C. R. & Rogers, M. M. 2002 The turbulent/non-turbulent interface bounding a far wake. J. Fluid Mech. 451, 383–410.CrossRefGoogle Scholar
Bohl, D. G. & Koochesfahani, M. M. 2009 MTV measurements of the vortical field in the wake of an airfoil oscillating at high reduced frequency. J. Fluid Mech. 620, 63–88.CrossRefGoogle Scholar
Borrell, G. & Jiménez, J. 2016 Properties of the turbulent/non-turbulent interface in boundary layers. J. Fluid Mech. 801, 554–596.CrossRefGoogle Scholar
Brown, G. L. & Roshko, A. 1974 On density effects and large structure in turbulent mixing layers. J. Fluid Mech. 64, 775–816.CrossRefGoogle Scholar
Carlier, J. & Sodjavi, K. 2016 Turbulent mixing and entrainment in a stratified horizontal plane shear layer: joint velocity–temperature analysis of experimental data. J. Fluid Mech. 806, 542–579.CrossRefGoogle Scholar
Champagne, F. H., Pao, Y. H. & Wygnanski, I. J. 1976 On the two-dimensional mixing region. J. Fluid Mech. 74, 209–250.CrossRefGoogle Scholar
Chauhan, K., Philip, J. & Marusic, I. 2014a Scaling of the turbulent/non-turbulent interface in boundary layers. J. Fluid Mech. 751, 298–328.CrossRefGoogle Scholar
Chauhan, K., Philip, J., de Silva, C. M., Hutchins, N. & Marusic, I. 2014b The turbulent/non-turbulent interface and entrainment in a boundary layer. J. Fluid Mech. 742, 119–151.CrossRefGoogle Scholar
Christensen, K. T. & Adrian, R. J. 2001 Statistical evidence of hairpin vortex packets in wall turbulence. J. Fluid Mech. 431, 433–443.CrossRefGoogle Scholar
Coleman, H. & Steele, W. 2009 Experimentation, Validation, and Uncertainty Analysis for Engineers, 3rd edn. Wiley.CrossRefGoogle Scholar
Corrsin, S. & Kistler, A. L.1955 Free-stream boundaries of turbulent flows. NACA Tech. Rep. 1244.Google Scholar
Foss, J. F., Bade, K. M., Neal, D. R., Prevost, R. J. & Morris, S. C. 2017 Single stream shear layer and the viscous super layer. In International Symposium on Turbulence and Shear Flow Phenomena, Chicago, USA, TSFP-10. Begell House.Google Scholar
Fransson, J. H. M., Matsubara, M. & Alfredsson, P. H. 2005 Transition induced by free-stream turbulence. J. Fluid Mech. 527, 1–25.CrossRefGoogle Scholar
Gaster, M., Kit, E. & Wygnanski, I. 1985 Large-scale structures in a forced turbulent mixing layer. J. Fluid Mech. 150, 23–39.CrossRefGoogle Scholar
Girimaji, S. S. 1991 Asymptotic behaviour of curvature of surface elements in isotropic turbulence. Phys. Fluids 3 (7), 1772–1777.CrossRefGoogle Scholar
Girimaji, S. S. & Pope, S. B. 1992 Propagating surfaces in isotropic turbulence. J. Fluid Mech. 234, 247–277.CrossRefGoogle Scholar
Gui, L. & Wereley, S. T. 2002 A correlation-based continuous window-shift technique to reduce the peak-locking effect in digital PIV image evaluation. Exp. Fluids 32 (4), 506–517.CrossRefGoogle Scholar
Holzner, M. & Lüthi, B. 2011 Laminar superlayer at the turbulence boundary. Phys. Rev. Lett. 106 (13), 134503.CrossRefGoogle ScholarPubMed
Jahanbakhshi, R. & Madnia, C. K. 2016 Entrainment in a compressible turbulent shear layer. J. Fluid Mech. 797, 564–603.CrossRefGoogle Scholar
Jahanbakhshi, R. & Madnia, C. K. 2018a The effect of heat release on the entrainment in a turbulent mixing layer. J. Fluid Mech. 844, 92–126.CrossRefGoogle Scholar
Jahanbakhshi, R. & Madnia, C. K. 2018b Viscous superlayer in a reacting compressible turbulent mixing layer. J. Fluid Mech. 848, 743–755.CrossRefGoogle Scholar
Jahanbakhshi, R., Vaghefi, N. S. & Madnia, C. K. 2015 Baroclinic vorticity generation near the turbulent/non-turbulent interface in a compressible shear layer. Phys. Fluids 27 (10), 105105.CrossRefGoogle Scholar
Khashehchi, M., Ooi, A., Soria, J. & Marusic, I. 2013 Evolution of the turbulent/non-turbulent interface of an axisymmetric turbulent jet. Exp. Fluids 54 (1), 1449.CrossRefGoogle Scholar
Kovasznay, L. S. G., Kibens, V. & Blackwelder, R. F. 1970 Large-scale motion in the intermittent region of a turbulent boundary layer. J. Fluid Mech. 41, 283–325.CrossRefGoogle Scholar
Krug, D., Chung, D., Philip, J. & Marusic, I. 2017 Global and local aspects of entrainment in temporal plumes. J. Fluid Mech. 812, 222–250.CrossRefGoogle Scholar
Krug, D., Holzner, M., Lüthi, B., Wolf, M., Kinzelbach, W. & Tsinober, A. 2015 The turbulent/non-turbulent interface in an inclined dense gravity current. J. Fluid Mech. 765, 303–324.CrossRefGoogle Scholar
Kurian, T. & Fransson, J. H. M. 2009 Grid-generated turbulence revisited. Fluid Dynamics Research, vol. 41, (2), p. 021403. IOP Publishing.Google Scholar
Kwon, Y. S., Philip, J., de Silva, C. M., Hutchins, N. & Monty, J. P. 2014 The quiescent core of turbulent channel flow. J. Fluid Mech. 751, 228–254.CrossRefGoogle Scholar
Lourenco, L. & Krothapalli, A. 1995 On the accuracy of velocity and vorticity measurements with PIV. Exp. Fluids 18 (6), 421–428.CrossRefGoogle Scholar
Mandal, A. C., Venkatakrishnan, L. & Dey, J. 2010 A study on boundary layer transition induced by free stream turbulence. J. Fluid Mech. 660, 114–146.CrossRefGoogle Scholar
Mandelbrot, B. B. 1982 The Fractal Geometry of Nature. W. H. Freeman and Company.Google Scholar
Mashayek, A. & Peltier, W. R. 2012 The ‘zoo’ of secondary instabilities precursory to stratified shear flow transition. Part 1. Shear aligned convection, pairing, and braid instabilities. J. Fluid Mech. 708, 5–44.CrossRefGoogle Scholar
Mathew, J. & Basu, A. J. 2002 Some characteristics of entrainment at a cylindrical turbulence boundary. Phys. Fluids 14 (7), 2065–2072.CrossRefGoogle Scholar
Mathew, J., Mahle, I. & Friedrich, R. 2008 Effects of compressibility and heat release on entrainment processes in mixing layers. J. Turbul. 9 (14), 1–12.Google Scholar
Mehta, R. D. 1991 Effect of velocity ratio on plane mixing layer development: influence of the splitter plate wake. Exp. Fluids 10 (4), 194–204.CrossRefGoogle Scholar
Mistry, D., Dawson, J. R., Philip, J. & Marusic, I. 2017 The influence of turbulent/non-turbulent interface geometry on local entrainment. In International Symposium on Turbulence and Shear Flow Phenomena, Chicago, USA, TSFP-10. Begell House.Google Scholar
Mistry, D., Philip, J. & Dawson, J. R. 2019 Kinematics of local entrainment and detrainment in a turbulent jet. J. Fluid Mech. 871, 896–924.CrossRefGoogle Scholar
Mistry, D., Philip, J., Dawson, J. R. & Marusic, I. 2016 Entrainment at multi-scales across the turbulent/non-turbulent interface in an axisymmetric jet. J. Fluid Mech. 802, 690–725.CrossRefGoogle Scholar
Oguchi, H. & Inoue, O. 1984 Mixing layer produced by a screen and its dependence on initial conditions. J. Fluid Mech. 142, 217–231.CrossRefGoogle Scholar
Orfanidis, S. J. 2010 Introduction to Signal Processing. Prentice Hall.Google Scholar
Phani Kumar, P., Mandal, A. C. & Dey, J. 2015 Effect of a mesh on boundary layer transitions induced by free-stream turbulence and an isolated roughness element. J. Fluid Mech. 772, 445–477.CrossRefGoogle Scholar
Philip, J., Bermejo-Moreno, I., Chung, D. & Marusic, I. 2015 Characteristics of the entrainment velocity in a developing wake. In International Symposium on Turbulence and Shear Flow Phenomena, Melbourne, Australia, TSFP-9. Begell House.Google Scholar
Philip, J., Meneveau, C., de Silva, C. M. & Marusic, I. 2014 Multiscale analysis of fluxes at the turbulent/non-turbulent interface in high Reynolds number boundary layers. Phys. Fluids 26 (1), 015105.CrossRefGoogle Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.CrossRefGoogle Scholar
Prasad, R. R. & Sreenivasan, K. R. 1989 Scalar interfaces in digital images of turbulent flows. Exp. Fluids 7 (4), 259–264.CrossRefGoogle Scholar
Raffel, M., Willert, C. E., Scarano, F., Kähler, C. J., Wereley, S. T. & Kompenhans, J. 2018 Particle Image Velocimetry: a Practical Guide. Springer.CrossRefGoogle Scholar
van Reeuwijk, M. & Holzner, M. 2014 The turbulence boundary of a temporal jet. J. Fluid Mech. 739, 254–275.CrossRefGoogle Scholar
Sciacchitano, A. 2019 Uncertainty quantification in particle image velocimetry. Meas. Sci. Technol. 30 (9), 092001.CrossRefGoogle Scholar
da Silva, C. B., Dos Reis, R. J. N. & Pereira, J. C. F. 2011 The intense vorticity structures near the turbulent/non-turbulent interface in a jet. J. Fluid Mech. 685, 165–190.CrossRefGoogle Scholar
da Silva, C. B., Hunt, J. C. R., Eames, I. & Westerweel, J. 2014 Interfacial layers between regions of different turbulence intensity. Annu. Rev. Fluid Mech. 46, 567–590.CrossRefGoogle Scholar
da Silva, C. B. & dos Reis, R. J. N. 2011 The role of coherent vortices near the turbulent/non-turbulent interface in a planar jet. Phil. Trans. R. Soc. Lond. A 369 (1937), 738–753.CrossRefGoogle Scholar
da Silva, C. B. & Taveira, R. R. 2010 The thickness of the turbulent/non-turbulent interface is equal to the radius of the large vorticity structures near the edge of the shear layer. Phys. Fluids 22 (12), 121702.CrossRefGoogle Scholar
de Silva, C. M., Philip, J., Chauhan, K., Meneveau, C. & Marusic, I. 2013 Multiscale geometry and scaling of the turbulent-nonturbulent interface in high Reynolds number boundary layers. Phys. Rev. Lett. 111, 044501.CrossRefGoogle ScholarPubMed
Silva, T. S., Zecchetto, M. & da Silva, C. B. 2018 The scaling of the turbulent/non-turbulent interface at high Reynolds numbers. J. Fluid Mech. 843, 156–179.CrossRefGoogle Scholar
Sreenivasan, K. R. & Meneveau, C. 1986 The fractal facets of turbulence. J. Fluid Mech. 173, 357–386.CrossRefGoogle Scholar
Taveira, R. R. & da Silva, C. B. 2014 Characteristics of the viscous superlayer in shear free turbulence and in planar turbulent jets. Phys. Fluids 26 (2), 021702.Google Scholar
Thielicke, W. & Stamhuis, E. 2014 Pivlab–towards user-friendly, affordable and accurate digital particle image velocimetry in matlab. J. Open Res. Software 2 (1), 1–10.CrossRefGoogle Scholar
Townsend, A. A. 1980 The Structure of Turbulent Shear Flow. Cambridge University Press.Google Scholar
Watanabe, T., Sakai, Y., Nagata, K., Ito, Y. & Hayase, T. 2014 Vortex stretching and compression near the turbulent/non-turbulent interface in a planar jet. J. Fluid Mech. 758, 754–785.CrossRefGoogle Scholar
Watanabe, T., Sakai, Y., Nagata, K., Ito, Y. & Hayase, T. 2015 Turbulent mixing of passive scalar near turbulent and non-turbulent interface in mixing layers. Phys. Fluids 27 (8), 085109.CrossRefGoogle Scholar
Watanabe, T., da Silva, C. B., Nagata, K. & Sakai, Y. 2017 Geometrical aspects of turbulent/non-turbulent interfaces with and without mean shear. Phys. Fluids 29 (8), 085105.CrossRefGoogle Scholar
Watanabe, T., da Silva, C. B., Sakai, Y., Nagata, K. & Hayase, T. 2016 Lagrangian properties of the entrainment across turbulent/non-turbulent interface layers. Phys. Fluids 28 (3), 031701.CrossRefGoogle Scholar
Watanabe, T., Zhang, X. & Nagata, K. 2018 Turbulent/non-turbulent interfaces detected in DNS of incompressible turbulent boundary layers. Phys. Fluids 30 (3), 035102.CrossRefGoogle Scholar
Westerweel, J., Fukushima, C., Pedersen, J. M. & Hunt, J. C. R. 2005 Mechanics of the turbulent-nonturbulent interface of a jet. Phys. Rev. Lett. 95 (17), 174501.Google ScholarPubMed
Westerweel, J., Fukushima, C., Pedersen, J. M. & Hunt, J. C. R. 2009 Momentum and scalar transport at the turbulent/non-turbulent interface of a jet. J. Fluid Mech. 631, 199–230.CrossRefGoogle Scholar
Westerweel, J., Hofmann, T., Fukushima, C. & Hunt, J. 2002 The turbulent/non-turbulent interface at the outer boundary of a self-similar turbulent jet. Exp. Fluids 33 (6), 873–878.CrossRefGoogle Scholar
Wolf, M., Holzner, M., Lüthi, B., Krug, D., Kinzelbach, W. & Tsinober, A. 2013 Effects of mean shear on the local turbulent entrainment process. J. Fluid Mech. 731, 95–116.CrossRefGoogle Scholar