Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-24T16:01:02.891Z Has data issue: false hasContentIssue false
  • English
  • Français

Capillary effects on wave breaking

Published online by Cambridge University Press:  25 March 2015

Luc Deike ,
Stephane Popinet  and
W. Kendall Melville
Luc Deike*
Affiliation:
Scripps Institution of Oceanography, University of California San Diego, La Jolla, CA 92093, USA National Institute for Water and Atmospheric Research, P.O. Box 14901, Kilbirnie, Wellington 6003, New Zealand
Stephane Popinet
Affiliation:
National Institute for Water and Atmospheric Research, P.O. Box 14901, Kilbirnie, Wellington 6003, New Zealand Sorbonne Universités, UPMC Univ Paris 06, CNRS, UMR 7190 Institut Jean Le Rond d’Alembert, F-75005 Paris, France
W. Kendall Melville
Affiliation:
Scripps Institution of Oceanography, University of California San Diego, La Jolla, CA 92093, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

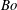We investigate the influence of capillary effects on wave breaking through direct numerical simulations of the Navier–Stokes equations for a two-phase air–water flow. A parametric study in terms of the Bond number, $\mathit{Bo}$, and the initial wave steepness, ${\it\epsilon}$, is performed at a relatively high Reynolds number. The onset of wave breaking as a function of these two parameters is determined and a phase diagram in terms of 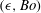$({\it\epsilon},\mathit{Bo})$ is presented that distinguishes between non-breaking gravity waves, parasitic capillaries on a gravity wave, spilling breakers and plunging breakers. At high Bond number, a critical steepness 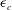${\it\epsilon}_{c}$ defines the onset of wave breaking. At low Bond number, the influence of surface tension is quantified through two boundaries separating, first gravity–capillary waves and breakers, and second spilling and plunging breakers; both boundaries scaling as 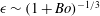${\it\epsilon}\sim (1+\mathit{Bo})^{-1/3}$. Finally the wave energy dissipation is estimated for each wave regime and the influence of steepness and surface tension effects on the total wave dissipation is discussed. The breaking parameter 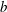$b$ is estimated and is found to be in good agreement with experimental results for breaking waves. Moreover, the enhanced dissipation by parasitic capillaries is consistent with the dissipation due to breaking waves.

JFM classification

Geophysical and Geological Flows: Air/sea interactions Waves/Free-surface Flows: Wave breaking Waves/Free-surface Flows: Waves/Free-surface Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 769 , 25 April 2015 , pp. 541 - 569
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Agbaglah, G., Delaux, S., Fuster, D., Hoepffner, J., Josserand, C., Popinet, S., Ray, P., Scardovelli, R. & Zaleski, S. 2011 Parallel simulation of multiphase flows using octree adaptivity and the volume-of-fluid method. C. R. Méc. 339 (23), 194–207.CrossRefGoogle Scholar
Bague, A., Fuster, D., Popinet, S., Scardovelli, R. & Zaleski, S. 2010 Instability growth rate of two-phase mixing layers from a linear eigenvalue problem and an initial value problem. Phys. Fluids 22 (9), 092104.CrossRefGoogle Scholar
Banner, M. L. & Melville, W. K. 1976 On the separation of air flow over water waves. J. Fluid Mech. 77, 825–842.CrossRefGoogle Scholar
Banner, M. L. & Peirson, W. L. 2007 Wave breaking onset and strength for two-dimensional deep-water wave groups. J. Fluid Mech. 585 (1), 93–115.CrossRefGoogle Scholar
Batchelor, G. K. 1967 An Introduction to Fluid Dynamics. Cambridge University Press.Google Scholar
Blenkinsopp, C. E. & Chaplin, J. R. 2007 Void fraction measurements in breaking waves. Proc. R. Soc. Lond. A 463 (2088), 3151–3170.Google Scholar
Caulliez, G. 2013 Dissipation regimes for short wind waves. J. Geophys. Res. 118 (2), 672–684.CrossRefGoogle Scholar
Caulliez, G., Ricci, N. & Dupont, R. 1998 The generation of the first visible wind waves. Phys. Fluids 10 (4), 757–759.CrossRefGoogle Scholar
Ceniceros, H. D. 2003 The effects of surfactants on the formation and evolution of capillary waves. Phys. Fluids 15 (1), 245–256.CrossRefGoogle Scholar
Chen, G., Kharif, C., Zaleski, S. & Li, J. 1999 Two dimensional Navier–Stokes simulation of breaking waves. Phys. Fluids 11, 121–133.CrossRefGoogle Scholar
Crapper, G. D. 1957 An exact solution for progressive capillary waves of arbitrary amplitude. J. Fluid Mech. 2, 532–540.CrossRefGoogle Scholar
Dias, F. & Kharif, C. 1999 Nonlinear gravity and capillary–gravity waves. Annu. Rev. Fluid Mech. 31, 301–346.CrossRefGoogle Scholar
Douady, S., Couder, Y. & Brachet, M. E. 1991 Direct observation of the intermittency of intense vorticity filaments in turbulence. Phys. Rev. Lett. 67, 983–986.CrossRefGoogle ScholarPubMed
Drazen, D. A., Melville, W. K. & Lenain, L. 2008 Inertial scaling of dissipation in unsteady breaking waves. J. Fluid Mech. 611 (1), 307–332.CrossRefGoogle Scholar
Duncan, J. H. 1981 An experimental investigation of breaking waves produced by a towed hydrofoil. Proc. R. Soc. Lond. A 377 (1770), 331–348.Google Scholar
Duncan, J. H. 2001 Spilling breakers. Annu. Rev. Fluid Mech. 33, 519–547.CrossRefGoogle Scholar
Duncan, J. H., Qiao, H. & Philomin, V. 1999 Gentle spilling breakers: crest profile evolution. J. Fluid Mech. 379, 191–222.CrossRefGoogle Scholar
Fedorov, A. V. & Melville, W. K. 1998 Non linear gravity–capillary waves with forcing dissipation. J. Fluid Mech. 354, 1–42.CrossRefGoogle Scholar
Fedorov, A. V., Melville, W. K. & Rozenberg, A. 1998 An experimental and numerical study of parasitic capillary waves. Phys. Fluids 10 (6), 1315–1323.CrossRefGoogle Scholar
Fenton, J. D. 1988 The numerical solution of steady water wave problems. Comput. Geosci. 14, 357–368.CrossRefGoogle Scholar
Fructus, D., Clamond, D., Grue, J. & Kristiansen, O. 2005 An efficient model for three-dimensional surface wave simulations. Part 1: free space problems. J. Comput. Phys. 205, 685–705.CrossRefGoogle Scholar
Furhman, D. R., Madsen, P. A. & Bingham, H. B. 2004 A numerical study of crescent wave. J. Fluid Mech. 513, 309–341.CrossRefGoogle Scholar
Fuster, D., Agbaglah, G., Josserand, C., Popinet, S. & Zaleski, S. 2009 Numerical simulation of droplets, bubbles and waves: state of the art. Fluid Dyn. Res. 41, 065001.CrossRefGoogle Scholar
Fuster, D., Matas, J.-P., Marty, S., Popinet, S., Hoepffner, J., Cartellier, A. & Zaleski, S. 2013 Instability regimes in the primary breakup region of planar coflowing sheets. J. Fluid Mech. 736, 150–176.CrossRefGoogle Scholar
Grare, L., Peirson, W. L., Branger, H., Walker, J. W., Giovanangeli, J.-P. & Makin, V. 2013 Growth and dissipation of wind-forced, deep-water waves. J. Fluid Mech. 722, 5–50.CrossRefGoogle Scholar
Hornung, H. G., Willert, C. & Turner, S. 1995 The flow field downstream of a hydraulic jump. J. Fluid Mech. 287, 299–316.CrossRefGoogle Scholar
Iafrati, A. 2009 Numerical study of the effects of the breaking intensity on wave breaking flows. J. Fluid Mech. 622, 371–411.CrossRefGoogle Scholar
Iafrati, A. 2011 Energy dissipation mechanisms in wave breaking processes: spilling and highly aerated plunging breaking events. J. Geophys. Res. 116, C7.CrossRefGoogle Scholar
Iafrati, A., Babanin, A. & Onorato, M. 2013 Modulational instability, wave breaking, and formation of large-scale dipoles in the atmosphere. Phys. Rev. Lett. 110 (18), 184504.CrossRefGoogle ScholarPubMed
Iafrati, A. & Campana, E. F. 2005 Free-surface fluctuations behind microbreakers: space–time behaviour and subsurface flow field. J. Fluid Mech. 529, 311–347.CrossRefGoogle Scholar
Jiang, L., Lin, H.-J., Schultz, W. W. & Perlin, M. 1999 Unsteady ripple generation on steep gravity capillary waves. J. Fluid Mech. 386, 281–304.CrossRefGoogle Scholar
Lamarre, E. & Melville, W. K. 1991 Air entrainment and dissipation in breaking waves. Nature 351, 469–472.CrossRefGoogle Scholar
Lamarre, E. & Melville, W. K. 1994 Void-fraction measurements and sound-speed fields in bubble plumes generated by breaking waves. J. Acoust. Soc. Am. 95 (3), 1317–1328.CrossRefGoogle Scholar
Lamb, H. 1932 Hydrodynamics. Cambridge University Press.Google Scholar
Lin, J. C. & Rockwell, D. 1995 Evolution of a quasi-steady breaking wave. J. Fluid Mech. 302, 29–44.CrossRefGoogle Scholar
Liu, X. & Duncan, J. H. 2003 The effects of surfactants on spilling breaking waves. Nature 421, 520–523.CrossRefGoogle ScholarPubMed
Liu, X. & Duncan, J. H. 2006 An experimental study of surfactant effects on spilling breakers. J. Fluid Mech. 567, 433–455.CrossRefGoogle Scholar
Loewen, M. R., O’Dor, M. A. & Skafel, M. G. 1996 Bubbles entrained by mechanically generated breaking waves. J. Geophys. Res. 101 (C9), 20759–20769.CrossRefGoogle Scholar
Longuet-Higgins, M. S. 1963 The generation of capillary waves by step gravity waves. J. Fluid Mech. 16, 138–159.CrossRefGoogle Scholar
Longuet-Higgins, M. S. 1992 Capillary rollers and bores. J. Fluid Mech. 240, 659–679.CrossRefGoogle Scholar
Longuet-Higgins, M. S. 1994 Shear instability in spilling breakers. Proc. R. Soc. A 446 (1927), 399–409.Google Scholar
Longuet-Higgins, M. S. & Cleaver, R. P. 1994 Crest instabilities of gravity waves. Part 1. The almost-highest wave. J. Fluid Mech. 258, 115–129.CrossRefGoogle Scholar
Longuet-Higgins, M. S. & Tanaka, M. 1997 On the crest instabilities of steep surface waves. J. Fluid Mech. 336, 51–68.CrossRefGoogle Scholar
McLean, J. 1982 Instabilities of finite amplitude water waves. J. Fluid Mech. 114, 315–330.CrossRefGoogle Scholar
Melville, W. K. 1982 The instability and breaking of deep-water waves. J. Fluid Mech. 115, 165–185.CrossRefGoogle Scholar
Melville, W. K. 1996 The role of surface wave breaking in air–sea interaction. Annu. Rev. Fluid Mech. 28, 279–321.CrossRefGoogle Scholar
Melville, W. K. & Fedorov, A. V. 2015 The equilibrium dynamics and statistics of gravity–capillary waves. J. Fluid Mech. 767, 449–466.CrossRefGoogle Scholar
Melville, W. K. & Matusov, P. 2002 Distribution of breaking waves at the ocean surface. Nature 417, 58–63.CrossRefGoogle ScholarPubMed
Melville, W. K. & Rapp, D. J. 1985 Momentum flux in breaking waves. Nature 317, 514–516.CrossRefGoogle Scholar
Melville, W. K., Veron, F. & White, C. J. 2002 The velocity field under breaking waves: coherent structure and turbulence. J. Fluid Mech. 454, 202–233.CrossRefGoogle Scholar
Mui, R. C. & Dommermuth, D. G. 1995 Vortex generation by deep-water breaking waves. Trans. ASME J. Fluids Engng 117, 355–361.CrossRefGoogle Scholar
Peregrine, D. H., Cokelet, E. D. & McIver, P. 1993 Wave breaking in deep water. Annu. Rev. Fluid Mech. 25, 373–397.Google Scholar
Perlin, M., Choi, W. & Tian, Z. 2013 Breaking waves in deep and intermediate waters. Annu. Rev. Fluid Mech. 45, 115–145.CrossRefGoogle Scholar
Perlin, M., Lin, H.-J. & Ting, C.-L. 1993 On parasitic capillary waves generated by steep gravity waves: an experimental investigation with spatial and temporal measurements. J. Fluid Mech. 255, 597–620.CrossRefGoogle Scholar
Phillips, O. M. 1985 Spectral and statistical properties of the equilibrium range in wind-generated gravity waves. J. Fluid Mech. 156 (1), 505–531.CrossRefGoogle Scholar
Pizzo, N. E. & Melville, W. K. 2013 Vortex generation by deep-water breaking waves. J. Fluid Mech. 734, 198–218.CrossRefGoogle Scholar
Popinet, S. 2003 Gerris: a tree-based adaptative solver for the incompressible Euler equations in complex geometries. J. Comput. Phys. 190, 572–600.CrossRefGoogle Scholar
Popinet, S. 2009 An accurate adaptative solver for surface-tension-driven interfacial flows. J. Comput. Phys. 228, 5838–5866.CrossRefGoogle Scholar
Qiao, H. & Duncan, J. H. 2001 Gentle spilling breakers: crest flow field evolution. J. Fluid Mech. 439, 57–85.CrossRefGoogle Scholar
Rapp, R. J. & Melville, W. K. 1990 Laboratory measurements of deep water breaking waves. Phil. Trans. R. Soc. Lond. A 331, 735–800.Google Scholar
Rojas, G. & Loewen, M. R. 2010 Void fraction measurements beneath plunging and spilling breaking waves. J. Geophys. Res. 118, 08001.Google Scholar
Romero, L., Melville, W. K. & Kleiss, J. M. 2012 Spectral energy dissipation due to surface wave breaking. J. Phys. Oceanogr. 42, 1421–1441.CrossRefGoogle Scholar
Schwartz, L. 1966 Mathematics for the Physical Sciences. Hermann.Google Scholar
Song, J.-B. & Banner, M. L. 2002 On determining the onset and strength of breaking for deep water waves. Part I: unforced irrotational wave groups. J. Phys. Oceanogr. 32 (9), 2541–2558.CrossRefGoogle Scholar
Song, C. & Sirviente, A. 2004 A numerical study of breaking waves. Phys. Fluids 16, 26–49.CrossRefGoogle Scholar
Su, M.-Y. 1982 Three dimensional deep water waves. Part 1. Experimental measurement of skew and symetric wave patterns. J. Fluid Mech. 124, 73–108.CrossRefGoogle Scholar
Sutherland, P. & Melville, W. K. 2013 Field measurements and scaling of ocean surface wave-breaking statistics. Geophys. Res. Lett. 40, 3074–3079.CrossRefGoogle Scholar
Tsai, W.-t. & Hung, L. 2007 Three-dimensional modeling of small-scale processes in the upper boundary layer bounded by a dynamic ocean surface. J. Geophys. Res. 112, C02019.CrossRefGoogle Scholar
Tsai, W.-t. & Hung, L. 2010 Enhanced energy dissipation by parasitic capillaries on short gravity–capillary waves. J. Phys. Oceanogr. 40, 2435–2450.CrossRefGoogle Scholar
Tulin, M. P. & Waseda, T. 1999 Laboratory observations of wave group evolution, including breaking effects. J. Fluid Mech. 378, 197–232.CrossRefGoogle Scholar
Veron, F., Melville, W. K. & Lenain, L. 2008 Wave-coherent air–sea heat flux. J. Phys. Oceanogr. 38, 788–802.CrossRefGoogle Scholar
Yang, Y. & Tryggvason, G. 1998 Dissipation of energy by finite amplitude surface waves. Comput. Fluids 27 (7), 829–845.CrossRefGoogle Scholar
Zhang, X. 1995 Capillary–gravity and capillary waves generated in a wind wave tank: observations and theories. J. Fluid Mech. 289, 51–82.CrossRefGoogle Scholar
Zhang, S., Duncan, J. H. & Chahine, G. L. 1993 The final stage of the collapse of a cavitation bubble near a rigid wall. J. Fluid Mech. 257, 147–181.CrossRefGoogle Scholar