Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Wu, Jiarong
Popinet, Stéphane
and
Deike, Luc
2022.
Revisiting wind wave growth with fully coupled direct numerical simulations.
Journal of Fluid Mechanics,
Vol. 951,
Issue. ,
Deike, Luc
2022.
Mass Transfer at the Ocean–Atmosphere Interface: The Role of Wave Breaking, Droplets, and Bubbles.
Annual Review of Fluid Mechanics,
Vol. 54,
Issue. 1,
p.
191.
Patankar, Sagar
Basak, Saswata
and
Dasgupta, Ratul
2022.
Dynamic stabilisation of Rayleigh–Plateau modes on a liquid cylinder.
Journal of Fluid Mechanics,
Vol. 946,
Issue. ,
van Hooft, J. Antoon
and
Popinet, Stéphane
2022.
A fourth-order accurate adaptive solver for incompressible flow problems.
Journal of Computational Physics,
Vol. 462,
Issue. ,
p.
111251.
Farsoiya, Palas Kumar
Popinet, Stéphane
and
Deike, Luc
2022.
Direct numerical simulations of dilute gas transfer by breaking waves.
Physical Review Fluids,
Vol. 7,
Issue. 11,
Mostert, W.
Popinet, S.
and
Deike, L.
2022.
High-resolution direct simulation of deep water breaking waves: transition to turbulence, bubbles and droplets production.
Journal of Fluid Mechanics,
Vol. 942,
Issue. ,
Farsoiya, Palas Kumar
Magdelaine, Quentin
Antkowiak, Arnaud
Popinet, Stéphane
and
Deike, Luc
2023.
Direct numerical simulations of bubble-mediated gas transfer and dissolution in quiescent and turbulent flows.
Journal of Fluid Mechanics,
Vol. 954,
Issue. ,
Farsoiya, Palas Kumar
Liu, Zehua
Daiss, Andreas
Fox, Rodney O.
and
Deike, Luc
2023.
Role of viscosity in turbulent drop break-up.
Journal of Fluid Mechanics,
Vol. 972,
Issue. ,
Huang, Guangyuan
Lv, Xin
Chen, Wuguang
Song, Yuchen
Yin, Junlian
and
Wang, Dezhong
2024.
Measurement of interfacial mass transfer of single bubbles rising in homogeneous turbulence.
Chemical Engineering Science,
Vol. 288,
Issue. ,
p.
119757.
Farsoiya, Palas Kumar
Popinet, Stéphane
Stone, Howard A.
and
Deike, Luc
2024.
Coupled volume of fluid and phase field method for direct numerical simulation of insoluble surfactant-laden interfacial flows and application to rising bubbles.
Physical Review Fluids,
Vol. 9,
Issue. 9,
Mikushin, Pavel
Nizovtseva, Irina
Starodumov, Ilya
Makhaeva, Ksenia
Vikharev, Sergey
Lezhnin, Sergey
and
Chernushkin, Dmitrii
2024.
Mass transfer estimation in gas–liquid systems through integration of hydrodynamic model and computer vision algorithms.
The European Physical Journal Special Topics,
Vol. 233,
Issue. 23-24,
p.
3535.
Tourbier, Alexis
Gamet, Lionel
Béard, Philippe
Michel, Typhène
Aubin, Joelle
and
Jasak, Hrvoje
2024.
A consistent methodology to transport a passive scalar with the geometric Volume-of-Fluid method isoAdvector.
Journal of Computational Physics,
Vol. 513,
Issue. ,
p.
113198.
Manthey, J.
Guesmi, M.
Schab, R.
Unz, S.
and
Beckmann, M.
2024.
Bubble evolution due to super-saturation in the cooling circuit of the PEM-electrolysis.
International Journal of Hydrogen Energy,
Vol. 78,
Issue. ,
p.
433.
Liu, Zehua
Farsoiya, Palas Kumar
Perrard, Stéphane
and
Deike, Luc
2024.
Direct numerical simulation of bubble rising in turbulence.
Journal of Fluid Mechanics,
Vol. 999,
Issue. ,
Gennari, Gabriele
Jefferson-Loveday, Richard
Pickering, Stephen J.
and
George, Michael W.
2024.
Bubble dissolution in Taylor–Couette flow.
Journal of Fluid Mechanics,
Vol. 999,
Issue. ,
Mangani, Francesca
Roccon, Alessio
Zonta, Francesco
and
Soldati, Alfredo
2024.
Heat transfer in drop-laden turbulence.
Journal of Fluid Mechanics,
Vol. 978,
Issue. ,
Wei, Xinyu
Song, Shiyu
Yu, Tianlu
Zhang, Yan
Li, Jiqun
Ma, Yifan
Yin, Yujie
and
Yang, Jianlin
2025.
In situ microbubble-mediated strategy for highly efficient anti-crude oil-fouling membrane with parallel nanosheet structure.
Separation and Purification Technology,
Vol. 357,
Issue. ,
p.
130220.
Cioncolini, Andrea
and
Magnini, Mirco
2025.
Solitary bubbles rising in quiescent liquids: A critical assessment of experimental data and high-fidelity numerical simulations, and performance evaluation of selected prediction methods.
Physics of Fluids,
Vol. 37,
Issue. 2,
Yang, Wenchang
Levin, Emma
Menemenlis, Sofia
Scapin, Nicoló
Igbinoba, Michael
Chung, Maya
Rios, Gabriel
Hsieh, Tsung-Lin
Deike, Luc
Mitevski, Ivan
and
Vecchi, Gabriel A.
2025.
Tropical Cyclones and Associated Impacts.
p.
1.
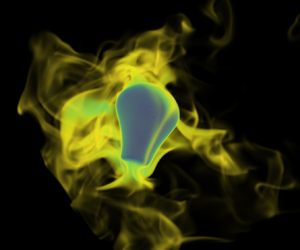
 $\widetilde {{Pe}}$. We propose a suitable time scale
$\widetilde {{Pe}}$. We propose a suitable time scale  $\theta$ for Higbie (Trans. AIChE, vol. 31, 1935, pp. 365–389) penetration theory,
$\theta$ for Higbie (Trans. AIChE, vol. 31, 1935, pp. 365–389) penetration theory,  $\theta = d_0/\tilde {u}$, based on
$\theta = d_0/\tilde {u}$, based on  $d_0$ the bubble diameter and
$d_0$ the bubble diameter and  $\tilde {u}$ a characteristic turbulent velocity, here
$\tilde {u}$ a characteristic turbulent velocity, here  $\tilde {u}=\sqrt {3}\,u_{{rms}}$, where
$\tilde {u}=\sqrt {3}\,u_{{rms}}$, where  $u_{{rms}}$ is the large-scale turbulence fluctuations. This leads to a non-dimensional transfer rate
$u_{{rms}}$ is the large-scale turbulence fluctuations. This leads to a non-dimensional transfer rate  ${Sh} = 2(3)^{1/4}\sqrt {\widetilde {{Pe}}/{\rm \pi} }$ from the bubble in the isotropic turbulent flow. The theoretical prediction is verified by direct numerical simulations of mass transfer of dilute gas from a bubble in homogeneous and isotropic turbulence, and very good agreement is observed as long as the thin boundary layer is properly resolved.
${Sh} = 2(3)^{1/4}\sqrt {\widetilde {{Pe}}/{\rm \pi} }$ from the bubble in the isotropic turbulent flow. The theoretical prediction is verified by direct numerical simulations of mass transfer of dilute gas from a bubble in homogeneous and isotropic turbulence, and very good agreement is observed as long as the thin boundary layer is properly resolved.