Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-28T03:52:07.492Z Has data issue: false hasContentIssue false
  • English
  • Français

Branching behaviour of the Rayleigh–Taylor instability in linear viscoelastic fluids

Published online by Cambridge University Press:  17 March 2021

B. Dinesh Open the ORCID record for B. Dinesh [Opens in a new window]  and
R. Narayanan
B. Dinesh*
Affiliation:
Department of Chemical Engineering, University of Florida, Gainesville, FL32611, USA
R. Narayanan
Affiliation:
Department of Chemical Engineering, University of Florida, Gainesville, FL32611, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

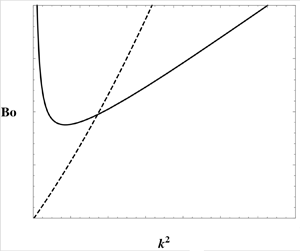
The Rayleigh–Taylor instability of a linear viscoelastic fluid overlying a passive gas is considered, where, under neutral conditions, the key dimensionless groups are the Bond number and the Weissenberg number. The branching behaviour upon instability to sinusoidal disturbances is determined by weak nonlinear analysis with the Bond number advanced from its critical value at neutral stability. It is shown that the solutions emanating from the critical state either branch out supercritically to steady waves at predictable wavelengths or break up subcritically with a wavelength having a single node. The nonlinear analysis leads to the counterintuitive observation that Rayleigh–Taylor instability of a viscoelastic fluid in a laterally unbounded layer must always result in saturated steady waves. The analysis also shows that the subcritical breakup in a viscoelastic fluid can only occur if the layer is laterally bounded below a critical horizontal width. If the special case of an infinitely deep viscoelastic layer is considered, a simple expression is obtained from which the transition between steady saturated waves and subcritical behaviour can be determined in terms of the leading dimensionless groups. This expression reveals that the supercritical saturation of the free surface is due to the influence of the normal elastic stresses, while the subcritical rupture of the free surface is attributed to the influence of capillary effects. In short, depending upon the magnitude of the scaled shear modulus, there exists a wavenumber at which a transition from saturated waves to subcritical breakup occurs.

JFM classification

Non-Newtonian Flows: Viscoelasticity Nonlinear Dynamical Systems: Bifurcation
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 915 , 25 May 2021 , A63
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Andrews, M.J. & Dalziel, S.B. 2010 Small Atwood number Rayleigh–Taylor experiments. Phil. Trans. R. Soc. Lond. A 368 (1916), 1663–1679.Google ScholarPubMed
Bellman, R. & Pennington, R.H. 1954 Effects of surface tension and viscosity on Taylor instability. Q. Appl. Maths 12 (2), 151–162.CrossRefGoogle Scholar
Brown, H.R. 1989 Rayleigh–Taylor instability in a finite thickness layer of a viscous fluid. Phys. Fluids A 1 (5), 895–896.CrossRefGoogle Scholar
Chakrabarti, A., Mora, S., Richard, F., Phou, T., Fromental, J.-M., Pomeau, Y. & Audoly, B. 2018 Selection of hexagonal buckling patterns by the elastic Rayleigh–Taylor instability. J. Mech. Phys. Solids 121, 234–257.CrossRefGoogle Scholar
Chandrasekhar, S. 1961 Hydrodynamic and Hydromagnetic Stability. Clarendon.Google Scholar
Dalziel, S.B. 1993 Rayleigh–Taylor instability: experiments with image analysis. Dyn. Atmos. Oceans 20 (1–2), 127–153.CrossRefGoogle Scholar
Dietze, G.F. & Ruyer-Quil, C. 2015 Films in narrow tubes. J. Fluid Mech. 762, 68–109.CrossRefGoogle Scholar
Dinesh, B. & Pushpavanam, S. 2017 Linear stability of layered two-phase flows through parallel soft-gel-coated walls. Phys. Rev. E 96 (1), 013119.CrossRefGoogle ScholarPubMed
Elgowainy, A. & Ashgriz, N. 1997 The Rayleigh–Taylor instability of viscous fluid layers. Phys. Fluids 9 (6), 1635–1649.CrossRefGoogle Scholar
Forbes, L.K. 2009 The Rayleigh–Taylor instability for inviscid and viscous fluids. J. Engng Maths 65 (3), 273–290.CrossRefGoogle Scholar
Guo, W., Labrosse, G. & Narayanan, R. 2013 The Application of the Chebyshev–Spectral Method in Transport Phenomena. Springer Science & Business Media.Google Scholar
Howell, P., Kozyreff, G. & Ockendon, J. 2009 Applied Solid Mechanics. Cambridge University Press.Google Scholar
Inogamov, N.A. 1999 The role of Rayleigh–Taylor and Richtmyer–Meshkov instabilities in astrophysics: an introduction. Astrophys. Space Phys. Rev. 10, 1–335.Google Scholar
Johns, L.E. & Narayanan, R. 2002 Interfacial Instability. Springer Science & Business Media.Google Scholar
Jørgensen, L., Le Merrer, M., Delanoë-Ayari, H. & Barentin, C. 2015 Yield stress and elasticity influence on surface tension measurements. Soft Matt. 11 (25), 5111–5121.CrossRefGoogle ScholarPubMed
Joseph, D.D. 1976 Stability of Fluid Motions II, vol. 28. Springer Tracts in Natural Philosophy.Google Scholar
Kull, H. 1991 Theory of the Rayleigh–Taylor instability. Phys. Rep. 206 (5), 197–325.CrossRefGoogle Scholar
Landau, L.D. & Lifshitz, E.M. 1989 Theory of Elasticity. Pergamon.Google Scholar
Lin, P., Lin, X., Johns, L.E. & Narayanan, R. 2019 Stability of a static liquid bridge knowing only its shape. Phys. Rev. Fluids 4 (12), 123904.CrossRefGoogle Scholar
Marthelot, J., Strong, E.F., Reis, P.M. & Brun, P.T. 2018 Designing soft materials with interfacial instabilities in liquid films. Nat. Commun. 9 (1), 1–7.CrossRefGoogle ScholarPubMed
Mikaelian, K.O. 1990 Rayleigh–Taylor and Richtmyer–Meshkov instabilities in multilayer fluids with surface tension. Phys. Rev. A 42 (12), 7211.CrossRefGoogle ScholarPubMed
Mora, S., Phou, T., Fromental, J.-M. & Pomeau, Y. 2014 Gravity driven instability in elastic solid layers. Phys. Rev. Lett. 113, 178301.CrossRefGoogle ScholarPubMed
Müller, H.W. & Zimmermann, W. 1999 Faraday instability in a linear viscoelastic fluid. Europhys. Lett. 45 (2), 169.CrossRefGoogle Scholar
Newhouse, L.A. & Pozrikidis, C. 1990 The Rayleigh–Taylor instability of a viscous liquid layer resting on a plane wall. J. Fluid Mech. 217, 615–638.CrossRefGoogle Scholar
Olson, D.H. & Jacobs, J.W. 2009 Experimental study of Rayleigh–Taylor instability with a complex initial perturbation. Phys. Fluids 21 (3), 034103.CrossRefGoogle Scholar
Patne, R., Giribabu, D. & Shankar, V. 2017 Consistent formulations for stability of fluid flow through deformable channels and tubes. J. Fluid Mech. 827, 31–66.CrossRefGoogle Scholar
Pullin, D.I. 1982 Numerical studies of surface-tension effects in nonlinear Kelvin–Helmholtz and Rayleigh–Taylor instability. J. Fluid Mech. 119, 507–532.CrossRefGoogle Scholar
Ramaprabhu, P. & Andrews, M.J. 2004 Experimental investigation of Rayleigh–Taylor mixing at small Atwood numbers. J. Fluid Mech. 502, 233–271.CrossRefGoogle Scholar
Ratafia, M. 1973 Experimental investigation of Rayleigh–Taylor instability. Phys. Fluids 16 (8), 1207–1210.CrossRefGoogle Scholar
Rayleigh, Lord 1882 Investigation of the character of the equilibrium of an incompressible heavy fluid of variable density. Proc. Lond. Math. Soc. s1-14, 170–177.CrossRefGoogle Scholar
Riccobelli, D. & Ciarletta, P. 2017 Rayleigh–Taylor instability in soft elastic layers. Phil. Trans. R. Soc. Lond. A 375 (2093), 20160421.Google ScholarPubMed
Shankar, V. & Kumaran, V. 2000 Stability of fluid flow in a flexible tube to non-axisymmetric disturbances. J. Fluid Mech. 407, 291–314.CrossRefGoogle Scholar
Sharp, D.H. 1984 An overview of Rayleigh–Taylor instability. Physica D, 12 (1–3), 3–18.CrossRefGoogle Scholar
Tryggvason, G. 1988 Numerical simulations of the Rayleigh–Taylor instability. J. Comput. Phys. 75 (2), 253–282.CrossRefGoogle Scholar
Yiantsios, S.G. & Higgins, B.G. 1989 Rayleigh–Taylor instability in thin viscous films. Phys. Fluids A 1 (9), 1484–1501.CrossRefGoogle Scholar
Yue, Z., Yang, L, Yuhang, H. & Shengqiang, C. 2019 Rayleigh–Taylor instability in a confined elastic soft cylinder. J. Mech. Phys. Solids 131, 221–229.Google Scholar
Supplementary material: File

Dinesh and Narayanan supplementary material

Dinesh and Narayanan supplementary material

Download Dinesh and Narayanan supplementary material(File)
File 265 KB