Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-12-01T00:46:55.107Z Has data issue: false hasContentIssue false
  • English
  • Français

Boundary layer structure in confined turbulent thermal convection

Published online by Cambridge University Press:  29 August 2012

R. Verzicco
R. Verzicco*
Affiliation:
Dipartimento di Ingegneria Industriale, Università di Roma ‘Tor Vergata’, via del Politecnico 1, 00133, Roma, Italy Physics of Fluids, University of Twente, PO Box 217, 7500 AE Enschede, The Netherlands
*
†Email address for correspondence: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The structure of viscous and thermal boundary layers at the heated and cooled plates in turbulent thermally driven flows are of fundamental importance for heat transfer and its dependence on the thermal forcing (the Rayleigh number 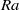in non-dimensional form). The paper by Shi, Emran & Schumacher (J. Fluid Mech., this issue, vol. 706, 2012, pp. 5–33) stresses the deviations of the boundary layer vertical profiles from the Prandtl–Blasius–Pohlhausen theory. Recent papers showing very similar results, in contrast, focus more on the similarities.

JFM classification

Boundary Layers: Boundary layer structure Convection: Buoyant boundary layers
Type
Focus on Fluids
Information
Journal of Fluid Mechanics , Volume 706 , 10 September 2012 , pp. 1 - 4
Copyright
Copyright © Cambridge University Press 2012

References

1. Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large-scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81 (2), 503–537.CrossRefGoogle Scholar
2. Blasius, H. 1908 Grenzschichten in Flüssigkeiten mit keiner Reibung. Z. Math. Phys. 56, 1–37.Google Scholar
3. Gauthier, F. & Roche, P.-E. 2008 Evidence of a boundary layer instability at very high Rayleigh number. Europhys. Lett. 83, 24005.Google Scholar
4. Grossmann, S. & Lohse, D. 2000 Scaling in thermal convection: a unifying theory. J. Fluid Mech. 407, 27–56.CrossRefGoogle Scholar
5. Kraichnan, R. H. 1962 Turbulent thermal convection at arbitrary Prandtl number. Phys. Fluids 5, 1374–1389.Google Scholar
6. Kunnen, R. P. J., Stevens, R. J. A. M., Overkamp, J., Sun, C., van Heijst, G. J. F. & Clercx, H. J. H. 2011 The role of Stewartson and Ekman layers in turbulent rotating Rayleigh–Bénard convection. J. Fluid Mech. 688, 422–442.CrossRefGoogle Scholar
7. Malkus, M. V. R. 1954 Heat transport and spectrum of thermal turbulence. Proc. R. Soc. Lond. A 225, 196–212.Google Scholar
8. du Puits, R., Resagk, C., Tilgner, A., Busse, F. H. & Thess, A. 2007 Structure of thermal boundary layers in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 572, 231–254.Google Scholar
9. Schlichting, H. 1979 Boundary Layer Theory. McGraw-Hill.Google Scholar
10. Shi, N., Emran, M. S. & Schumacher, J. 2012 Boundary layer structure in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 706, 5–33.CrossRefGoogle Scholar
11. Shraiman, B. I. & Siggia, E. D. 1990 Heat transport in high Rayleigh number convection. Phys. Rev. A 42, 3650–3653.CrossRefGoogle ScholarPubMed
12. Stevens, R. J. A. M., Zhou, Q., Grossmann, S., Verzicco, R., Xia, K.-Q. & Lohse, D. 2012 Thermal boundary layer profiles in turbulent Rayleigh–Bénard convection in a cylindrical sample. Phys. Rev. E 85, 027301.CrossRefGoogle Scholar
13. Sun, C., Cheung, Y.-H. & Xia, K.-Q. 2008 Experimental studies of the viscous boundary layer properties in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 605, 79–113.CrossRefGoogle Scholar
14. Zhou, Q. & Xia, K.-Q. 2010 Measured instantaneous viscous boundary layer in turbulent Rayleigh–Bénard convection. Rayleigh–Bénard convection in a cylindrical sample. Phys. Rev. Lett. 104, 104301.Google Scholar