Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-30T20:49:41.908Z Has data issue: false hasContentIssue false
  • English
  • Français

Bistabilities in two parallel Kármán wakes

Published online by Cambridge University Press:  19 October 2021

Chengjiao Ren Open the ORCID record for Chengjiao Ren [Opens in a new window] ,
Liang Cheng Open the ORCID record for Liang Cheng [Opens in a new window] ,
Chengwang Xiong Open the ORCID record for Chengwang Xiong [Opens in a new window] ,
Feifei Tong Open the ORCID record for Feifei Tong [Opens in a new window]  and
Tingguo Chen
Chengjiao Ren
Affiliation:
State Key Laboratory of Coastal and Offshore Engineering, Dalian University of Technology, Dalian 116024, PR China Faculty of Infrastructure Engineering, Dalian University of Technology, Dalian 116024, PR China School of Engineering, The University of Western Australia, 35 Stirling Hwy, Crawley, WA 6009, Australia
Liang Cheng*
Affiliation:
State Key Laboratory of Coastal and Offshore Engineering, Dalian University of Technology, Dalian 116024, PR China Faculty of Infrastructure Engineering, Dalian University of Technology, Dalian 116024, PR China School of Engineering, The University of Western Australia, 35 Stirling Hwy, Crawley, WA 6009, Australia
Chengwang Xiong
Affiliation:
College of Shipbuilding Engineering, Harbin Engineering University, Harbin 150001, PR China School of Engineering, Ocean University of China, Qingdao 266100, PR China
Feifei Tong
Affiliation:
School of Engineering, The University of Western Australia, 35 Stirling Hwy, Crawley, WA 6009, Australia
Tingguo Chen
Affiliation:
Faculty of Infrastructure Engineering, Dalian University of Technology, Dalian 116024, PR China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

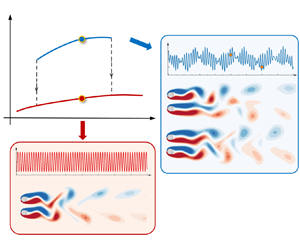
Bistabilities of two equilibrium states discovered in the coupled side-by-side Kármán wakes are investigated through Floquet analysis and direct numerical simulation (DNS) with different initial conditions over a range of gap-to-diameter ratio ($g^*= 0.2\text {--}3.5$) and Reynolds number ($Re = 47\text {--}100$). Two bistabilities are found in the transitional $g^*-Re$ regions from in-phase (IP) to anti-phase (AP) vortex shedding states. By initialising the flow in DNS with zero initial conditions, the flow in the first bistable region (i.e. bistable IP/FF$_C$ at $g^*= 1.4 \text {--} 2.0$, where FF$_C$ denotes the conditional flip-flop flow) attains flip-flop (FF) flow, it settles into the IP state by initialising the flow with an IP flow. The second bistability is observed between cylinder-scale IP and AP states at large $g^*$ ($=$ 2.0–3.5). The transition from the FF$_C$ to IP is dependent on initial conditions and irreversible over the parameter space, meaning that the flow cannot revert back to the FF$_C$ state once it jumps to the IP state irrespective of the direction of $Re$ variations. Its counterpart for the bistable IP/AP state is reversible. We also found that the FF$_C$ flow in the first bistable region is primarily bifurcated from synchronised AP with cluster-scale features, possibly because the cluster-scale AP flow is inherently unstable to FF flow instabilities. It is demonstrated that the irreversible bistability exists in other interacting wakes around multiple cylinders. A good understanding of flow bistabilities is pivotal to flow control applications and the interpretation of desynchronised flow features observed at high $Re$ values.

JFM classification

Nonlinear Dynamical Systems: Bifurcation Wakes/Jets: Vortex streets
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 929 , 25 December 2021 , A5
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Aswathy, M.S. & Sarkar, S. 2021 Frequency characteristics and phase dynamics of a stochastic vortex induced vibration system. J. Sound Vib. 509, 116230.CrossRefGoogle Scholar
Barkley, D. & Henderson, R.D. 1996 Three-dimensional floquet stability analysis of the wake of a circular cylinder. J. Fluid Mech. 322, 215–241.CrossRefGoogle Scholar
Blackburn, H.M. & Sherwin, S.J. 2004 Formulation of a Galerkin spectral element–Fourier method for three-dimensional incompressible flows in cylindrical geometries. J. Comput. Phys. 197 (2), 759–778.CrossRefGoogle Scholar
Cantwell, C.D., et al. 2015 Nektar++: an open-source spectral/$hp$ element framework. Comput. Phys. Commun. 192, 205–219.CrossRefGoogle Scholar
Carini, M., Auteri, F. & Giannetti, F. 2015 Secondary instabilities of the in-phase synchronized wakes past two circular cylinders in side-by-side arrangement. J. Fluids Struct. 53, 70–83.CrossRefGoogle Scholar
Carini, M., Giannetti, F. & Auteri, F. 2014 a First instability and structural sensitivity of the flow past two side-by-side cylinders. J. Fluid Mech. 749, 627–648.CrossRefGoogle Scholar
Carini, M., Giannetti, F. & Auteri, F. 2014 b On the origin of the flip–flop instability of two side-by-side cylinder wakes. J. Fluid Mech. 742, 552–576.CrossRefGoogle Scholar
Citro, V., Luchini, P., Giannetti, F. & Auteri, F. 2017 Efficient stabilization and acceleration of numerical simulation of fluid flows by residual recombination. J. Comput. Phys. 344, 234–246.CrossRefGoogle Scholar
Elston, J.R., Blackburn, H.M. & Sheridan, J. 2006 The primary and secondary instabilities of flow generated by an oscillating circular cylinder. J. Fluid Mech. 550, 359–389.CrossRefGoogle Scholar
Elston, J.R., Sheridan, J. & Blackburn, H.M. 2004 Two-dimensional Floquet stability analysis of the flow produced by an oscillating circular cylinder in quiescent fluid. Eur. J. Mech. (B/Fluids) 23 (1), 99–106.CrossRefGoogle Scholar
Feng, C.C. 1968 The measurement of vortex induced effects in flow past stationary and oscillating circular and D-section cylinders. Master's thesis, University of British Columbia, Vancouver, BC, Canada.Google Scholar
Ganapathisubramanian, N. & Showalter, K. 1984 Bistability, mushrooms, and isolas. J. Chem. Phys. 80 (9), 4177–4184.CrossRefGoogle Scholar
Guermond, J. & Shen, J. 2003 Velocity-correction projection methods for incompressible flows. SIAM J. Numer. Anal. 41 (1), 112–134.CrossRefGoogle Scholar
Guidi, G.M. & Goldbeter, A. 1997 Bistability without hysteresis in chemical reaction systems: a theoretical analysis of irreversible transitions between multiple steady states. J. Phys. Chem. A 101 (49), 9367–9376.CrossRefGoogle Scholar
Henderson, R.D. & Barkley, D. 1996 Secondary instability in the wake of a circular cylinder. Phys. Fluids 8 (6), 1683–1685.CrossRefGoogle Scholar
Kang, S. 2003 Characteristics of flow over two circular cylinders in a side-by-side arrangement at low Reynolds numbers. Phys. Fluids 15 (9), 2486–2498.CrossRefGoogle Scholar
Karniadakis, G.E., Israeli, M. & Orszag, S.A. 1991 High-order splitting methods for the incompressible Navier–Stokes equations. J. Comput. Phys. 97 (2), 414–443.CrossRefGoogle Scholar
Kashinath, K., Li, L.K.B. & Juniper, M.P. 2018 Forced synchronization of periodic and aperiodic thermoacoustic oscillations: lock-in, bifurcations and open-loop control. J. Fluid Mech. 838, 690–714.CrossRefGoogle Scholar
Khalak, A. & Williamson, C.H.K. 1999 Motions, forces and mode transitions in vortex-induced vibrations at low mass-damping. J. Fluids Struct. 13 (7), 813–851.CrossRefGoogle Scholar
Konstantinidis, E., Zhao, J., Leontini, J., Lo Jacono, D. & Sheridan, J. 2020 Phase dynamics of effective drag and lift components in vortex-induced vibration at low mass–damping. J. Fluids Struct. 96, 103028.CrossRefGoogle Scholar
Li, L.K.B. & Juniper, M.P. 2013 Phase trapping and slipping in a forced hydrodynamically self-excited jet. J. Fluid Mech. 735, R5.CrossRefGoogle Scholar
Ma, S., Kang, C., Lim, T., Wu, C. & Tutty, O. 2017 Wake of two side-by-side square cylinders at low Reynolds numbers. Phys. Fluids 29 (3), 033604.CrossRefGoogle Scholar
Mizushima, J. & Hatsuda, G. 2014 Nonlinear interactions between the two wakes behind a pair of square cylinders. J. Fluid Mech. 759, 295–320.CrossRefGoogle Scholar
Mizushima, J. & Ino, Y. 2008 Stability of flows past a pair of circular cylinders in a side-by-side arrangement. J. Fluid Mech. 595, 491–507.CrossRefGoogle Scholar
Pikovsky, A., Rosenblum, M. & Kurths, J. 2003 Synchronization, Part of Cambridge Nonlinear Science Series, vol. 2. Cambridge University Press.CrossRefGoogle Scholar
Ren, C., Cheng, L., Tong, F., Xiong, C. & Chen, T. 2019 Oscillatory flow regimes around four cylinders in a diamond arrangement. J. Fluid Mech. 877, 955–1006.CrossRefGoogle Scholar
Ren, C., Lu, L., Cheng, L. & Chen, T. 2021 Hydrodynamic damping of an oscillating cylinder at small Keulegan–Carpenter numbers. J. Fluid Mech. 913, A36.CrossRefGoogle Scholar
Vos, P.E.J., Eskilsson, C., Bolis, A., Chun, S., Kirby, R.M. & Sherwin, S.J. 2011 A generic framework for time-stepping partial differential equations (PDEs): general linear methods, object-oriented implementation and application to fluid problems. Intl J. Comput. Fluid Dyn. 25 (3), 107–125.CrossRefGoogle Scholar
Xiong, C., Cheng, L., Tong, F. & An, H. 2018 Oscillatory flow regimes for a circular cylinder near a plane boundary. J. Fluid Mech. 844, 127–161.CrossRefGoogle Scholar