Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Bourguet, Rémi
2020.
Vortex-induced vibrations of a flexible cylinder at subcritical Reynolds number.
Journal of Fluid Mechanics,
Vol. 902,
Issue. ,
Boersma, Pieter R.
Zhao, Jay
Rothstein, Jonathan P.
and
Modarres-Sadeghi, Yahya
2021.
Experimental evidence of vortex-induced vibrations at subcritical Reynolds numbers.
Journal of Fluid Mechanics,
Vol. 922,
Issue. ,
Bourquard, Claire
Faure-Beaulieu, Abel
and
Noiray, Nicolas
2021.
Whistling of deep cavities subject to turbulent grazing flow: intermittently unstable aeroacoustic feedback.
Journal of Fluid Mechanics,
Vol. 909,
Issue. ,
Liu, Bin
and
Zhu, Hongjun
2021.
Secondary lock-in of vortex-induced vibration and energy transfer characteristics of a vibrating cylinder subject to cross buoyancy.
Physics of Fluids,
Vol. 33,
Issue. 7,
Lyu, Zhen
Kou, Jiaqing
and
Zhang, Weiwei
2022.
An experimental modal testing method for subcritical flow around a cylinder.
Physics of Fluids,
Vol. 34,
Issue. 8,
Cheng, Zhi
Lien, Fue-Sang
Dowell, Earl H.
Wang, Ryne
and
Zhang, Ji Hao
2022.
Data-driven stability analysis via the superposition of reduced-order models for the flutter of circular cylinder submerged in three-dimensional spanwise shear inflow at subcritical Reynolds number.
Physics of Fluids,
Vol. 34,
Issue. 12,
Lorite-Díez, Manuel
Ern, Patricia
Cazin, Sébastien
Mougel, Jérôme
and
Bourguet, Rémi
2022.
An experimental study of flow–structure interaction regimes of a freely falling flexible cylinder.
Journal of Fluid Mechanics,
Vol. 946,
Issue. ,
Dolci, Daiane Iglesia
and
Carmo, Bruno Souza
2022.
Sensitivity of the least stable modes to passive control for a flow around an elastically mounted circular cylinder.
Physical Review Fluids,
Vol. 7,
Issue. 8,
Patel, Umang N.
Rothstein, Jonathan P.
and
Modarres-Sadeghi, Yahya
2022.
Vortex-induced vibrations of a cylinder in inelastic shear-thinning and shear-thickening fluids.
Journal of Fluid Mechanics,
Vol. 934,
Issue. ,
Bourguet, Rémi
2023.
Forced rotation enhances cylinder flow-induced vibrations at subcritical Reynolds number.
Journal of Fluid Mechanics,
Vol. 955,
Issue. ,
Bourguet, Rémi
2023.
Path curvature enhances the flow-induced vibrations of a cylinder without structural restoring force.
Journal of Fluid Mechanics,
Vol. 977,
Issue. ,
Dorogi, Dániel
Baranyi, László
and
Konstantinidis, Efstathios
2023.
Modulation and hysteresis in vortex-induced vibration of a spring-mounted cylinder in a slowly varying oscillatory stream.
Journal of Fluids and Structures,
Vol. 122,
Issue. ,
p.
103982.
Zhang, Zhiyu
Lu, Jianfeng
and
Zhang, Xing
2024.
Global stability analysis of flow-induced-vibration problems using an immersed boundary method.
Journal of Fluids and Structures,
Vol. 130,
Issue. ,
p.
104187.
Carvalho, I.A.
and
Assi, G.R.S.
2024.
On the omnidirectionality of a system with eight spinning rods for wake control of a circular cylinder in laminar regime.
Journal of Fluids and Structures,
Vol. 124,
Issue. ,
p.
104053.
Carvalho, I.A.
and
Assi, G.R.S.
2024.
Omnidirectional control of the wake of a circular cylinder with spinning rods subject to a turbulent flow.
Journal of Fluids and Structures,
Vol. 130,
Issue. ,
p.
104191.
Zhao, Yawei
Chen, Weilin
Ji, Chunning
and
Zhang, Zhimeng
2024.
Flow-induced vibrations of an equilateral triangular prism at subcritical Reynolds number.
Physics of Fluids,
Vol. 36,
Issue. 11,
Bourguet, Rémi
2024.
Flow-induced vibrations with and without structural restoring force: convergence under the effect of path curvature.
Journal of Fluid Mechanics,
Vol. 984,
Issue. ,
Heydari, Shayan
and
Jaiman, Rajeev K.
2025.
Flow-induced vibration of a flexible cantilever in tandem configuration.
Physical Review Fluids,
Vol. 10,
Issue. 2,
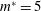 $m^{\ast }=5$ and 50) were studied, and reduced velocity (
$m^{\ast }=5$ and 50) were studied, and reduced velocity ( $V_{r}$) values are chosen inside (
$V_{r}$) values are chosen inside (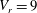 $V_{r}=9$) and outside (
$V_{r}=9$) and outside (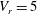 $V_{r}=5$ and
$V_{r}=5$ and 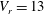 $V_{r}=13$) the lock-in range for low Reynolds numbers. For each combination of
$V_{r}=13$) the lock-in range for low Reynolds numbers. For each combination of  $m^{\ast }$ and
$m^{\ast }$ and 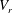 $V_{r}$, a global linear stability analysis was applied to find the critical Reynolds number
$V_{r}$, a global linear stability analysis was applied to find the critical Reynolds number  $Re_{c}$ of the fluid–structure system. For
$Re_{c}$ of the fluid–structure system. For 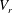 $V_{r}$ in the lock-in range, the values of
$V_{r}$ in the lock-in range, the values of 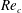 $Re_{c}$ were noticeably less than the critical Reynolds number of the flow around a fixed circular cylinder (
$Re_{c}$ were noticeably less than the critical Reynolds number of the flow around a fixed circular cylinder (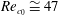 $Re_{c_{0}}\cong 47$). On the other hand, for
$Re_{c_{0}}\cong 47$). On the other hand, for 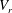 $V_{r}$ outside the lock-in range, the values of
$V_{r}$ outside the lock-in range, the values of 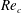 $Re_{c}$ were close to
$Re_{c}$ were close to 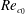 $Re_{c_{0}}$. Next, nonlinear analyses were performed in the vicinity of
$Re_{c_{0}}$. Next, nonlinear analyses were performed in the vicinity of  $Re_{c}$ for each case. Subcritical character (with hysteresis) was observed for
$Re_{c}$ for each case. Subcritical character (with hysteresis) was observed for  $V_{r}$ in the lock-in range, while for
$V_{r}$ in the lock-in range, while for 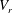 $V_{r}$ outside the lock-in region the bifurcations were found to be supercritical (without hysteresis). This shows that when the coupling between the structure and flow is strong, due to the proximity of the natural frequencies of the isolated systems, it significantly changes both the linear and nonlinear responses observed.
$V_{r}$ outside the lock-in region the bifurcations were found to be supercritical (without hysteresis). This shows that when the coupling between the structure and flow is strong, due to the proximity of the natural frequencies of the isolated systems, it significantly changes both the linear and nonlinear responses observed.

