Published online by Cambridge University Press: 09 November 2016
In this paper, axial interaction of a vortex ring with a thin circular cylinder has been studied. An apparatus to generate clean vortex rings, free of piston and stopping vortex effects, has been used. Flow visualization and particle image velocimetry (PIV) experiments are carried out to determine and compare the characteristics of free and interacting vortex rings in the Reynolds number (defined with the circulation of the free travelling vortex ring) range of 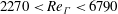 $2270<Re_{\unicode[STIX]{x1D6E4}}<6790$. It is observed that due to the presence of the cylinder, there is an increase in the velocity of the vortex ring. Also, noticeable changes in the characteristic properties of vortex ring such as core circulation, core diameter and ring diameter have been observed. Changes in these parameters are explained by two changes in the flow field between the vortex ring and the cylinder due to axial interactions: (i) displacement of the streamlines and (ii) acceleration in the induced velocity field in this region. These two mutually opposing effects determine the changes in the primary vortex ring properties that take place during interaction. To justify these experimental observations quantitatively, an analytical study of the interaction under an inviscid assumption is performed. The inviscid analysis does predict the increase in velocity during the interaction, but fails to predict the values observed in the present experiments. However, when the theory is used to correct the velocity change through incorporation of the effects of an axisymmetric induced boundary layer region over the cylinder, modelled as an annular vortex sheet of varying strength, the changes in the translational velocities of the vortex rings match closely with the experimental values.
$2270<Re_{\unicode[STIX]{x1D6E4}}<6790$. It is observed that due to the presence of the cylinder, there is an increase in the velocity of the vortex ring. Also, noticeable changes in the characteristic properties of vortex ring such as core circulation, core diameter and ring diameter have been observed. Changes in these parameters are explained by two changes in the flow field between the vortex ring and the cylinder due to axial interactions: (i) displacement of the streamlines and (ii) acceleration in the induced velocity field in this region. These two mutually opposing effects determine the changes in the primary vortex ring properties that take place during interaction. To justify these experimental observations quantitatively, an analytical study of the interaction under an inviscid assumption is performed. The inviscid analysis does predict the increase in velocity during the interaction, but fails to predict the values observed in the present experiments. However, when the theory is used to correct the velocity change through incorporation of the effects of an axisymmetric induced boundary layer region over the cylinder, modelled as an annular vortex sheet of varying strength, the changes in the translational velocities of the vortex rings match closely with the experimental values.