Article contents
Analytical solution for laminar entrance flow in circular pipes
Published online by Cambridge University Press: 25 January 2024
Abstract
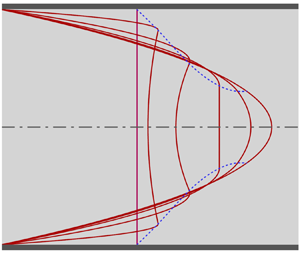
This study introduces an analytical solution for the laminar entrance flow in circular pipes, aiming to confirm the occurrence of velocity overshoot. Velocity overshoot is characterised by the maximum axial velocity appearing near the pipe wall instead of the central axis. Similar to the previous studies, the analytical solution is derived from the parabolised Navier–Stokes equation; however, the specific approach used in linearising the momentum equation has not been attempted before. The accuracy of this analytical solution has been verified through a comprehensive comparison with various published experimental data. The existence of velocity overshoot at a short distance from the inlet, which is evident in numerous numerical calculations based on the full Navier–Stokes equations and corroborated by recent magnetic resonance (MR) velocimetry experiments, is identified analytically for the first time. The parabolised Navier–Stokes equation has inherent self-similarity with respect to the Reynolds number, implying that  $Re$ is incorporated into the dimensionless variables rather than serving as an independent flow parameter. According to both MR velocimetry measurements and the present analytical solution, the self-similarity is not valid immediately following the pipe inlet, and this becomes more evident as
$Re$ is incorporated into the dimensionless variables rather than serving as an independent flow parameter. According to both MR velocimetry measurements and the present analytical solution, the self-similarity is not valid immediately following the pipe inlet, and this becomes more evident as  $Re$ decreases; hence, the analytical solution derived from the parabolised Navier–Stokes equation cannot accurately predict the evolution of the velocity profile within this region near the pipe inlet.
$Re$ decreases; hence, the analytical solution derived from the parabolised Navier–Stokes equation cannot accurately predict the evolution of the velocity profile within this region near the pipe inlet.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press
References
- 1
- Cited by



