Hostname: page-component-599cfd5f84-jhfc5 Total loading time: 0 Render date: 2025-01-07T06:24:06.589Z Has data issue: false hasContentIssue false
  • English
  • Français

An intermittency based Reynolds-averaged transition model for mixing flows induced by interfacial instabilities

Published online by Cambridge University Press:  03 January 2025

Hansong Xie ,
Han Qi ,
Mengjuan Xiao Open the ORCID record for Mengjuan Xiao [Opens in a new window] ,
Yousheng Zhang Open the ORCID record for Yousheng Zhang [Opens in a new window]  and
Yaomin Zhao Open the ORCID record for Yaomin Zhao [Opens in a new window]
Hansong Xie
Affiliation:
HEDPS, Center for Applied Physics and Technology, and College of Engineering, Peking University, Beijing 100871, PR China State Key Laboratory for Turbulence and Complex Systems, College of Engineering, Peking University, Beijing 100871, PR China
Han Qi
Affiliation:
Institute of Applied Physics and Computational Mathematics, Beijing 100094, PR China
Mengjuan Xiao
Affiliation:
Institute of Applied Physics and Computational Mathematics, Beijing 100094, PR China National Key Laboratory of Computational Physics, Beijing 100088, PR China
Yousheng Zhang*
Affiliation:
HEDPS, Center for Applied Physics and Technology, and College of Engineering, Peking University, Beijing 100871, PR China Institute of Applied Physics and Computational Mathematics, Beijing 100094, PR China National Key Laboratory of Computational Physics, Beijing 100088, PR China
Yaomin Zhao*
Affiliation:
HEDPS, Center for Applied Physics and Technology, and College of Engineering, Peking University, Beijing 100871, PR China State Key Laboratory for Turbulence and Complex Systems, College of Engineering, Peking University, Beijing 100871, PR China
*
†Email addresses for correspondence: [email protected], [email protected]
†Email addresses for correspondence: [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

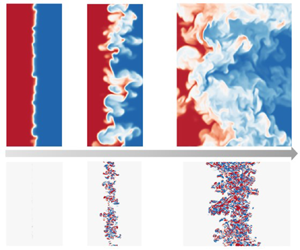
The evolutionary process of mixing induced by Rayleigh–Taylor (RT) and Richtmyer–Meshkov (RM) instabilities typically progresses through three stages: initial instability growth, subsequent mixing transition and ultimate turbulent mixing. Accurate prediction of this entire process is crucial for both scientific research and engineering applications. For engineering applications, Reynolds-averaged Navier–Stokes (RANS) simulation stands as the most viable method currently. However, it is noteworthy that existing RANS mixing models are primarily tailored for the fully developed turbulent mixing stage, rendering them ineffective in predicting the crucial mixing transition. To address that, the present study proposes a RANS mixing transition model. Specifically, we extend the idea of the intermittent factor, which has been widely employed to integrate with turbulence models for predicting boundary layer transition, to mixing problems. Based on a high-fidelity simulation of a RT case, the intermittent factor defined based on enstrophy is extracted and then applied to RANS calculations, showing that it is possible to accurately predict mixing transition by introducing the intermittent factor to the turbulence production from the baseline K-L turbulence mixing model. Furthermore, to facilitate practical predictions, a transport equation has been established to model the spatio-temporal evolution of the intermittent factor. Coupled with the K-L model, the intermittent factor provided by the transport equation is applied to modify the Reynolds stress in RANS calculations. Thereafter, the present transition model has been validated in a series of tests, demonstrating its accuracy and robustness in the capturing mixing process in different types and stages of interfacial mixing flows.

JFM classification

Instability: Transition to turbulence Mixing: Turbulent mixing Turbulent Flows: Turbulence modelling
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 1002 , 10 January 2025 , A31
Check for updates
Copyright
© The Author(s), 2025. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Andrews, M.J., Youngs, D.L., Livescu, D. & Wei, T. 2014 Computational studies of two-dimensional Rayleigh–Taylor driven mixing for a tilted-rig. J. Fluids Engng 136 (9), 091212.CrossRefGoogle Scholar
Bin, Y., Xiao, M., Shi, Y., Zhang, Y. & Chen, S. 2021 A new idea to predict reshocked Richtmyer–Meshkov mixing: constrained large-eddy simulation. J. Fluid Mech. 918, R1.CrossRefGoogle Scholar
Braun, N. & Gore, R.A. 2020 A passive model for the evolution of subgrid-scale instabilities in turbulent flow regimes. Physica D 404, 132373.CrossRefGoogle Scholar
Burrows, A. 2000 Supernova explosions in the Universe. Nature 403 (6771), 727–733.CrossRefGoogle ScholarPubMed
Cabot, W. & Cook, A. 2006 Reynolds number effects on Rayleigh–Taylor instability with possible implications for type Ia supernovae. Nat. Phys. 2, 562–568.CrossRefGoogle Scholar
Canfield, J., Denissen, N., Francois, M., Gore, R., Rauenzahn, R., Reisner, J. & Shkoller, S. 2020 A comparison of interface growth models applied to Rayleigh–Taylor and Richtmyer–Meshkov instabilities. J. Fluids Engng 142 (12), 121108.CrossRefGoogle Scholar
Denissen, N., Rollin, B., Reisner, J. & Andrews, M. 2014 The tilted rocket rig: a Rayleigh–Taylor test case for RANS models. J. Fluids Engng 136, 091301.CrossRefGoogle Scholar
Dimonte, G. & Tipton, R. 2006 K-L turbulence model for the self-similar growth of the Rayleigh–Taylor and Richtmyer–Meshkov instabilities. Phys. Fluids 18 (8), 85101.CrossRefGoogle Scholar
Dimotakis, P.E. 2000 The mixing transition in turbulent flows. J. Fluid Mech. 409, 69–98.CrossRefGoogle Scholar
Gauding, M., Bode, M., Brahami, Y., Varea, E. & Danaila, L. 2021 Self-similarity of turbulent jet flows with internal and external intermittency. J. Fluid Mech. 919, A41.CrossRefGoogle Scholar
Goncharov, V.N. 2002 Analytical model of nonlinear, single-mode, classical Rayleigh–Taylor instability at arbitrary Atwood numbers. Phys. Rev. Lett. 88, 134502.CrossRefGoogle ScholarPubMed
Haan, S.W. 1991 Weakly nonlinear hydrodynamic instabilities in inertial fusion. Phys. Fluids B 3 (8), 2349–2355.CrossRefGoogle Scholar
Hahn, M., Drikakis, D., Youngs, D.L. & Williams, R.J.R. 2011 Richtmyer–Meshkov turbulent mixing arising from an inclined material interface with realistic surface perturbations and reshocked flow. Phys. Fluids 23 (4), 046101.CrossRefGoogle Scholar
Haines, B.M., Grinstein, F.F. & Schwarzkopf, J.D. 2013 Reynolds-averaged Navier–Stokes initialization and benchmarking in shock-driven turbulent mixing. J. Turbul. 14 (2), 46–70.CrossRefGoogle Scholar
Helmholtz, V. 1868 On discontinuous movements of fluids. Lond. Edinb. Dubl. Phil. Mag. J. Sci. 36 (244), 337–346.CrossRefGoogle Scholar
Holzner, M., Liberzon, A., Nikitin, N., Luthi, B., Kinzelbach, W. & Tsinober, A. 2008 A lagrangian investigation of the small-scale features of turbulent entrainment through particle tracking and direct numerical simulation. J. Fluid Mech. 598, 465–475.CrossRefGoogle Scholar
Hu, Z., Zhang, Y. & Tian, B. 2020 Evolution of Rayleigh–Taylor instability under interface discontinuous acceleration induced by radiation. Phys. Rev. E 101, 043115.CrossRefGoogle ScholarPubMed
Jiang, G.-S. & Shu, C.-W. 1996 Efficient implementation of weighted ENO schemes. J. Comput. Phys. 126 (1), 202–228.CrossRefGoogle Scholar
Kelvin, L. 1871 Hydrokinetic solutions and observations. Lond. Edinb. Dubl. Phil. Mag. J. Sci. 42 (281), 362–377.Google Scholar
Kokkinakis, I.W., Drikakis, D. & Youngs, D.L. 2019 Modeling of Rayleigh–Taylor mixing using single-fluid models. Phys. Rev. E 99, 013104.CrossRefGoogle ScholarPubMed
Kokkinakis, I.W., Drikakis, D. & Youngs, D.L. 2020 Two-equation and multi-fluid turbulence models for Richtmyer–Meshkov mixing. Phys. Fluids 32 (7), 074102.CrossRefGoogle Scholar
Kokkinakis, I.W., Drikakis, D., Youngs, D.L. & Williams, R.J.R. 2015 Two-equation and multi fluid turbulence models for Rayleigh–Taylor mixing. Intl J. Heat Fluid Flow 56, 233–250.CrossRefGoogle Scholar
Layzer, D. 1955 On the instability of superposed fluids in a gravitational field. Astrophys. J. 122, 1.CrossRefGoogle Scholar
Li, H., He, Z., Zhang, Y. & Tian, B. 2019 On the role of rarefaction/compression waves in Richtmyer–Meshkov instability with reshock. Phys. Fluids 31 (5), 054102.CrossRefGoogle Scholar
Li, Z., Ju, Y. & Zhang, C. 2023 Machine-learning data-driven modeling of laminar-turbulent transition in compressor cascade. Phys. Fluids 35, 085133.Google Scholar
Liu, C., Wu-Wang, H., Zhang, Y. & Xiao, Z. 2023 A decoupled mechanism of interface growth in single-mode hydrodynamic instabilities. J. Fluid Mech. 964, A37.CrossRefGoogle Scholar
Liu, C., Zhang, Y. & Xiao, Z. 2022 A unified theoretical model for spatiotemporal development of Rayleigh–Taylor and Richtmyer–Meshkov fingers. J. Fluid Mech. 954, A13.CrossRefGoogle Scholar
Livescu, D. 2013 Numerical simulations of two-fluid turbulent mixing at large density ratios and applications to the Rayleigh–Taylor instability. Phil. Trans. R. Soc. A 371 (2003), 20120185.CrossRefGoogle Scholar
Livescu, D., Ristorcelli, J., Gore, R., Dean, S., Cabot, W. & Cook, A. 2009 High-Reynolds number Rayleigh–Taylor turbulence. J. Turbul. 10, N13.CrossRefGoogle Scholar
Livescu, D., Wei, T. & Brady, P.T. 2021 Rayleigh–Taylor instability with gravity reversal. Physica D 417, 132832.CrossRefGoogle Scholar
Menter, F.R., Esch, T. & Kubacki, S. 2002 Transition modelling based on local variables. In Engineering Turbulence Modelling and Experiments 5 (ed. W. Rodi & N. Fueyo), pp. 555–564. Elsevier Science.CrossRefGoogle Scholar
Menter, F.R., Langtry, R.B., Likki, S.R., Suzen, Y.B., Huang, P.G. & Völker, S. 2006 A correlation-based transition model using local variables. Part I. Model formulation. J. Turbomach. 128 (3), 413–422.CrossRefGoogle Scholar
Meshkov, E.E. 1969 Instability of the interface of two gases accelerated by a shock wave. Fluid Dyn. 4 (5), 101–104.CrossRefGoogle Scholar
Mikaelian, K.O. 1998 Analytic approach to nonlinear Rayleigh–Taylor and Richtmyer–Meshkov instabilities. Phys. Rev. Lett. 80, 508–511.CrossRefGoogle Scholar
Morán-López, J. & Schilling, O. 2013 Multicomponent Reynolds-averaged Navier–Stokes simulations of reshocked Richtmyer–Meshkov instability-induced mixing. High Energy Density Phys. 9, 112–121.CrossRefGoogle Scholar
Morgan, B. & Greenough, J. 2015 Large-eddy and unsteady RANS simulations of a shock-accelerated heavy gas cylinder. Shock Waves 26, 355–383.Google Scholar
Ni, W., Zeng, Q. & Zhang, Y. 2023 Dependence of high-density-ratio Rayleigh–Taylor spike on initial perturbations. Acta Mech. Sin. 39 (3), 322181.CrossRefGoogle Scholar
Rayleigh, L. 1882 Investigation of the character of the equilibrium of an incompressible heavy fluid of variable density. Proc. Lond. Math. Soc. 201 (1), 170–177.CrossRefGoogle Scholar
Richtmyer, R.D. 1960 Taylor instability in shock acceleration of compressible fluids. Commun. Pure Appl. Maths 13 (2), 297–319.CrossRefGoogle Scholar
Rollin, B. & Andrews, M.J. 2013 On generating initial conditions for turbulence models: the case of Rayleigh–Taylor instability turbulent mixing. J. Turbul. 14 (3), 77–106.CrossRefGoogle Scholar
Rosafio, N., Lopes, G., Salvadori, S., Lavagnoli, S., Be, L. & Misul, D. 2023 RANS prediction of losses and transition onset in a high-speed low-pressure turbine cascade. Energies 16, N21.CrossRefGoogle Scholar
da Silva, C.B., Hunt, J.C.R., Eames, I. & Westerweel, J. 2014 Interfacial layers between regions of different turbulence intensity. Annu. Rev. Fluid Mech. 46, 567–590.CrossRefGoogle Scholar
Silva, T.S., Zecchetto, M. & Da Silva, C.B. 2018 The scaling of the turbulent/non-turbulent interface at high Reynolds numbers. J. Fluid Mech. 843, 156–179.CrossRefGoogle Scholar
Taylor, G. 1950 The instability of liquid surfaces when accelerated in a direction perpendicular to their planes. I. Proc. R. Soc. Lond. A 201 (1065), 192.Google Scholar
Thomas, V.A. & Kares, R.J. 2012 Drive asymmetry and the origin of turbulence in an ICF implosion. Phys. Rev. Lett. 109 (7), 075004.CrossRefGoogle Scholar
Thornber, B., Drikakis, D., Williams, R.J.R. & Youngs, D. 2008 a On entropy generation and dissipation of kinetic energy in high-resolution shock-capturing schemes. J. Comput. Phys. 227 (10), 4853–4872.CrossRefGoogle Scholar
Thornber, B., Mosedale, A., Drikakis, D., Youngs, D. & Williams, R.J.R. 2008 b An improved reconstruction method for compressible flows with low mach number features. J. Comput. Phys. 227 (10), 4873–4894.CrossRefGoogle Scholar
Toro, E.F., Spruce, M. & Speares, W. 1994 Restoration of the contact surface in the HLL-Riemann solver. Shock Waves 4, 25–34.CrossRefGoogle Scholar
Vetter, M. & Sturtevant, B. 1995 Experiments on the Richtmyer–Meshkov instability of an air/SF6 interface. Shock Waves 4 (5), 247–252.CrossRefGoogle Scholar
Wang, L. & Fu, S. 2011 Development of an intermittency equation for the modeling of the supersonic/hypersonic boundary layer flow transition. Flow Turbul. Combust. 87 (1), 165–187.CrossRefGoogle Scholar
Wang, R., Song, Y., Ma, Z., Ma, D., Wang, L. & Wang, P. 2022 The transition to turbulence in rarefaction-driven Rayleigh–Taylor mixing: effects of diffuse interface. Phys. Fluids 34 (1), 015125.CrossRefGoogle Scholar
Watanabe, T., Da Silva, C.B. & Nagata, K. 2019 Non-dimensional energy dissipation rate near the turbulent/non-turbulent interfacial layer in free shear flows and shear free turbulence. J. Fluid Mech. 875, 321–344.CrossRefGoogle Scholar
Wei, T. & Livescu, D. 2012 Late-time quadratic growth in single-mode Rayleigh–Taylor instability. Phys. Rev. E 86, 046405.CrossRefGoogle ScholarPubMed
Xiao, M., Hu, Z., Dai, Z. & Zhang, Y. 2022 Experimentally consistent large-eddy simulation of re-shocked Richtmyer–Meshkov turbulent mixing. Phys. Fluids 34 (12), 125125.CrossRefGoogle Scholar
Xiao, M., Zhang, Y. & Tian, B. 2020 Modeling of turbulent mixing with an improved K-L model. Phys. Fluids 32 (9), 092104.CrossRefGoogle Scholar
Xiao, M., Zhang, Y. & Tian, B. 2021 A K-L model with improved realizability for turbulent mixing. Phys. Fluids 33 (2), 022104.CrossRefGoogle Scholar
Xie, H., Zhao, Y. & Zhang, Y. 2023 Data-driven nonlinear K-L turbulent mixing model via gene expression programming method. Acta Mech. Sin. 39 (2), 322315.CrossRefGoogle Scholar
Xie, H.-S., Xiao, M.-J. & Zhang, Y.-S. 2021 Unified prediction of turbulent mixing induced by interfacial instabilities via Besnard-Harlow-Rauenzahn-2 model. Phys. Fluids 33 (10), 105123.CrossRefGoogle Scholar
Youngs, D.L. 2013 The density ratio dependence of self-similar Rayleigh–Taylor mixing. Phil. Trans. R. Soc. A 371 (2003), 20120173.CrossRefGoogle ScholarPubMed
Zhang, Q. & Guo, W. 2016 Universality of finger growth in two-dimensional Rayleigh–Taylor and Richtmyer–Meshkov instabilities with all density ratios. J. Fluid Mech. 786, 47–61.CrossRefGoogle Scholar
Zhang, Q. & Guo, W. 2022 Quantitative theory for spikes and bubbles in the Richtmyer–Meshkov instability at arbitrary density ratios. Phys. Rev. Fluids 7, 093904.CrossRefGoogle Scholar
Zhang, Y., He, Z., Xie, H., Xiao, M. & Tian, B. 2020 Methodology for determining coefficients of turbulent mixing model. J. Fluid Mech. 905, A26.CrossRefGoogle Scholar
Zhang, Y. & Ni, W. 2023 Unified 2D/3D bubble merger model for Rayleigh–Taylor mixing. Acta Mech. Sin. 39 (5), 322199.CrossRefGoogle Scholar
Zhou, Y. 2007 Unification and extension of the similarity scaling criteria and mixing transition for studying astrophysics using high energy density laboratory experiments or numerical simulations. Phys. Plasmas 14 (8), 82701.CrossRefGoogle Scholar
Zhou, Y., Robey, H. & Buckingham, A. 2003 Onset of turbulence in accelerated high-Reynolds-number flow. Phys. Rev. E 67, 056305.CrossRefGoogle ScholarPubMed
Zhou, Z.-R., Zhang, Y.-S. & Tian, B.-L. 2018 Dynamic evolution of Rayleigh–Taylor bubbles from sinusoidal, W-shaped, and random perturbations. Phys. Rev. E 97, 033108.CrossRefGoogle ScholarPubMed