Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-12-02T22:20:04.546Z Has data issue: false hasContentIssue false
  • English
  • Français

Air-assisted atomization of liquid jets in varying levels of turbulence

Published online by Cambridge University Press:  23 December 2014

A. Kourmatzis  and
A. R. Masri
A. Kourmatzis*
Affiliation:
Combustion Research Group, Aerospace Mechanical and Mechatronic Engineering, The University of Sydney, NSW 2006, Australia
A. R. Masri
Affiliation:
Combustion Research Group, Aerospace Mechanical and Mechatronic Engineering, The University of Sydney, NSW 2006, Australia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Air-assisted primary atomization is investigated in a configuration where liquid is injected in a turbulent gaseous jet flow both within as well as outside of the potential core. Cases are studied where the injection point is moved within the flow to maintain a range of constant gaseous mean velocities but changing local fluctuating velocity root-mean-square (r.m.s.) levels. Over a range of mean conditions, this allows for a systematic understanding of both the effects of gas-phase turbulence and mean shear on primary break-up independently. Extensive data is obtained and analysed from laser Doppler anemometry/phase Doppler anemometry, high-speed microscopic backlit imaging and advanced image processing. It is found that the ratio of the turbulent Weber number 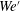$\mathit{We}^{\prime }$ to the mean Weber number $\mathit{We}$ is a relevant parameter as is the turbulence intensity. The primary break-up length is found to be heavily influenced not only by the mean velocity, but also by the turbulence level and the mass fuel to air ratio. Above a particular threshold intensity level the break-up time changes in proportion to the change in the integral time scale of the flow. In addition, it is found that regardless of diameter and turbulent flow conditions at the liquid jet, the final size of ligaments converges to a value which is of the order of the measured primary instability wavelength (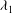${\it\lambda}_{1}$). In contrast, cases of different turbulence intensity show the mean of droplet sizes diverging as the spray is advected downstream and this is because droplets are generated from ligaments, the latter of which are subjected both to Rayleigh–Taylor instabilities and turbulent fluctuations. This contribution, for the first time, examines the theoretical applicability of the Rayleigh–Taylor instability in flows where the turbulence is substantial with respect to the mean flow. It is shown that for high turbulence intensities a full theoretical reconstruction of the measured final droplet size distribution is possible from a probability density function of model Rayleigh–Taylor wavelengths (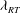${\it\lambda}_{RT}$). In agreement with the literature (Varga et al. J. Fluid Mech., vol. 497, 2003, pp. 405–434), mean droplet sizes are found to be equal to a mean theoretical Rayleigh–Taylor wavelength normalized by a particular constant value. This, however, is only true for local turbulence intensities less than 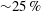${\sim}25\,\%$, or for ratios of the turbulent Weber number to mean Weber number (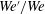$\mathit{We}^{\prime }/\mathit{We}$) of less than ${\sim}6\,\%$. Above this, the normalization value is no longer constant, but increases with 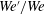$\mathit{We}^{\prime }/\mathit{We}$. Finally, the instability wavelengths can be used as part of an approximation that estimates the total number of objects formed after break-up, where the object number is found to be dictated by a balance of both mean flow conditions and local turbulence.

JFM classification

Drops and Bubbles: Breakup/coalescence Drops and Bubbles: Drops and Bubbles
Type
Papers
Information
Journal of Fluid Mechanics , Volume 764 , 10 February 2015 , pp. 95 - 132
Check for updates
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Antonia, R. A. & Bilger, R. W. 1973 An experimental investigation of an axisymmetric jet in a co-flowing air stream. J. Fluid Mech. 61, 805–822.Google Scholar
Arcoumanis, C., Whitelaw, D. S. & Whitelaw, J. H. 1996 Breakup of Newtonian and non-Newtonian fluids. Atomiz. Sprays 6, 245–256.Google Scholar
Beale, J. D. & Reitz, R. D. 1999 Modeling spray atomisation with the Kelvin–Helmholtz/Rayleigh–Taylor hybrid model. Atomiz. Sprays 9, 623–650.Google Scholar
Chigier, N. & Reitz, R. D. 1995 Regimes of jet breakup and breakup mechanisms (physical aspects). In Recent Advances in Spray Combustion: Spray Atomization and Drop Burning Phenomena, vol. 1, pp. 109–135. American Institute of Aeronautics and Astronautics.Google Scholar
Desjardins, O., Moureau, V. & Pitsch, H. 2008 An accurate level set/ghost fluid method for simulating turbulent atomization. J. Comput. Phys. 227, 8395–8416.CrossRefGoogle Scholar
Dumouchel, C. 2008 On the experimental investigation on primary atomization of liquid streams. Exp. Fluids 45, 371–422.CrossRefGoogle Scholar
Engelbert, C., Hardalupas, Y. & Whitelaw, J. H. 1995 Breakup phenomena in coaxial airblast atomizers. Proc. R. Soc. Lond. A 451, 189–229.Google Scholar
Faeth, G. M., Hsiang, L. P. & Wu, P. K. 1995 Structure and breakup properties of sprays. Intl J. Multiphase Flow 21, 99–127.Google Scholar
Ghaemi, S., Rahimi, P. & Nobes, D. S. 2009 Assessment of parameters for distinguishing droplet shape in a spray field using image-based techniques. Atomiz. Sprays 19, 809–831.Google Scholar
Gorokhovski, M. & Herrmann, M. 2008 Modeling primary atomization. Annu. Rev. Fluid Mech. 40, 343–366.CrossRefGoogle Scholar
Gounder, J. D., Kourmatzis, A. & Masri, A. R. 2012 Turbulent piloted dilute spray flames: flow fields and droplet dynamics. Combust. Flame 159, 3372–3397.Google Scholar
Guildenbecher, D. R., Lopez-Rivera, C. & Sojka, P. E. 2009 Secondary atomization. Exp. Fluids 46, 371–402.Google Scholar
Herrmann, M. 2011 The influence of density ratio on the primary atomization of a turbulent liquid jet in crossflow. Proc. Combust. Inst. 33, 2079–2088.CrossRefGoogle Scholar
Hinze, J. O. 1955 Fundamentals of the hydrodynamic mechanism of splitting in dispersion processes. AIChE J. 1, 289–295.Google Scholar
Hsiang, L. P. & Faeth, G. M. 1992 Near-limit drop deformation and secondary breakup. Intl J. Multiphase Flow 18, 635–652.Google Scholar
Hsiang, L. P. & Faeth, G. M. 1995 Drop deformation and breakup due to shock wave and steady disturbances. Intl J. Multiphase Flow 21, 545–560.CrossRefGoogle Scholar
Ju, D., Shrimpton, J. S. & Hearn, A. 2012 A multi-thresholding algorithm for sizing out of focus particles. Part. Part. Syst. Charact. 29, 78–92.Google Scholar
Kolmogorov, A. N. 1949 On the disintegration of drops in a turbulent flow. Dokl. Akad. Nauk SSSR 66, 825–828.Google Scholar
Kourmatzis, A. & Masri, A. R. 2014 The influence of gas phase velocity fluctuations on primary atomization and droplet deformation. Exp. Fluids 55 (2), 1–20.CrossRefGoogle Scholar
Kourmatzis, A., Pham, P. X. & Masri, A. R. 2013 Air assisted atomization and spray density characterization of ethanol and a range of biodiesels. Fuel 108, 758–770.CrossRefGoogle Scholar
Kourmatzis, A., Pham, P. X. & Masri, A. R. 2014 Characterization of atomization and combustion in moderately dense turbulent spray flames. Combust. Flame (in press), doi:10.1016/j.combustflame.2014.09.021.Google Scholar
Lasheras, J. C. & Hopfinger, E. J. 2000 Liquid jet instability and atomization in a coaxial gas stream. Annu. Rev. Fluid Mech. 32, 275–308.Google Scholar
Lasheras, J. C., Villermaux, E. & Hopfinger, E. J. 1998 Break-up and atomization of a round water jet by a high-speed annual air jet. J. Fluid Mech. 357, 351–379.CrossRefGoogle Scholar
Lee, C. S. & Park, S. W. 2002 An experimental and numerical study of fuel atomisation characteristics of high-pressure diesel injection sprays. Fuel 81, 2417–2423.CrossRefGoogle Scholar
Lee, C. S. & Reitz, R. D. 1993 An experimental study of the effect of gas density on the distortion and breakup mechanism of drops in high speed gas stream. Intl J. Multiphase Flow 3, 55–75.Google Scholar
Lee, C. S. & Reitz, R. D. 2001 Effect of liquid properties on the breakup mechanism of high-speed liquid drops. Atomiz. Sprays 11, 1–19.Google Scholar
Lin, S. P. & Lian, Z. W. 1990 Mechanisms of the breakup of liquid jets. AIAA J. 28, 120–126.Google Scholar
Liu, H.-F., Li, W.-H., Gong, X., Cao, X.-K., Xu, J.-L., Chen, X.-L., Wang, Y.-F., Yu, G.-S., Wang, F.-C. & Yu, Z.-H. 2006 Effect of liquid jet diameter on performance of coaxial two-fluid airblast atomizers. Chem. Engng Prog. 45, 240–245.Google Scholar
Liu, A. B. & Reitz, R. D. 2000 Mechanisms of air-assisted liquid atomization. Atomiz. Sprays 26, 229–244.Google Scholar
Lorenzetto, G. E. & Lefebvre, A. H. 1977 Measurements of drop size on a plain-jet air blast atomiser. AIAA J. 15, 1006–1010.Google Scholar
Nukiyama, G. E. & Tanasawa, Y. 1939 Experiments on the atomisation of liquids by means of air streams, parts III and IV. Trans. Soc. Mech. Engng Japan 5, 63–75.Google Scholar
Raynal, L.1997 Instabilite et entrainment a l’interface d’une couche de melange liquid-gaz. PhD thesis, Universite Joseph Fourier, Grenoble, France.Google Scholar
Rizkalla, A. A. & Lefebvre, A. H. 1975 The influence of air and liquid properties on air blast atomisation. Trans. ASME J. Fluids Engng 97, 316–320.Google Scholar
Sevik, M. & Park, S. H. 1973 The splitting of drops and bubbles by turbulent fluid flow. Trans. ASME J. Fluids Engng 95, 53–60.Google Scholar
Sharp, D. H. 1984 An overview of Rayleigh–Taylor instability. Physica D 12, 3–10.Google Scholar
Shavit, U. 2001 Gas–liquid interaction in the liquid breakup region of twin-fluid atomization. Exp. Fluids 31, 550–557.CrossRefGoogle Scholar
Shinzo, J. & Umemura, A. 2010 Simulation of liquid jet primary breakup: dynamics of ligament and droplet formation. Intl J. Multiphase Flow 36, 513–532.Google Scholar
Shinzo, J. & Umemura, A. 2011 Surface instability and primary atomization characteristics of straight liquid jet sprays. Intl J. Multiphase Flow 37, 1294–1304.Google Scholar
Tamaki, H., Myojin, H. & Yamaji, T.2011 Effects of construction and supply methods of atomising air of air-blast atomiser for aero-engine on spray characteristics. The 24th Annual Conference on Liquid Atomization and Spray Systems, Estoril, Portugal. ILASS.Google Scholar
Varga, C. M., Lasheras, J. C. & Hopfinger, E. J. 2003 Initial breakup of a small-diameter liquid jet by a high-speed gas stream. J. Fluid Mech. 497, 405–434.Google Scholar
Villermaux, E. 1998 Mixing and spray formation in coaxial jets. J. Propul. Power 14, 807–817.Google Scholar