Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-28T18:51:04.273Z Has data issue: false hasContentIssue false
  • English
  • Français

The aeroacoustics of finite wall-mounted square cylinders

Published online by Cambridge University Press:  26 October 2017

Ric Porteous ,
Danielle J. Moreau Open the ORCID record for Danielle J. Moreau [Opens in a new window]  and
Con J. Doolan
Ric Porteous
Affiliation:
School of Mechanical Engineering, The University of Adelaide, SA 5005 Australia
Danielle J. Moreau*
Affiliation:
School of Mechanical and Manufacturing Engineering, UNSW Sydney, NSW 2052 Australia
Con J. Doolan
Affiliation:
School of Mechanical and Manufacturing Engineering, UNSW Sydney, NSW 2052 Australia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

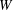This paper presents the results of an experimental study that relates the flow structures in the wake of a square finite wall-mounted cylinder with the radiated noise. Acoustic and hot-wire measurements were taken in an anechoic wind tunnel. The cylinder was immersed in a near-zero-pressure gradient boundary layer whose thickness was 130 % of the cylinder width, $W$. Aspect ratios were in the range $0.29\leqslant L/W\leqslant 22.9$ (where 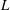$L$ is the cylinder span), and the Reynolds number, based on width, was $1.4\times 10^{4}$. Four shedding regimes were identified, namely R0 (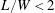$L/W<2$), RI (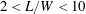$2<L/W<10$), RII (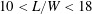$10<L/W<18$) and RIII (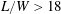$L/W>18$), with each shedding regime displaying an additional acoustic tone as the aspect ratio was increased. At low aspect ratios (R0 and RI), downwash dominated the wake, creating a highly three-dimensional shedding environment with maximum downwash at 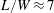$L/W\approx 7$. Looping vortex structures were visualised using a phase eduction technique. The principal core of the loops generated the most noise perpendicular to the cylinder. For higher aspect ratios in RII and RIII, the main noise producing structures consisted of a series of inclined vortex filaments, where the angle of inclination varied between vortex cells.

JFM classification

Acoustics: Aeroacoustics Vortex Flows: Vortex shedding
Type
Papers
Information
Journal of Fluid Mechanics , Volume 832 , 10 December 2017 , pp. 287 - 328
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Amiet, R. K. 1975 Correction of open jet wind tunnel measurements for shear layer refraction. In 2nd AIAA Aeroacoustics Conference. AIAA Paper 75-532.Google Scholar
Becker, S., Hahn, C., Kaltenbacher, M. & Lerch, R. 2008 Flow-induced sound of wall-mounted cylinders with different geometries. AIAA J. 46 (9), 2265–2281.CrossRefGoogle Scholar
Bendat, J. S. & Piersol, A. G. 2010 Random Data: Analysis and Measurement Procedures, 4th edn. Wiley.CrossRefGoogle Scholar
Bourgeois, J. A.2012 Three-dimensional topology and dynamical modelling of vortex shedding from finite surface-mounted bluff bodies. PhD thesis, University of Calgary.Google Scholar
Bourgeois, J. A., Noack, B. R. & Martinuzzi, R. J. 2013 Generalized phase average with applications to sensor-based flow estimation of the wall-mounted square cylinder wake. J. Fluid Mech. 736, 316–350.CrossRefGoogle Scholar
Bourgeois, J. A., Sattari, P. & Martinuzzi, R. J. 2010 Quasi-periodic structure of vortical flows produced in the wake of finite bluff bodies partially immersed in a boundary layer. In ASME 2010 3rd Joint US–European Fluids Engineering Summer Meeting: Volume 1, Symposia Parts A, B, and C, pp. 2827–2836. ASME.Google Scholar
Bourgeois, J. A., Sattari, P. & Martinuzzi, R. J. 2011 Alternating half-loop shedding in the turbulent wake of a finite surface-mounted square cylinder with a thin boundary layer. Phys. Fluids 23 (9), 095101.CrossRefGoogle Scholar
Bradbury, L. J. S. 1976 Measurements with a pulsed-wire and a hot-wire anemometer in the highly turbulent wake of a normal flat plate. J. Fluid Mech. 77 (03), 473–497.CrossRefGoogle Scholar
Bruun, H. H. 1995 Hot-Wire Anemometry: Principles and Signal Analysis. Oxford University Press.CrossRefGoogle Scholar
Casalino, D. & Jacob, M. 2003 Prediction of aerodynamic sound from circular rods via spanwise statistical modelling. J. Sound Vib. 262, 815–844.CrossRefGoogle Scholar
Coles, D. 1956 The law of the wake in the turbulent boundary layer. J. Fluid Mech. 1 (02), 191–226.CrossRefGoogle Scholar
Curle, N. 1955 The influence of solid boundaries upon aerodynamic sound. Proc. R. Soc. Lond. A 231 (1187), 505–514.Google Scholar
van Driest, E. R. 1956 On turbulent flow near a wall. J. Aero. Sci. 23 (11), 1007–1011.Google Scholar
Fox, T. A. & West, G. S. 1990 On the use of end plates with circular cylinders. Exp. Fluids 9, 237–239.CrossRefGoogle Scholar
Fox, T. A. & West, G. S. 1993 Fluid-induced loading of cantilevered circular cylinders in a low-turbulence uniform flow. Part 1: mean loading with aspect ratios in the range 4–30. J. Fluids Struct. 7 (1), 1–14.CrossRefGoogle Scholar
Fujita, H. 2010 The characteristics of the Aeolian tone radiated from two-dimensional cylinders. Fluid Dyn. Res. 42 (1), 1–25.CrossRefGoogle Scholar
Fujita, H., Sha, W., Furutani, H. & Suzuki, H. 1998 Experimental investigation and prediction of aerodynamic sound generated from square cylinders. AIAA Paper 98-30896.Google Scholar
Gerich, D. & Eckelmann, H. 1982 Influence of end plate and free ends on the shedding frequency of circular cylinders. J. Fluid Mech. 122, 109–121.CrossRefGoogle Scholar
Gerrard, J. H. 1966 The mechanics of the formation region of vortices behind bluff bodies. J. Fluid Mech. 25, 401–413.CrossRefGoogle Scholar
Hassan, M. E., Bourgeois, J. A. & Martinuzzi, R. 2015 Boundary layer effect on the vortex shedding of a wall-mounted rectangular cylinder. Exp. Fluids 56, 33.CrossRefGoogle Scholar
Hosseini, Z., Bourgeois, J. A. & Martinuzzi, R. J. 2013 Large-scale structures in dipole and quadrupole wakes of a wall-mounted finite rectangular cylinder. Exp. Fluids 54.CrossRefGoogle Scholar
Hutcheson, F. V. & Brooks, T. F. 2006 Noise radiation from single and multiple rod configurations. In 12th AIAA/CEAS Aeroacoustics Conference. AIAA Paper-2006-2629.Google Scholar
Kawamura, T., Hiwada, M., Hibino, T., Mabuchi, I. & Kumada, M. 1984 Flow around a finite circular cylinder on a flat plate (cylinder height greater than turbulent boundary layer thickness). JSME 27 (232), 2142–2151.CrossRefGoogle Scholar
Keefe, R. T.1961 An investigation of the fluctuating forces acting on a stationary circular cylinder in a subsonic stream, and of the associated sound field. Tech. Rep. UTIA.CrossRefGoogle Scholar
King, W. F. & Pfizenmaier, E. 2009 An experimental study of sound generated by flow around cylinders of different cross-section. J. Sound Vib. 328 (3), 318–337.CrossRefGoogle Scholar
Leclercq, D. & Doolan, C. J. 2009 The interaction of a bluff body with a vortex wake. J. Fluids Struct. 25 (5), 867–888.CrossRefGoogle Scholar
Lira, I. 2002 Evaluating the Measurement Uncertainty. IOP Publishing Ltd.CrossRefGoogle Scholar
Lyn, D. A., Einav, S., Rod, W. & Park, J. H. 1995 A laser-Doppler velocimetry study of ensemble-averaged characteristics of the turbulent near wake of a square cylinder. J. Fluid Mech. 304, 285–319.CrossRefGoogle Scholar
Moreau, D. & Doolan, C. 2013 Flow-induced sound of wall-mounted finite length cylinders. AIAA J. 51, 2493–2502.CrossRefGoogle Scholar
Moreau, D. J., Brooks, L. A. & Doolan, C. J. 2012 The effect of boundary layer type on trailing edge noise from sharp-edged flat plates at low-to-moderate Reynolds number. J. Sound Vib. 331 (17), 3976–3988.CrossRefGoogle Scholar
Norberg, C. 2003 Fluctuating lift on a circular cylinder: review and new measurements. J. Fluids Struct. 17 (1), 57–96.CrossRefGoogle Scholar
Oguma, Y., Yamagata, T. & Fujisawa, N. 2013 Measurement of sound source distribution around a circular cylinder in a uniform flow by combined particle image velocimetry and microphone technique. J. Wind Engng Ind. Aerodyn. 18, 1–11.Google Scholar
Ogunremi, A. R. & Sumner, D. 2015 The effect of a splitter plate on the flow around a finite prism. J. Fluids Struct. 59, 1–21.CrossRefGoogle Scholar
Passchier-Vermeer, W. & Passchier, W. F. 2000 Noise exposure and public health. Environ. Health Perspectives 108 (1), 123–131.Google ScholarPubMed
Perry, A. E. 1982 Hot-wire Anemometry. Clarendon.Google Scholar
Phillips, O. M. 1956 The intensity of Aeolian tones. J. Fluid Mech. 1 (06), 607–624.CrossRefGoogle Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.CrossRefGoogle Scholar
Porteous, R.2016 The aeroacoustics of finite wall-mounted cylinders. PhD thesis, University of Adelaide, School of Mechanical Engineering.CrossRefGoogle Scholar
Porteous, R., Moreau, D. J. & Doolan, C. J. 2014 A review of flow-induced noise from finite wall-mounted cylinders. J. Fluids Struct. 51, 240–254.CrossRefGoogle Scholar
Rostamy, N., Sumner, D., Bergstrom, D. J. & Bugg, J. D. 2012 Local flow field of a surface-mounted finite circular cylinder. J. Fluids Struct. 34, 105–122.CrossRefGoogle Scholar
Sakamoto, H. & Arie, M. 1983 Vortex shedding from a rectangular prism and a circular cylinder placed vertically in a turbulent boundary layer. J. Fluid Mech. 126, 147–165.CrossRefGoogle Scholar
Sakamoto, H. & Oiwake, S. 1984 Fluctuating forces on a rectangular prism and a circular cylinder placed vertically in a turbulent boundary layer. Trans. ASME 106, 160–166.Google Scholar
Sattari, P., Bourgeois, J. A. & Martinuzzi, R. J. 2012 On the vortex dynamics in the wake of a finite surface-mounted square cylinder. Exp. Fluids 52 (5), 1149–1167.CrossRefGoogle Scholar
Schlinker, R. H. & Fink, M. R. 1976 Vortex noise from nonrotating cylinders and airfoils. In 14th Aerospace Sciences Meeting. AIAA Paper 76-81.Google Scholar
Spalart, P. R. 1988 Direct simulation of a turbulent boundary layer up to r 𝜃 = 1410. J. Fluid Mech. 187, 61–98.CrossRefGoogle Scholar
Sumner, D., Heseltine, J. L. & Dansereau, O. J. P. 2004 Wake structure of a finite circular cylinder of small aspect ratio. Exp. Fluids 37 (5), 720–730.CrossRefGoogle Scholar
Unal, M. F. & Rockwell, D. 1988 On vortex formation from a cylinder. Part 1. The initial instability. J. Fluid Mech. 190, 491–512.CrossRefGoogle Scholar
Vickery, B. J. 1966 Fluctuating lift and drag on a long cylinder of square cross-section in a smooth and in a turbulent stream. J. Fluid Mech. 25 (3), 481–494.CrossRefGoogle Scholar
Wang, H. F., Cao, H. L. & Zhou, Y. 2014 POD analysis of a finite-length cylinder near wake. Exp. Fluids 55, 1790–1805.CrossRefGoogle Scholar
Wang, H. & Zhou, Y. 2009 The finite-length square cylinder near wake. J. Fluid Mech. 638, 453–490.CrossRefGoogle Scholar
Wang, H., Zhou, Y., Chan, C. K. & Lam, K. S. 2006 Effect of initial conditions on interaction between boundary layer and a wall-mounted finite-length-cylinder wake. Phys. Fluids 18, 065106.CrossRefGoogle Scholar
Welch, P. D. 1967 The use of fast Fourier transform for the estimation of power spectra: a method based on time averaging over short, modified periodograms. IEEE Trans. Audio Electroacoust. AU‐15, 70–73.CrossRefGoogle Scholar
Wilcox, D. C. 2006 Turbulence Modeling for CFD. DCW Industries.Google Scholar
Williamson, C. H. K. 1996 Vortex dynamics in the cylinder wake. Annu. Rev. Fluid Mech. 28, 477–539.CrossRefGoogle Scholar
Yoshida, M., Koike, M. & Fukano, T. 2003 A study on the Aeolian tones generated from square cylinders with rounded corners. In 9th AIAA/CEAS Aeroacoustics Conference and Exhibit. AIAA Paper 2003-3218.Google Scholar