Article contents
Work-minimizing kinematics for small displacement of an infinitely long cylinder
Published online by Cambridge University Press: 17 April 2020
Abstract
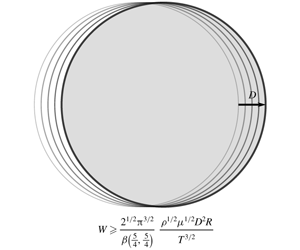
We consider the time-dependent speed of an infinitely long cylinder that minimizes the net work done on the surrounding fluid to travel a given distance perpendicular to its axis in a fixed amount of time. The flow that develops is two-dimensional. An analytical solution is possible using calculus of variations for the case that the distance travelled and the viscous boundary layer thickness that develops are much smaller than the circle radius. If  $t$ represents the time since the commencement of motion and
$t$ represents the time since the commencement of motion and  $T$ the final time, then the optimum speed profile is
$T$ the final time, then the optimum speed profile is  $Ct^{1/4}(T-t)^{1/4}$, where
$Ct^{1/4}(T-t)^{1/4}$, where  $C$ is determined by the distance travelled. The result also holds for rigid-body translations and rotation of cylinders formed by extrusion of smooth but otherwise arbitrary curves.
$C$ is determined by the distance travelled. The result also holds for rigid-body translations and rotation of cylinders formed by extrusion of smooth but otherwise arbitrary curves.
- Type
- JFM Rapids
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
- 1
- Cited by


