Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-29T01:36:12.009Z Has data issue: false hasContentIssue false
  • English
  • Français

Weak magnetohydrodynamic turbulence and intermittency

Published online by Cambridge University Press:  27 March 2015

R. Meyrand ,
K. H. Kiyani  and
S. Galtier
R. Meyrand
Affiliation:
Laboratoire de Physique des Plasmas, École Polytechnique, F-91128 Palaiseau CEDEX, France
K. H. Kiyani
Affiliation:
Laboratoire de Physique des Plasmas, École Polytechnique, F-91128 Palaiseau CEDEX, France Centre for Fusion, Space and Astrophysics, Department of Physics, University of Warwick, Coventry CV4 7AL, UK
S. Galtier*
Affiliation:
Laboratoire de Physique des Plasmas, École Polytechnique, F-91128 Palaiseau CEDEX, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

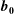Three-dimensional numerical simulation is used to investigate intermittency in incompressible weak magnetohydrodynamic turbulence with a strong uniform magnetic field $\boldsymbol{b}_{\mathbf{0}}$ and zero cross-helicity. At leading order, this asymptotic regime is achieved via three-wave resonant interactions with the scattering of a wave on a 2D mode for which $k_{\Vert }=0$. When the interactions with the 2D modes are artificially reduced, we show numerically that the system exhibits an energy spectrum with 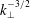$k_{\bot }^{-3/2}$, whereas the expected exact solution with 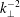$k_{\bot }^{-2}$ is recovered with the full nonlinear system. In the latter case, strong intermittency is found when the vector separation of structure functions is taken transverse to 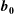$\boldsymbol{b}_{\mathbf{0}}$. This result may be explained by the influence of the 2D modes whose regime belongs to strong turbulence. In addition to shedding light on the origin of this intermittency, we derive a log-Poisson law, ${\it\zeta}_{p}=p/8+1-(1/4)^{p/2}$, which fits the data perfectly and highlights the important role of parallel current sheets.

JFM classification

Turbulent Flows: Intermittency MHD and Electrohydrodynamics: MHD turbulence
Type
Rapids
Information
Journal of Fluid Mechanics , Volume 770 , 10 May 2015 , R1
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abramenko, V. I., Yurchyshyn, V. B., Wang, H., Spirock, T. J. & Goode, P. R. 2002 Scaling behavior of structure functions of the longitudinal magnetic field in active regions on the sun. Astrophys. J. 577, 487–495.CrossRefGoogle Scholar
Batchelor, G. K. & Townsend, A. A. 1949 The nature of turbulent motion at large wavenumbers. Proc. R. Soc. Lond. A 199, 238–255.Google Scholar
Bigot, B., Galtier, S. & Politano, H. 2008a An anisotropic turbulent model for solar coronal heating. Astron. Astrophys. 490, 325–337.CrossRefGoogle Scholar
Bigot, B., Galtier, S. & Politano, H. 2008b Development of anisotropy in incompressible magnetohydrodynamic turbulence. Phys. Rev. E 78 (6), 066301.CrossRefGoogle ScholarPubMed
Biskamp, D. 2003 Magnetohydrodynamic Turbulence. Cambridge University Press.CrossRefGoogle Scholar
van Bokhoven, L. J. A., Clercx, H. J. H., van Heijst, G. J. F. & Trieling, R. R. 2009 Experiments on rapidly rotating turbulent flows. Phys. Fluids 21 (9), 096601.CrossRefGoogle Scholar
Boldyrev, S. & Perez, J. C. 2009 Spectrum of weak magnetohydrodynamic turbulence. Phys. Rev. Lett. 103 (22), 225001.CrossRefGoogle ScholarPubMed
Cabral, B. & Leedom, L. 1993 Imaging vector fields using line integral convolution. In Proceedings of the 20th Annual Conference on Computer Graphics and Interactive Techniques, SIGGRAPH’93, vol. 8, pp. 263–270. ACM.CrossRefGoogle Scholar
Choi, Y., Lvov, Y. V. & Nazarenko, S. 2004 Probability densities and preservation of randomness in wave turbulence. Phys. Lett. A 332, 230–238.CrossRefGoogle Scholar
Denissenko, P., Lukaschuk, S. & Nazarenko, S. 2007 Gravity wave turbulence in a laboratory flume. Phys. Rev. Lett. 99 (1), 014501.CrossRefGoogle Scholar
Douady, S., Couder, Y. & Brachet, M. E. 1991 Direct observation of the intermittency of intense vorticity filaments in turbulence. Phys. Rev. Lett. 67, 983–986.CrossRefGoogle ScholarPubMed
Dyachenko, S., Newell, A. C., Pushkarev, A. & Zakharov, V. E. 1992 Optical turbulence: weak turbulence, condensates and collapsing filaments in the nonlinear Schrödinger equation. Physica D 57, 96–160.CrossRefGoogle Scholar
Falcon, E., Fauve, S. & Laroche, C. 2007 Observation of intermittency in wave turbulence. Phys. Rev. Lett. 98 (15), 154501.Google ScholarPubMed
Galtier, S. 2003 Weak inertial-wave turbulence theory. Phys. Rev. E 68 (1), 015301.CrossRefGoogle ScholarPubMed
Galtier, S. 2006 Multi-scale turbulence in the inner solar wind. J. Low Temp. Phys. 145, 59–74.CrossRefGoogle Scholar
Galtier, S., Nazarenko, S. V., Newell, A. C. & Pouquet, A. 2000 A weak turbulence theory for incompressible magnetohydrodynamics. J. Plasma Phys. 63, 447–488.CrossRefGoogle Scholar
Galtier, S., Nazarenko, S. V., Newell, A. C. & Pouquet, A. 2002 Anisotropic turbulence of shear-Alfvén waves. Astrophys. J. Lett. 564, L49–L52.CrossRefGoogle Scholar
Janssen, P. A. E. M. 2003 Nonlinear four-wave interactions and freak waves. J. Phys. Oceanogr. 33, 863–884.2.0.CO;2>CrossRefGoogle Scholar
Kiyani, K. H., Chapman, S. C., Sahraoui, F., Hnat, B., Fauvarque, O. & Khotyaintsev, Y. V. 2013 Enhanced magnetic compressibility and isotropic scale invariance at sub-ion larmor scales in solar wind turbulence. Astrophys. J. 763, 10.CrossRefGoogle Scholar
Kolmogorov, A. N. 1962 A refinement of previous hypotheses concerning the local structure of turbulence in a viscous incompressible fluid at high Reynolds number. J. Fluid Mech. 13, 82–85.CrossRefGoogle Scholar
Kraichnan, R. H. 1965 Inertial range spectrum in hydromagnetic turbulence. Phys. Fluids 8, 1385–1387.CrossRefGoogle Scholar
Lvov, Y. V., Nazarenko, S. & Pokorni, B. 2006 Discreteness and its effect on water-wave turbulence. Physica D 218, 24–35.CrossRefGoogle Scholar
Lvov, Y., Nazarenko, S. & West, R. 2003 Wave turbulence in Bose–Einstein condensates. Physica D 184, 333–351.CrossRefGoogle Scholar
Meyrand, R. & Galtier, S. 2012 Spontaneous chiral symmetry breaking of Hall MHD turbulence. Phys. Rev. Lett. 109, 194501.CrossRefGoogle Scholar
Mininni, P. D. & Pouquet, A. 2010 Rotating helical turbulence. II. Intermittency, scale invariance, and structures. Phys. Fluids 22 (3), 035106.CrossRefGoogle Scholar
Müller, W.-C., Biskamp, D. & Grappin, R. 2003 Statistical anisotropy of magnetohydrodynamic turbulence. Phys. Rev. E 67 (6), 066302.CrossRefGoogle ScholarPubMed
Nazarenko, S. 2011 Wave Turbulence, Lecture Notes in Physics. Springer.CrossRefGoogle Scholar
Nazarenko, S. & Onorato, M. 2007 Freely decaying turbulence and Bose Einstein condensation in Gross Pitaevski model. J. Low Temp. Phys. 146, 31–46.CrossRefGoogle Scholar
Newell, A. C. & Zakharov, V. E. 1992 Rough sea foam. Phys. Rev. Lett. 69, 1149–1151.CrossRefGoogle ScholarPubMed
Ng, C. S. & Bhattacharjee, A. 1996 Interaction of shear-Alfven wavepackets: implication for weak magnetohydrodynamic turbulence in astrophysical plasmas. Astrophys. J. 465, 845–854.CrossRefGoogle Scholar
Saur, J., Politano, H., Pouquet, A. & Matthaeus, W. H. 2002 Evidence for weak MHD turbulence in the middle magnetosphere of Jupiter. Astron. Astrophys. 386, 699–708.CrossRefGoogle Scholar
Scott, J. F. 2014 Wave turbulence in a rotating channel. J. Fluid Mech. 741, 316–349.CrossRefGoogle Scholar
She, Z.-S., Jackson, E. & Orszag, S. A. 1988 Scale-dependent intermittency and coherence in turbulence. J. Sci. Comput. 3, 407–434.CrossRefGoogle Scholar
She, Z.-S. & Leveque, E. 1994 Universal scaling laws in fully developed turbulence. Phys. Rev. Lett. 72, 336–339.CrossRefGoogle ScholarPubMed
Shebalin, J. V., Matthaeus, W. H. & Montgomery, D. 1983 Anisotropy in MHD turbulence due to a mean magnetic field. J. Plasma Phys. 29, 525–547.CrossRefGoogle Scholar
Spyksma, K., Magcalas, M. & Campbell, N. 2012 Quantifying effects of hyperviscosity on isotropic turbulence. Phys. Fluids 24 (12), 125102.CrossRefGoogle Scholar
Sridhar, S. & Goldreich, P. 1994 Toward a theory of interstellar turbulence. 1: weak alfvenic turbulence. Astrophys. J. 432, 612–621.CrossRefGoogle Scholar
Teaca, B., Verma, M. K., Knaepen, B. & Carati, D. 2009 Energy transfer in anisotropic magnetohydrodynamic turbulence. Phys. Rev. E 79 (4), 046312.CrossRefGoogle ScholarPubMed
Zakharov, V. E., L’Vov, V. S. & Falkovich, G. 1992 Kolmogorov Spectra of Turbulence I: Wave Turbulence, Springer Series in Nonlinear Dynamics. Springer.CrossRefGoogle Scholar