Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-11-24T18:30:31.714Z Has data issue: false hasContentIssue false
  • English
  • Français

Weak formulation and scaling properties of energy fluxes in three-dimensional numerical turbulent Rayleigh–Bénard convection

Published online by Cambridge University Press:  19 December 2019

Valentina Valori Open the ORCID record for Valentina Valori [Opens in a new window] ,
Alessio Innocenti ,
Bérengère Dubrulle Open the ORCID record for Bérengère Dubrulle [Opens in a new window]  and
Sergio Chibbaro Open the ORCID record for Sergio Chibbaro [Opens in a new window]
Valentina Valori
Affiliation:
SPEC, CEA, CNRS, Université Paris-Saclay, CEA Saclay, F-91191Gif-sur-Yvette CEDEX, France
Alessio Innocenti
Affiliation:
Sorbonne Université, CNRS, UMR 7190, Institut Jean Le Rond d’Alembert, F-75005Paris, France
Bérengère Dubrulle
Affiliation:
SPEC, CEA, CNRS, Université Paris-Saclay, CEA Saclay, F-91191Gif-sur-Yvette CEDEX, France
Sergio Chibbaro*
Affiliation:
Sorbonne Université, CNRS, UMR 7190, Institut Jean Le Rond d’Alembert, F-75005Paris, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

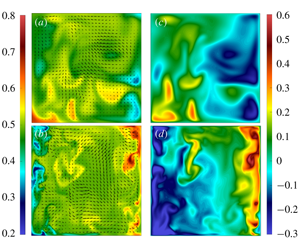
We apply the weak formalism on the Boussinesq equations to characterize scaling properties of the mean and the standard deviation of the potential, kinetic and viscous energy fluxes in very well-resolved numerical simulations. The local Bolgiano–Oboukhov (BO) length is investigated and it is found that its value may change by an order of magnitude through the domain, in agreement with previous results. We then investigate the scale-by-scale averaged terms of the weak equations, which are a generalization of the Kármán–Howarth–Monin and Yaglom equations. We have not found the classical BO picture, but evidence of a mixture of BO and Kolmogorov scalings. In particular, all the energy fluxes are compatible with a BO local Hölder exponent for the temperature and a Kolmogorov 41 for the velocity. This behaviour may be related to anisotropy and to the strong heterogeneity of the convective flow, reflected in the wide distribution of BO local scales. The scale-by-scale analysis allows us also to compare the theoretical BO length computed from its definition with that empirically extracted through scalings obtained from weak analysis. Scalings are observed, but over a limited range. The key result of the work is to show that the analysis of local weak formulation of the problem is powerful to characterize the fluctuation properties.

JFM classification

Turbulent Flows: Turbulent convection Turbulent Flows: Turbulence simulation
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 885 , 25 February 2020 , A14
Copyright
© 2019 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81 (2), 503.CrossRefGoogle Scholar
Ashkenazi, S. & Steinberg, V. 1999 Spectra and statistics of velocity and temperature fluctuations in turbulent convection. Phys. Rev. Lett. 83 (23), 4760.CrossRefGoogle Scholar
Benzi, R., Ciliberto, S., Tripiccione, R., Baudet, C., Massaioli, F. & Succi, S. 1993 Extended self similarity in turbulent flows. Phys. Rev. E 48, R29–R32.Google ScholarPubMed
Benzi, R., Paladin, G., Parisi, G. & Vulpiani, A. 1984 On the multifractal nature of fully developed turbulence and chaotic systems. J. Phys. A: Math. Gen. 17 (18), 3521.CrossRefGoogle Scholar
Benzi, R., Toschi, F. & Tripiccione, R. 1998 On the heat transfer in Rayleigh–Bénard systems. J. Stat. Phys. 93 (3–4), 901–918.CrossRefGoogle Scholar
Benzi, R., Tripiccione, R., Massaioli, F., Succi, S. & Ciliberto, S. 1994 On the scaling of the velocity and temperature structure functions in Rayleigh–Bénard convection. Europhys. Lett. 25 (5), 341.CrossRefGoogle Scholar
Biferale, L. & Procaccia, I. 2005 Anisotropy in turbulent flows and in turbulent transport. Phys. Rep. 414 (2–3), 43–164.CrossRefGoogle Scholar
Boffetta, G., Mazzino, A. & Vulpiani, A. 2008 Twenty-five years of multifractals in fully developed turbulence: a tribute to Giovanni Paladin. J. Phys. A 41 (36), 363001.CrossRefGoogle Scholar
Bolgiano, J. R. 1959 Turbulent spectra in a stably stratified atmosphere. J. Geophys. Res. 64 (12), 2226–2229.CrossRefGoogle Scholar
Calzavarini, E., Toschi, F. & Tripiccione, R. 2002 Evidences of Bolgiano-Obhukhov scaling in three-dimensional Rayleigh–Bénard convection. Phys. Rev. E 66 (1), 016304.Google ScholarPubMed
Camussi, R. & Verzicco, R. 2004 Temporal statistics in high Rayleigh number convective turbulence. Eur. J. Mech. (B/Fluids) 23 (3), 427–442.CrossRefGoogle Scholar
Castaing, B., Gunaratne, G., Heslot, F., Kadanoff, L., Libchaber, A., Thomae, S., Wu, X.-Z., Zaleski, S. & Zanetti, G. 1989 Scaling of hard thermal turbulence in Rayleigh–Bénard convection. J. Fluid Mech. 204, 1–30.CrossRefGoogle Scholar
Castillo-Castellanos, A.2017 Turbulent convection in Rayleigh–Bénard cells with modified boundary conditions. PhD thesis, Université Pierre et Marie Curie.Google Scholar
Castillo-Castellanos, A., Sergent, A., Podvin, B. & Rossi, M. 2019 Cessation and reversals of large-scale structures in square Rayleigh–Bénard cells. J. Fluid Mech. 877, 922–954.CrossRefGoogle Scholar
Castillo-Castellanos, A., Sergent, A. & Rossi, M. 2016 Reversal cycle in square Rayleigh–Bénard cells in turbulent regime. J. Fluid Mech. 808, 614–640.CrossRefGoogle Scholar
Chillà, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh–Bénard convection. Eur. Phys. J. E 35 (7), 58.CrossRefGoogle ScholarPubMed
Ching, E. S. C. 2007 Scaling laws in the central region of confined turbulent thermal convection. Phys. Rev. E 75 (5), 056302.Google ScholarPubMed
Ching, E. S. C., Chui, K. W., Shang, X.-D., Qiu, X. L., Tong, P. & Xia, K.-Q. 2004 Velocity and temperature cross-scaling in turbulent thermal convection. J. Turbul. 5, N27.CrossRefGoogle Scholar
Chorin, A. J. 1969 On the convergence of discrete approximations to the Navier–Stokes equations. Maths Comput. 23 (106), 341–353.CrossRefGoogle Scholar
Cimarelli, A., De Angelis, E. & Casciola, C. M. 2013 Paths of energy in turbulent channel flows. J. Fluid Mech. 715, 436–451.CrossRefGoogle Scholar
Cioni, S., Ciliberto, S. & Sommeria, J. 1995 Temperature structure functions in turbulent convection at low Prandtl number. Europhys. Lett. 32 (5), 413.CrossRefGoogle Scholar
Danaila, L., Anselmet, F., Zhou, T. & Antonia, R. A. 1999 A generalization of Yaglom’s equation which accounts for the large-scale forcing in heated decaying turbulence. J. Fluid Mech. 391, 359–372.CrossRefGoogle Scholar
Dubrulle, B. 2019 Beyond Kolmogorov cascades. J. Fluid Mech. 867, P1.CrossRefGoogle Scholar
Duchon, J. & Robert, R. 2000 Inertial energy dissipation for weak solutions of incompressible Euler and Navier–Stokes equations. Nonlinearity 13 (1), 249.CrossRefGoogle Scholar
Eyink, G. & Sreenivasan, K. 2006 Onsager and the theory of hydrodynamic turbulence. Rev. Mod. Phys. 78 (1), 87.CrossRefGoogle Scholar
Faranda, D., Lembo, V., Iyer, M., Kuzzay, D., Chibbaro, S., Daviaud, F. & Dubrulle, B. 2018 Computation and characterization of local subfilter-scale energy transfers in atmospheric flows. J. Atmos. Sci. 75 (7), 2175–2186.CrossRefGoogle Scholar
Farge, M. 1992 Wavelet transforms and their applications to turbulence. Annu. Rev. Fluid Mech. 24 (1), 395–458.CrossRefGoogle Scholar
Frisch, U. 1995 Turbulence. The legacy of A.N Kolmogorov. Cambridge University Press.CrossRefGoogle Scholar
Fuster, D. 2013 An energy preserving formulation for the simulation of multiphase turbulent flows. J. Comput. Phys. 235, 114–128.CrossRefGoogle Scholar
Gauding, M., Wick, A., Pitsch, H. & Peters, N. 2014 Generalised scale-by-scale energy-budget equations and large-eddy simulations of anisotropic scalar turbulence at various Schmidt numbers. J. Turbul. 15 (12), 857–882.CrossRefGoogle Scholar
Hill, R. J. 1997 Applicability of Kolmogorov’s and Monin’s equations of turbulence. J. Fluid Mech. 353, 67–81.CrossRefGoogle Scholar
van Hooft, A., van Heerwaarden, C., Popinet, S., de Roode, S. & van de Wiel, B. 2017 Adaptive grid refinement for atmospheric boundary layer simulations. In EGU General Assembly Conference Abstracts, vol. 19, p. 7784; Geophysical Research Abstracts. Provided by the SAO/NASA Astrophysics Data System.Google Scholar
Jaffard, S., Meyer, Y. & Ryan, R. D. 2001 Wavelets: Tools for Science and Technology, vol. 69. SIAM.CrossRefGoogle Scholar
Kaczorowski, M. & Xia, K.-Q. 2013 Turbulent flow in the bulk of Rayleigh–Bénard convection: small-scale properties in a cubic cell. J. Fluid Mech. 722, 596–617.CrossRefGoogle Scholar
Kestener, P. & Arneodo, A. 2004 Generalizing the wavelet-based multifractal formalism to random vector fields: application to three-dimensional turbulence velocity and vorticity data. Phys. Rev. Lett. 93 (4), 044501.CrossRefGoogle ScholarPubMed
Kunnen, R. P. J., Clercx, H. J. H., Geurts, B. J., van Bokhoven, L. J. A., Akkermans, R. A. D. & Verzicco, R. 2008 Numerical and experimental investigation of structure-function scaling in turbulent Rayleigh–Bénard convection. Phys. Rev. E 77 (1), 016302.Google ScholarPubMed
Lagrée, P.-Y., Staron, L. & Popinet, S. 2011 The granular column collapse as a continuum: validity of a two-dimensional Navier–Stokes model with a 𝜇 (i)-rheology. J. Fluid Mech. 686, 378–408.CrossRefGoogle Scholar
Liot, O., Seychelles, F., Zonta, F., Chibbaro, S., Coudarchet, T., Gasteuil, Y., Pinton, J.-F., Salort, J. & Chillà, F. 2016 Simultaneous temperature and velocity Lagrangian measurements in turbulent thermal convection. J. Fluid Mech. 794, 655–675.CrossRefGoogle Scholar
Lohse, D. & Xia, K.-Q. 2010 Small-scale properties of turbulent Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 42 (1), 335–364.CrossRefGoogle Scholar
Lvov, V. S. 1991 Spectra of velocity and temperature fluctuations with constant entropy flux of fully developed free-convective turbulence. Phys. Rev. Lett. 67 (6), 687.CrossRefGoogle Scholar
L’vov, V. S. & Falkovich, G. E. 1992 Conservation laws and two-flux spectra of hydrodynamic convective turbulence. Physica D 57 (1–2), 85–95.CrossRefGoogle Scholar
Meneveau, C. 1991 Dual spectra and mixed energy cascade of turbulence in the wavelet representation. Phys. Rev. Lett. 66 (11), 1450.CrossRefGoogle ScholarPubMed
Mollicone, J.-P., Battista, F., Gualtieri, P. & Casciola, C. M. 2018 Turbulence dynamics in separated flows: the generalised Kolmogorov equation for inhomogeneous anisotropic conditions. J. Fluid Mech. 841, 1012–1039.CrossRefGoogle Scholar
Monin, A. S. & Yaglom, A. M. 1975 Statistical Fluid Mechanics. MIT Press.Google Scholar
Oboukhov, A. 1959 Effect of Archimedean forces on the structure of the temperature field in a turbulent flow. Dokl. Akad. Nauk SSSR 125 (6), 1246–1248.Google Scholar
Onsager, L. 1949 Statistical hydrodynamics. Il Nuovo Cimento (1943–1954) 6, 279–287.CrossRefGoogle Scholar
Paladin, G. & Vulpiani, A. 1987 Anomalous scaling laws in multifractal objects. Phys. Rep. 156 (4), 147–225.CrossRefGoogle Scholar
Popinet, S. 2003 Gerris: a tree-based adaptive solver for the incompressible Euler equations in complex geometries. J. Comput. Phys. 190 (2), 572–600.CrossRefGoogle Scholar
Popinet, S. 2009 An accurate adaptive solver for surface-tension-driven interfacial flows. J. Comput. Phys. 228 (16), 5838–5866.CrossRefGoogle Scholar
Rincon, F. 2006 Anisotropy, inhomogeneity and inertial-range scalings in turbulent convection. J. Fluid Mech. 563, 43–69.CrossRefGoogle Scholar
Saw, E.-W., Debue, P., Kuzzay, D., Daviaud, F. & Dubrulle, B. 2018 On the universality of anomalous scaling exponents of structure functions in turbulent flows. J. Fluid Mech. 837, 657–669.CrossRefGoogle Scholar
Saw, E.-W., Kuzzay, D., Faranda, D., Guittonneau, A., Daviaud, F., Wiertel-Gasquet, C., Padilla, V. & Dubrulle, B. 2016 Experimental characterization of extreme events of inertial dissipation in a turbulent swirling flow. Nature Commun. 7, 12466.CrossRefGoogle Scholar
Shang, X.-D., Qiu, X.-L., Tong, P. & Xia, K.-Q. 2003 Measured local heat transport in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 90 (7), 074501.CrossRefGoogle ScholarPubMed
Shishkina, O., Stevens, R. J. A. M., Grossmann, S. & Lohse, D. 2010 Boundary layer structure in turbulent thermal convection and its consequences for the required numerical resolution. New J. Phys. 12 (7), 075022.CrossRefGoogle Scholar
Siggia, E. D. 1994 High Rayleigh number convection. Annu. Rev. Fluid Mech. 26 (1), 137–168.CrossRefGoogle Scholar
Stevens, R. J. A. M., Verzicco, R. & Lohse, D. 2010 Radial boundary layer structure and Nusselt number in Rayleigh–Bénard convection. J. Fluid Mech. 643, 495–507.CrossRefGoogle Scholar
Sun, C., Zhou, Q. & Xia, K.-Q. 2006 Cascades of velocity and temperature fluctuations in buoyancy-driven thermal turbulence. Phys. Rev. Lett. 97 (14), 144504.CrossRefGoogle ScholarPubMed
Togni, R., Cimarelli, A. & De Angelis, E. 2015 Physical and scale-by-scale analysis of Rayleigh–Bénard convection. J. Fluid Mech. 782, 380–404.CrossRefGoogle Scholar
Vallis, G. K. 2017 Atmospheric and Oceanic Fluid Dynamics. Cambridge University Press.CrossRefGoogle Scholar
Verma, M. K., Kumar, A. & Pandey, A. 2017 Phenomenology of buoyancy-driven turbulence: recent results. New J. Phys. 19 (2), 025012.CrossRefGoogle Scholar
Verzicco, R. & Camussi, R. 2003 Numerical experiments on strongly turbulent thermal convection in a slender cylindrical cell. J. Fluid Mech. 477, 19–49.CrossRefGoogle Scholar
Wu, X.-Z., Kadanoff, L., Libchaber, A. & Sano, M. 1990 Frequency power spectrum of temperature fluctuations in free convection. Phys. Rev. Lett. 64 (18), 2140.CrossRefGoogle ScholarPubMed
Zhou, Q., Sun, C. & Xia, K.-Q. 2007 Morphological evolution of thermal plumes in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 98 (7), 074501.CrossRefGoogle ScholarPubMed