Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Lauga, Eric
and
Eloy, Christophe
2013.
Shape of optimal active flagella.
Journal of Fluid Mechanics,
Vol. 730,
Issue. ,
Michelin, Sébastien
Lauga, Eric
and
Bartolo, Denis
2013.
Spontaneous autophoretic motion of isotropic particles.
Physics of Fluids,
Vol. 25,
Issue. 6,
Toppaladoddi, S.
and
Balmforth, N. J.
2014.
Slender axisymmetric Stokesian swimmers.
Journal of Fluid Mechanics,
Vol. 746,
Issue. ,
p.
273.
Ishikawa, Takuji
and
Pedley, T. J.
2014.
Dispersion of model microorganisms swimming in a nonuniform suspension.
Physical Review E,
Vol. 90,
Issue. 3,
Michelin, Sébastien
and
Lauga, Eric
2014.
Phoretic self-propulsion at finite Péclet numbers.
Journal of Fluid Mechanics,
Vol. 747,
Issue. ,
p.
572.
Khair, Aditya S.
and
Chisholm, Nicholas G.
2014.
Expansions at small Reynolds numbers for the locomotion of a spherical squirmer.
Physics of Fluids,
Vol. 26,
Issue. 1,
Ishimoto, Kenta
and
Gaffney, Eamonn A.
2014.
Swimming efficiency of spherical squirmers: Beyond the Lighthill theory.
Physical Review E,
Vol. 90,
Issue. 1,
Izri, Ziane
van der Linden, Marjolein N.
Michelin, Sébastien
and
Dauchot, Olivier
2014.
Self-Propulsion of Pure Water Droplets by Spontaneous Marangoni-Stress-Driven Motion.
Physical Review Letters,
Vol. 113,
Issue. 24,
Delmotte, Blaise
Keaveny, Eric E.
Plouraboué, Franck
and
Climent, Eric
2015.
Large-scale simulation of steady and time-dependent active suspensions with the force-coupling method.
Journal of Computational Physics,
Vol. 302,
Issue. ,
p.
524.
Pedley, T. J.
2016.
Spherical squirmers: models for swimming micro-organisms.
IMA Journal of Applied Mathematics,
Vol. 81,
Issue. 3,
p.
488.
Ishikawa, Takuji
Kajiki, Shunsuke
Imai, Yohsuke
and
Omori, Toshihiro
2016.
Nutrient uptake in a suspension of squirmers.
Journal of Fluid Mechanics,
Vol. 789,
Issue. ,
p.
481.
Ishimoto, Kenta
2017.
Guidance of microswimmers by wall and flow: Thigmotaxis and rheotaxis of unsteady squirmers in two and three dimensions.
Physical Review E,
Vol. 96,
Issue. 4,
Fernandez, Vicente I.
and
Stocker, Roman
2017.
Modus vivendi.
Nature Physics,
Vol. 13,
Issue. 4,
p.
326.
Shaik, Vaseem A.
and
Ardekani, Arezoo M.
2017.
Motion of a model swimmer near a weakly deforming interface.
Journal of Fluid Mechanics,
Vol. 824,
Issue. ,
p.
42.
Mueller, Peter
and
Thiffeault, Jean-Luc
2017.
Fluid transport and mixing by an unsteady microswimmer.
Physical Review Fluids,
Vol. 2,
Issue. 1,
Nganguia, Herve
Pietrzyk, Kyle
and
Pak, On Shun
2017.
Swimming efficiency in a shear-thinning fluid.
Physical Review E,
Vol. 96,
Issue. 6,
Morozov, Matvey
and
Michelin, Sébastien
2019.
Nonlinear dynamics of a chemically-active drop: From steady to chaotic self-propulsion.
The Journal of Chemical Physics,
Vol. 150,
Issue. 4,
Morozov, Matvey
and
Michelin, Sébastien
2019.
Orientational instability and spontaneous rotation of active nematic droplets.
Soft Matter,
Vol. 15,
Issue. 39,
p.
7814.
Ishikawa, Takuji
2019.
Stability of a Dumbbell Micro-Swimmer.
Micromachines,
Vol. 10,
Issue. 1,
p.
33.
Mandre, Shreyas
2020.
Work-minimizing kinematics for small displacement of an infinitely long cylinder.
Journal of Fluid Mechanics,
Vol. 893,
Issue. ,
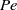 $\mathit{Pe}$) on the advection–diffusion problem for the nutrients. We show that the mean rate of feeding as well as its fluctuations in time depend only on the swimming modes of the squirmer up to order
$\mathit{Pe}$) on the advection–diffusion problem for the nutrients. We show that the mean rate of feeding as well as its fluctuations in time depend only on the swimming modes of the squirmer up to order 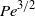 ${\mathit{Pe}}^{3/ 2} $, even when no swimming occurs on average, while the influence of non-swimming modes comes in only at order
${\mathit{Pe}}^{3/ 2} $, even when no swimming occurs on average, while the influence of non-swimming modes comes in only at order  ${\mathit{Pe}}^{2} $. We also show that generically we expect a phase delay between feeding and swimming of
${\mathit{Pe}}^{2} $. We also show that generically we expect a phase delay between feeding and swimming of 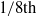 $1/ 8\mathrm{th} $ of a period. Numerical computations for illustrative strokes at finite
$1/ 8\mathrm{th} $ of a period. Numerical computations for illustrative strokes at finite 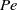 $\mathit{Pe}$ confirm quantitatively our analytical results linking swimming and feeding. We finally derive, and use, an adjoint-based optimization algorithm to determine the optimal unsteady strokes maximizing feeding rate for a fixed energy budget. The overall optimal feeder is always the optimal steady swimmer. Within the set of time-periodic strokes, the optimal feeding strokes are found to be equivalent to those optimizing periodic swimming for all values of the Péclet number, and correspond to a regularization of the overall steady optimal.
$\mathit{Pe}$ confirm quantitatively our analytical results linking swimming and feeding. We finally derive, and use, an adjoint-based optimization algorithm to determine the optimal unsteady strokes maximizing feeding rate for a fixed energy budget. The overall optimal feeder is always the optimal steady swimmer. Within the set of time-periodic strokes, the optimal feeding strokes are found to be equivalent to those optimizing periodic swimming for all values of the Péclet number, and correspond to a regularization of the overall steady optimal.