Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-24T16:34:46.114Z Has data issue: false hasContentIssue false
  • English
  • Français

Turbulent three-dimensional dielectric electrohydrodynamic convection between two plates

Published online by Cambridge University Press:  24 February 2012

A. Kourmatzis  and
J. S. Shrimpton
A. Kourmatzis
Affiliation:
Clean Combustion Group, Aerospace, Mechanical and Mechatronic Engineering, University of Sydney, Sydney, NSW 2006, Australia
J. S. Shrimpton*
Affiliation:
Energy Technology Research Group, School of Engineering Sciences, University of Southampton, Southampton SO17 1BJ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The fundamental mechanisms responsible for the creation of electrohydrodynamically driven roll structures in free electroconvection between two plates are analysed with reference to traditional Rayleigh–Bénard convection (RBC). Previously available knowledge limited to two dimensions is extended to three-dimensions, and a wide range of electric Reynolds numbers is analysed, extending into a fully inherently three-dimensional turbulent regime. Results reveal that structures appearing in three-dimensional electrohydrodynamics (EHD) are similar to those observed for RBC, and while two-dimensional EHD results bear some similarities with the three-dimensional results there are distinct differences. 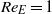Analysis of two-point correlations and integral length scales show that full three-dimensional electroconvection is more chaotic than in two dimensions and this is also noted by qualitatively observing the roll structures that arise for both low () and high electric Reynolds numbers (up to 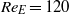). Furthermore, calculations of mean profiles and second-order moments along with energy budgets and spectra have examined the validity of neglecting the fluctuating electric field 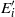in the Reynolds-averaged EHD equations and provide insight into the generation and transport mechanisms of turbulent EHD. Spectral and spatial data clearly indicate how fluctuating energy is transferred from electrical to hydrodynamic forms, on moving through the domain away from the charging electrode. It is shown that is not negligible close to the walls and terms acting as sources and sinks in the turbulent kinetic energy, turbulent scalar flux and turbulent scalar variance equations are examined. Profiles of hydrodynamic terms in the budgets resemble those in the literature for RBC; however there are terms specific to EHD that are significant, indicating that the transfer of energy in EHD is also attributed to further electrodynamic terms and a strong coupling exists between the charge flux and variance, due to the ionic drift term.

JFM classification

Convection: Buoyancy-driven instability Complex Fluids: Dielectrics Drops and Bubbles: Electrohydrodynamic effects
Type
Papers
Information
Journal of Fluid Mechanics , Volume 696 , 10 April 2012 , pp. 228 - 262
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Atten, P. 1996 Electrohydrodynamic instability and motion induced by injected space charge in insulating liquids. IEEE Trans. Dielec. Elec. Insul. 3, 1–17.CrossRefGoogle Scholar
2. Atten, P. 1998 Instability and convection induced by injected space charge. In Electrohydrodynamics, 1st edn (ed. Castellanos, A ). Springer.Google Scholar
3. Bailey, A. G. 1986 The theory and practice of electrostatic spraying. Atomiz. Spray Technol. 2, 95–134.Google Scholar
4. Balachandran, W., Machowski, W., Halimic, M., Morgan, L., Gray, C. & Wilson, C. 1999 Development of an electrostatic nozzle for gas turbine applications. In Proceedings of ILASS, Toulouse, France. ILASS.Google Scholar
5. Bankston, C. P., Back, L. H., Kwack, E. Y. & Kelly, A. J. 1988 Experimental investigation of electrostatic dispersion and combustion of diesel fuel jets. Trans. ASME: J. Engng Gas Turbines Power 110, 361–368.Google Scholar
6. Batchelor, G. K. 1959 Small-scale variation of convected quantities like temperature in turbulent fluid. J. Fluid Mech. 5, 113–133.Google Scholar
7. Castellanos, A. 1991 Coulomb-driven convection in electrohydrodynamics. IEEE Trans. Elec. Insul. 26, 1201–1215.Google Scholar
8. Castellanos, A. 1998 Basic concepts and equations in electrohydrodynamics. In Electrohydrodynamics, 1st edn (ed. Castellanos, A. ). Springer.Google Scholar
9. Castro, I. P. 1989 An Introduction to the Digital Analysis of Stationary Signals. IOP.Google Scholar
10. Chandra, L. & Grotzbach, G. 2008 Analysis and modelling of the turbulent diffusion of turbulent heat fluxes in natural convection. Intl J. Heat Fluid Flow 29, 743–751.Google Scholar
11. Chavanne, M. X., Chilla, F., Chabaud, B., Castaing, B. & Hebral, B. 2001 Turbulent Rayleigh Benard convection in gaseous and liquid He. Phys. Fluids 13, 1300–1320.CrossRefGoogle Scholar
12. Chicon, R., Castellanos, A. & Martin, E. 1997 Numerical modelling of Coulomb-driven convection in insulating liquids. J. Fluid Mech. 344, 46–66.Google Scholar
13. Crowley, J. M. 1999 Fundamentals of Applied Electrostatics. Laplacian.Google Scholar
14. Davidson, J. H. & McKinney, P. J. 1989 Turbulent mixing in a barbed plate-to-plate electrostatic precipitator. Atmos. Environ. 23, 2093–2107.CrossRefGoogle Scholar
15. Davidson, J. H. & McKinney, P. J. 1991 EHD flow visualization in the wire-plate and barbed plate electrostatic precipitator. IEEE Trans. Ind. Applics. 27, 154–160.Google Scholar
16. Eckhardt, B., Grossman, S. & Lohse, D. 2007 Fluxes and energy dissipation in thermal convection and shear flows. Europhys. Lett. 78 (24001), 1–6.Google Scholar
17. Getling, A. V. 1998 Rayleigh Benard Convection, Structures and Dynamics. World Scientific.Google Scholar
18. Hong, L., Wang, L.-Z. & Wu, Z.-N. 2008 EHD turbulent flow and monte-carlo simulation for particle charging and tracing in a wire-plate electrostatic precipitator. J. Electrostat. 66, 130–141.Google Scholar
19. Hopfinger, E. J. & Gosse, J. P. 1971 Charge transport by self-generated turbulence in insulating liquids submitted to unipolar injection. Phys. Fluids 14, 1671–1682.Google Scholar
20. Kelly, A. J. 1984 The electrostatic atomization of hydrocarbons. J. Inst. Energy 57, 312–320.Google Scholar
21. Kelly, A. J. 1990 Charge injection electrostatic atomizer modelling. Aerosol Sci. Technol. 12, 526–537.Google Scholar
22. Kerr, R. M. 2001 Energy budget in Rayleigh-Benard convection. Phys. Rev. Lett. 87 (244502), 1–4.Google Scholar
23. Kim, K. & Turnbull, J. 1976 Generation of charged drops of insulating liquids by electrostatic spraying. J. Appl. Phys. 47, 1964–1969.Google Scholar
24. Kourmatzis, A. & Shrimpton, J. 2009 Electrohydrodynamics and charge injection atomizers: a review of the governing equations and turbulence. Atomiz. Sprays 19, 1045–1063.CrossRefGoogle Scholar
25. Kourmatzis, A. & Shrimpton, J. S. 2012 Simulation of dielectric liquid electrohydrodynamic (EHD) convection with a conventional finite volume scheme, Intl J. Numer. Meth. Fluids (submitted).Google Scholar
26. Lacroix, J. C., Atten, P. & Hopfinger, E. J. 1975 Electro-convection in a dielectric liquid layer subjected to unipolar injection. J. Fluid Mech. 69, 539–564.CrossRefGoogle Scholar
27. Lehr, W. & Hiller, W. 1993 Electrostatic atomization of liquid hydrocarbons. J. Electrostat. 30, 433–440.CrossRefGoogle Scholar
28. Melcher, R. J. 1981 Continuum Electromechanics. MIT.Google Scholar
29. Perez, A. T., Traore, P. H., Koulova-Nenova, D. & Romat, H. 2009 Numerical study of an electrohydrodynamic plume between a blade injector and a flat plate. IEEE Trans. Dielec. Elec. Insul. 16, 448–455.Google Scholar
30. Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.CrossRefGoogle Scholar
31. Rigit, A. R. H & Shrimpton, J. S. 2006 Spray characteristics of charge injection electrostatic atomizers with small-orifice diameters. Atomiz. Sprays 16, 421–442.Google Scholar
32. Schneider, J. M. & Watson, P. K. 1970 Electrohydrodynamic stability of space-charge-limited currents in dielectric liquids. Part I. Theoretical study. Phys. Fluids 13, 1948–1954.Google Scholar
33. Shishkina, O. & Wagner, C. 2006 Analysis of thermal dissipation rates in turbulent Rayleigh Benard convection. J. Fluid Mech. 546, 51–60.Google Scholar
34. Shrimpton, J. & Kourmatzis, A. 2010 Direct numerical simulation of forced flow dielectric EHD within charge injection atomizers. IEEE Trans. Dielec. Elec. Insul. 17, 1838–1845.Google Scholar
35. Shrimpton, J. S. & Yule, A. J. 2003 Electrohydrodynamics of charge injection atomization: Regimes and fundamental limits. Atomiz. Sprays 12, 173–190.Google Scholar
36. Soldati, A. & Banerjee, S. 1998 Turbulence modification by large-scale organized electrohydrodynamic flows. Phys. Fluids 10, 1742–1756.Google Scholar
37. Tobazeon, R. 1984 Electrohydrodynamic instabilities and electroconvection in the transient and A.C. regime of unipolar injection in insulating liquids: A review. J. Electrostat. 15, 359–384.Google Scholar
38. Traore, P. H., Perez, A. T., Koulova, D. & Romat, H. 2010 Numerical modelling of finite-amplitude electro-thermo-convection in a dielectric liquid layer subjected to both unipolar injection and temperature gradient. J. Fluid Mech. 658, 279–293.Google Scholar
39. Vazquez, P. A., Gheorgiou, G. E. & Castellanos, A. 2006 Characterization of injection instabilities in electrohydrodynamics by numerical modelling: comparison of particle in cell and flux corrected transport methods for electroconvection between two plates. J. Phys. D: Appl. Phys. 39, 2754–2763.Google Scholar
40. Vazquez, P. A., Gheorgiou, G. E. & Castellanos, A. 2008 Numerical analysis of the stability of the electrohydrodynamic (EHD) electroconvection between two plates. J. Phys. D: Appl. Phys. 41, 10pp.CrossRefGoogle Scholar
41. Vazquez, P. A., Perez, A. T. & Castellanos, A. 1996 Thermal and electrohydrodynamic plumes. a comparative study. Phys. Fluids 8, 2091–2096.CrossRefGoogle Scholar
42. Versteeg, H. K. & Malalasekera, W. 1995 An Introduction to Computational Fluid Dynamics. Longman.Google Scholar
43. Verzicco, R. & Sreenivasan, K. R. 2008 A comparison of turbulent thermal convection between conditions of constant temperature and constant heat flux. J. Fluid Mech. 595, 203–219.Google Scholar
44. Worner, M. & Grotzbach, G. 1998 Pressure transport in direct numerical simulations of turbulent natural convection in horizontal fluid layers. Intl J. Heat Fluid Flow 19, 150–158.Google Scholar
45. Wu, X.-Z., Kadanoff, L., Libchaber, A. & Sano, M. 1990 Frequency power spectrum of temperature fluctuations in free convection. Phys. Rev. Lett. 64, 2140–2143.Google Scholar
46. Xi, H.-W. & Gunton, J. D. 1993 Spiral-pattern formation in Rayleigh-Benard convection. Phys. Rev. 47, R2987–R2990.Google ScholarPubMed
47. Yamamoto, T., Morita, Y., Fujishuma, H. & Okubo, M. 2006 Three-dimensional EHD simulation for point corona electrostatic precipitator based on laminar and turbulent models. J. Electrostat. 64, 628–633.Google Scholar
48. Ye, Q., Domnick, A. S. & Pulli, K. 2005 Numerical simulation of electrostatic spray-painting processes in the automotive industry. High Performance Comput. Sci. Engng 4, 261–275.Google Scholar
49. Yeung, P. K., Xu, S. & Sreenivasan, K. R. 2002 Schmidt number effects on turbulent transport with uniform mean scalar gradient. Phys. Fluids 14, 4178–4191.Google Scholar