Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-28T08:58:03.273Z Has data issue: false hasContentIssue false
  • English
  • Français

Turbulent structure of high-amplitude pressure peaks within the turbulent boundary layer

Published online by Cambridge University Press:  24 October 2013

S. Ghaemi  and
F. Scarano
S. Ghaemi*
Affiliation:
Department of Aerodynamics, Delft University of Technology, Kluyverweg 1, 2629 HS, Delft, The Netherlands
F. Scarano
Affiliation:
Department of Aerodynamics, Delft University of Technology, Kluyverweg 1, 2629 HS, Delft, The Netherlands
*
†Present address: Department of Mechanical Engineering, University of Alberta, Edmonton, Alberta, T6G 2G8, Canada. Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The positive and negative high-amplitude pressure peaks (HAPP) are investigated in a turbulent boundary layer at 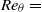$R{e}_{\theta } = $ 1900 in order to identify their turbulent structure. The three-dimensional velocity field is measured within the inner layer of the turbulent boundary layer using tomographic particle image velocimetry (tomo-PIV). The measurements are performed at an acquisition frequency of 10 000 Hz and over a volume of 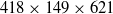$418\times 149\times 621$ wall units in the streamwise, wall-normal and spanwise directions, respectively. The time-resolved velocity fields are applied to obtain the material derivative using the Lagrangian method followed by integration of the Poisson pressure equation to obtain the three-dimensional unsteady pressure field. The simultaneous volumetric velocity, acceleration, and pressure data are conditionally sampled based on local maxima and minima of wall pressure to analyse the three-dimensional turbulent structure of the HAPPs. Analysis has associated the positive HAPPs to the shear layer structures formed by an upstream sweep of high-speed flow opposing a downstream ejection event. The sweep event is initiated in the outer layer while the ejection of near-wall fluid is formed by the hairpin category of vortices. The shear layers were observed to be asymmetric in the instantaneous visualizations of the velocity and acceleration fields. The asymmetric pattern originates from the spanwise component of temporal acceleration of the ejection event downstream of the shear layer. The analysis also demonstrated a significant contribution of the pressure transport term to the budget of the turbulent kinetic energy in the shear layers. Investigation of the conditional averages and the orientation of the vortices showed that the negative HAPPs are linked to both the spanwise and quasi-streamwise vortices of the turbulent boundary layer. The quasi-streamwise vortices can be associated with the hairpin category of vortices or the isolated quasi-streamwise vortices of the inner layer. A bi-directional analysis of the link between the HAPPs and the hairpin paradigm is also conducted by conditionally averaging the pressure field based on the detection of hairpin vortices using strong ejection events. The results demonstrated positive pressure in the shear layer region of the hairpin model and negative pressure overlapping with the vortex core.

JFM classification

Boundary Layers: Boundary layer structure Turbulent Flows: Turbulent boundary layers
Type
Papers
Information
Journal of Fluid Mechanics , Volume 735 , 25 November 2013 , pp. 381 - 426
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Acalar, M. S. & Smith, C. R. 1987 A study of hairpin vortices in a laminar boundary layer. Part 2. Hairpin vortices generated by fluid injection. J. Fluid Mech. 175, 43–83.Google Scholar
Adrian, R. J. 2007 Hairpin vortex organization in wall turbulence. Phys. Fluids 19, 041301.Google Scholar
Adrian, R. J., Meinhart, C. D. & Tomkins, C. D. 2000 Vortex organization in the outer region of the turbulent boundary layer. J. Fluid Mech. 422, 1–54.Google Scholar
Alfredsson, P. H., Johansson, A. V., Haritonidis, J. H. & Eckelmann, H. 1988a The fluctuating wall-shear stress and the velocity field in the viscous sublayer. Phys. Fluids 31, 1026–1033.Google Scholar
Alfredsson, P. H., Johansson, A. V. & Kim, J. 1988b Turbulence production near walls: the role of flow structures with spanwise symmetry. In Proceeding of the Summer Program, pp. 131–141, Center for Turbulence research, Stanford, California.Google Scholar
Andreopoulos, J. & Agui, J. H. 1996 Wall-vorticity flux dynamics in two-dimensional turbulent boundary layer. J. Fluid Mech. 309, 45–84.Google Scholar
Antonia, R. A. 1981 Conditional sampling in turbulence measurement. Annu. Rev. Fluid Mech. 13, 131–156.Google Scholar
Baur, T. & Köngeter, J. 1999 PIV with high temporal resolution for the determination of local pressure reductions from coherent turbulent phenomena. In Proceedings of the 3rd International Workshop on Particle Image Velocimetry, Santa Barbara, USA.Google Scholar
Blackwelder, R. F. & Kaplan, R. E. 1976 On the wall structure of the turbulent boundary layer. J. Fluid Mech. 76, 89.Google Scholar
Blake, W. K. 1970 Turbulent boundary-layer wall-pressure fluctuations on smooth and rough walls. J. Fluid Mech. 44, 637.Google Scholar
Boillot, W. K. & Prasad, A. K. 1996 Optimization procedure for pulse separation in cross-correlation PIV. Exp. Fluids 21, 87–93.Google Scholar
Bradshaw, P. ‘Inactive’ motion and pressure fluctuations in turbulent boundary layers. J. Fluid Mech. 30, 241.Google Scholar
Brouwer, H. H. & Rienstra, S. W. 2008 Aeroacoustics research in Europe: the CEAS–ASC report on 2007 highlights. J. Sound Vib. 318, 625–654.CrossRefGoogle Scholar
Bull, M. K. 1967 Wall pressure fluctuations associated with subsonic turbulent boundary layer flow. J. Fluid Mech. 28, 719.CrossRefGoogle Scholar
Chang, P. A. III, Piomelli, U. & Blake, W. K. 1999 Relationship between wall pressure and velocity-field sources. Phys. Fluids 11, 3434–3448.Google Scholar
Charonko, J. J., King, C. V., Smith, B. L. & Vlachos, P. P. 2010 Assessment of pressure field calculations from particle image velocimetry measurements. Meas. Sci. Technol. 21, 105401.CrossRefGoogle Scholar
Chew, Y. T., Khoo, B. C. & Li, G. L. 1994 A time-resolved hot-wire shear stress probe for turbulent flow: use of laminar flow calibration. Exp. Fluids 17, 75–83.Google Scholar
Choi, K. S. 1989 Near-wall structure of a turbulent boundary layer with riblets. J. Fluid Mech. 208, 417.Google Scholar
Clinch, J. M. 1969 Measurement of the wall pressure field at the surface of a smooth-wall pipe containing turbulent water flow. J. Sound Vib. 9, 398.Google Scholar
Corcos, G. M. 1963 Resolution of pressure in turbulence. J. Acoust. Soc. Am. 35, 192–199.Google Scholar
Davidson, P. A. 2004 Turbulence: An Introduction for Scientists and Engineers. Oxford University Press.Google Scholar
De Kat, R. & van Oudheusden, B. W. 2012 Instantaneous planar pressure determination from PIV in turbulent flow. Exp. Fluids 52, 1089–1106.Google Scholar
De Ojeda, W. & Wark, C. E. 1997 Instantaneous velocity and wall pressure features in a turbulent boundary layer. Final Rep. ONR N00014-93-1-0639.CrossRefGoogle Scholar
Eggels, J. G. M., Unger, F., Weiss, M. H., Westerweel, J., Adrian, R. J., Friedrich, R. & Nieuwstadt, F. T. M. 1994 Fully developed turbulent pipe flow: a comparison between direct numerical simulation and experiment. J. Fluid Mech. 268, 175–209.Google Scholar
Elsinga, G. E. 2008 Tomographic particle image velocimetry and its application to turbulent boundary layer, PhD thesis, Delft University of Technology.Google Scholar
Farabee, T. M. & Casarella, M. J. 1991 Spectral features of wall pressure fluctuations beneath turbulent boundary layers. Phys. Fluids 3, 2410.CrossRefGoogle Scholar
Ffowcs Williams, J. E. & Hall, L. H. 1970 Aerodynamic sound generation by turbulent flow in the vicinity of a scattering half plane. J. Fluid Mech. 40, 657.Google Scholar
Ganapathisubramani, B., Hutchins, N., Monty, J. P., Chung, D. & Marusic, I. 2012 Amplitude and frequency modulation in wall turbulence. J. Fluid Mech. 712, 61–91.Google Scholar
Ganapathisubramani, B., Longmire, E. K. & Marusic, I. 2006 Experimental investigation of vortex properties in a turbulent boundary layer. Phys. Fluids 18, 055105.Google Scholar
Ghaemi, S., Ragni, D. & Scarano, F. 2012 PIV-based pressure fluctuations in the turbulent boundary layer. Exp. Fluids 53 (6), 1823–1840.Google Scholar
Ghaemi, S. & Scarano, F. 2010 Multi-pass light amplification for tomographic particle image velocimetry applications. Meas. Sci. Technol. 21 (12), 127002.Google Scholar
Ghaemi, S. & Scarano, F. 2011 Counter-hairpin vortices in the turbulent wake of a sharp trailing-edge. J. Fluid Mech. 689, 317–356.Google Scholar
Gravante, S. P., Naguib, A. M., Wark, C. E. & Nagib, H. M. 1998 Characterization of the pressure fluctuations under a fully developed turbulent boundary layer. AIAA J. 36 (10), 1808–1816.CrossRefGoogle Scholar
Guezennec, Y. G., Piomelli, U. & Kim, J. 1989 On the shape and dynamics of wall structures in turbulent channel flow. Phys. Fluids A 1 (4), 764–766.CrossRefGoogle Scholar
Gurka, R., Liberzon, A., Hefetz, D., Rubinstein, D. & Shavit, U. 1999 Computation of pressure distribution using PIV velocity data. In Proceedings of the 3rd International Workshop on Particle Image Velocimetry, Santa Barbara, USA.Google Scholar
Haritonidis, J. H., Gresko, L. S. & Breuer, K. S. 1990 Wall pressure peaks and waves. In Near-Wall Turbulence, Proceedings of the 1988 Zoran Zaric Memorial Conference (ed. Kline, S. J. & Afgan, N. H.), pp. 397–417. Hemisphere.Google Scholar
Head, M. R. & Bandyopadhyay, P. 1981 New aspects of turbulent boundary-layer structure. J. Fluid Mech. 107, 297–338.CrossRefGoogle Scholar
Herman, G. T. & Lent, A. 1976 Iterative reconstruction algorithms. Comput. Biol. Med. 6, 273–294.Google Scholar
Howe, M. S. 1991 Surface pressures and sound produced by turbulent flow over smooth and rough walls. J. Acoust. Soc. Am. 90, 1041.Google Scholar
Hunt, J. C. R., Wray, A. A. & Moin, P. 1988 Eddies, stream, and convergence zones in turbulent flows. Research Rep. CTR-S88, pp. 193–208. Center for Turbulence Research, Stanford.Google Scholar
Hussain, A. K. M. F. & Reynolds, W. C. 1975 Measurements in fully developed turbulent channel flow. J. Fluid Mech. 97, 568–578.Google Scholar
Hutchins, N., Monty, J. P., Ganapathisubramani, B., Ng, H. C. H. & Marusic, I. 2011 Three-dimensional conditional structure of a high-Reynolds-number turbulent boundary layer. J. Fluid Mech. 673, 255–285.Google Scholar
Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.Google Scholar
Johansson, A. V. & Alfredsson, P. H. 1982 On the structure of turbulent channel flow. J. Fluid Mech. 122, 295–314.Google Scholar
Johansson, A. V., Alfredsson, P. H. & Kim, J. 1991 Evolution and dynamics of shear-layer structures in near-wall turbulence. J. Fluid Mech. 224, 579–599.CrossRefGoogle Scholar
Johansson, A. V., Her, J. Y. & Haritonidis, J. H. 1987 On the generation of high-amplitude wall-pressure peaks in turbulent boundary layers and spots. J. Fluid Mech. 175, 119.Google Scholar
Karangelen, C. C., Wilczynski, V. & Casarella, M. J. 1993 Large amplitude wall pressure events beneath a turbulent boundary layer. Trans. ASME: J. Fluids Engng 115, 653–659.Google Scholar
Kim, H. T., Kline, S. J. & Reynolds, W. C. 1971 The production of turbulence near smooth wall in a turbulent boundary layer. J. Fluid Mech. 50, 133–160.Google Scholar
Kim, J. 1983 On the structure of wall-bounded turbulent flows. Phys. Fluids 26 (8), 2088–2097.CrossRefGoogle Scholar
Kim, J. 1989 On the structure of pressure fluctuations in simulated turbulent channel flow. J. Fluid. Mech. 205, 421.Google Scholar
Kim, J., Choi, J. & Sung, H. J. 2002 Relationship between wall-pressure fluctuations and streamwise vortices in a turbulent boundary layer. Phys. Fluids 14 (2), 898–901.Google Scholar
Kim, J., Moin, P. & Moser, R. 1987 Turbulence statistics in fully developed channel flow at low Reynolds number. J. Fluid Mech. 177, 133–166.Google Scholar
Klewicki, J. C. & Hirschi, C. R. 2004 Flow field properties local to near-wall shear layers in a low Reynolds number turbulent boundary layer. Phys. Fluids 16, 4163–4176.Google Scholar
Kobashi, Y. & Ichijo, M. 1986 Wall pressure and its relation to turbulent structure of a boundary layer. Exp. Fluids 4, 49.CrossRefGoogle Scholar
Koschatzky, V., Moore, P. D., Westerweel, J., Scarano, F. & Boersma, B. J. 2011 High speed PIV applied to aerodynamic noise investigation. Exp. Fluids 50, 863–876.Google Scholar
Krogstad, P.-Å. & Antonia, R. A. 1994 Structure of turbulent boundary layers in smooth and rough walls. J. Fluid Mech. 277, 1–21.CrossRefGoogle Scholar
Liu, X. & Katz, J. 2006 Instantaneous pressure and material acceleration measurements using a four-exposure PIV system. Exp. Fluids 41, 227–240.CrossRefGoogle Scholar
Liu, Z. C., Landreth, C. C., Adrian, R. J. & Hanratty, T. J. 1991 High resolution measurement of turbulent structure in a channel with particle image velocimetry. Exp. Fluids 10, 301–312.Google Scholar
Lueptow, R. M. 1995 Transducer resolution and the turbulent wall pressure spectrum. J. Acoust. Soc. Am. 97, 370–378.Google Scholar
Meinhart, C. D., Wereley, S. T. & Santiago, J. G. 2000 A PIV algorithm for estimating time-averaged velocity fields. Trans. ASME: J. Fluids Engng 122, 285–289.Google Scholar
Na, Y. & Moin, P. 1998 The structure of wall-pressure fluctuations in turbulent boundary layers with adverse pressure gradient and separation. J. Fluid Mech. 377, 347–373.Google Scholar
Naguib, A. M., Wark, C. E. & Juckenhöfel, O. 2001 Stochastic estimation and flow sources associated with surface pressure events in a turbulent boundary layer. Phys. Fluids 13 (9), 2611–2626.Google Scholar
Novara, M., Ianiro, A. & Scarano, F. 2013 Adaptive interrogation for 3D-dimensional-PIV. Meas. Sci. Technol. 24, 024012.Google Scholar
Perry, A. E, Henbest, S. & Chong, M. S. 1986 A theoretical and experimental study of wall turbulence. J. Fluid Mech. 165, 163–199.Google Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.Google Scholar
Rathnasingham, R. & Breuer, K. S. 2003 Active control of turbulent boundary layers. J. Fluid Mech. 495, 209.Google Scholar
Robinson, S. K. 1990 A perspective on coherent structures and conceptual models for turbulent boundary layer physics. AIAA Paper 90-1638.Google Scholar
Robinson, S. K. 1991 Coherent motions in the turbulent boundary layer. Annu. Rev. Fluid Mech. 23, 601–636.Google Scholar
Scarano, F. & Poelma, C. 2009 Three-dimensional vorticity patterns of cylinder wakes. Exp. Fluids 47, 69–83.Google Scholar
Schewe, G. 1983 On the structure and resolution of wall-pressure fluctuations associated with turbulent boundary-layer flow. J. Fluid Mech. 134, 311.Google Scholar
Schröder, A., Geisler, R., Elsinga, G. E., Scarano, F. & Dierksheide, U. 2008 Investigation of a turbulent spot and tripped turbulent boundary layer flow using time-resolved tomographic PIV. Exp. Fluids 44, 305–316.Google Scholar
Shaw, R. 1960 Influence of hole dimensions on static pressure measurements. J. Fluid Mech. 7, 550–564.Google Scholar
Sheng, J., Malkiel, E. & Katz, J. 2009 Buffer layer structures associated with extreme wall stress events in a smooth wall turbulent boundary layer. J. Fluid Mech. 633, 17–60.Google Scholar
Smith, C. R. & Metzler, S. P. 1983 The characteristics of low-speed streaks in the near-wall region of a turbulent boundary layer. J. Fluid Mech. 129, 27–54.Google Scholar
Spalart, P. R. 1988 Direct simulation of a turbulent boundary layer up to ${\mathit{Re}}_{\theta } = 1400$ . J. Fluid Mech. 187, 61–89.Google Scholar
Stanislas, M., Perret, L. & Foucaut, J. M. 2008 Vortical structures in the turbulent boundary layer: a possible route to a universal representation. J. Fluid Mech. 602, 327.Google Scholar
Theunissen, R., Scarano, F. & Reithmuller, M. L. 2008 On improvement of PIV interrogation near stationary interfaces. Exp. Fluids 45, 557–572.Google Scholar
Thomas, A. S. W. & Bull, M. K. 1983 On the role of wall-pressure fluctuations in deterministic motions in the turbulent boundary layer. J. Fluid Mech. 128, 283.Google Scholar
Townsend, A. 1976 The Structure of Turbulent Shear Flow, 2nd edn. Cambridge University Press.Google Scholar
Tsuji, Y., Fransson, J. H. M., Alfredsson, P. H. & Johansson, A. V. 2007 Pressure statistics and their scaling in high-Reynolds-number turbulent boundary layers. J. Fluid Mech. 585, 1–40.Google Scholar
Vedula, P. & Yeung, P. K. 1999 Similarity scaling of acceleration and pressure statistics in numerical simulations of isotropic turbulence. Phys. Fluids 11, 1208–1220.CrossRefGoogle Scholar
Violato, D., Moore, P. & Scarano, F. 2011 Lagrangian and Eulerian pressure field evaluation of rod-aerofoil flow from time-resolved tomographic PIV. Exp. Fluids 50, 1057–1070.Google Scholar
Westerweel, J. 1997 Fundamentals of digital particle image velocimetry. Meas. Sci. Technol. 8, 1379–1392.Google Scholar
Wieneke, B. 2008 Volume self-calibration for three-dimensional particle image velocimetry. Exp. Fluids 45, 549–556.Google Scholar
Willmarth, W. W. 1975 Pressure fluctuations beneath turbulent boundary layers. Annu. Rev. Fluid Mech. 7, 13.Google Scholar
Willmarth, W. W. & Wooldridge, C. E. 1962 Measurements of the fluctuating pressure at the wall beneath a thick turbulent boundary layer. J. Fluid Mech. 14, 187.Google Scholar