Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Zürner, Till
2020.
Refined mean field model of heat and momentum transfer in magnetoconvection.
Physics of Fluids,
Vol. 32,
Issue. 10,
Madonia, Matteo
Aguirre Guzmán, Andrés J.
Clercx, Herman J. H.
and
Kunnen, Rudie P. J.
2021.
Velocimetry in rapidly rotating convection: Spatial correlations, flow structures and length scales
(a)
.
EPL (Europhysics Letters),
Vol. 135,
Issue. 5,
p.
54002.
Leng, X.-Y.
Krasnov, D.
Li, B.-W.
and
Zhong, J.-Q.
2021.
Flow structures and heat transport in Taylor–Couette systems with axial temperature gradient.
Journal of Fluid Mechanics,
Vol. 920,
Issue. ,
Zikanov, Oleg
Belyaev, Ivan
Listratov, Yaroslav
Frick, Peter
Razuvanov, Nikita
and
Sviridov, Valentin
2021.
Mixed Convection in Pipe and Duct Flows With Strong Magnetic Fields.
Applied Mechanics Reviews,
Vol. 73,
Issue. 1,
Horn, Susanne
and
Aurnou, Jonathan M.
2022.
The Elbert range of magnetostrophic convection. I. Linear theory.
Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 478,
Issue. 2264,
Leng, X.-Y.
and
Zhong, J.-Q.
2022.
Mutual coherent structures for heat and angular momentum transport in turbulent Taylor-Couette flows.
Physical Review Fluids,
Vol. 7,
Issue. 4,
Guillou, Julien
Bergez, Wladimir
Zamansky, Rémi
Boulin, Anne
and
Tordjeman, Philippe
2022.
Liquid metal convection induced by the Lorentz force in a Rayleigh-Bénard cell.
Physical Review Fluids,
Vol. 7,
Issue. 7,
Grannan, Alexander M.
Cheng, Jonathan S.
Aggarwal, Ashna
Hawkins, Emily K.
Xu, Yufan
Horn, Susanne
Sánchez-Álvarez, Jose
and
Aurnou, Jonathan M.
2022.
Experimental pub crawl from Rayleigh–Bénard to magnetostrophic convection.
Journal of Fluid Mechanics,
Vol. 939,
Issue. ,
Nicoski, Justin A.
Yan, Ming
and
Calkins, Michael A.
2022.
Quasistatic magnetoconvection with a tilted magnetic field.
Physical Review Fluids,
Vol. 7,
Issue. 4,
Xu, Yufan
Horn, Susanne
and
Aurnou, Jonathan M.
2022.
Thermoelectric precession in turbulent magnetoconvection.
Journal of Fluid Mechanics,
Vol. 930,
Issue. ,
Bader, Shujaut H.
and
Zhu, Xiaojue
2023.
Scaling relations in quasi-static magnetoconvection with a strong vertical magnetic field.
Journal of Fluid Mechanics,
Vol. 976,
Issue. ,
Krasnov, Dmitry
Akhtari, Ali
Zikanov, Oleg
and
Schumacher, Jörg
2023.
Tensor-product-Thomas elliptic solver for liquid-metal magnetohydrodynamics.
Journal of Computational Physics,
Vol. 474,
Issue. ,
p.
111784.
McCormack, Matthew
Teimurazov, Andrei
Shishkina, Olga
and
Linkmann, Moritz
2023.
Wall mode dynamics and transition to chaos in magnetoconvection with a vertical magnetic field.
Journal of Fluid Mechanics,
Vol. 975,
Issue. ,
Mandal, Sutapa
Sarkar, Snehashish
and
Pal, Pinaki
2023.
Effect of horizontal magnetic field on Küppers–Lortz instability.
Physics of Fluids,
Vol. 35,
Issue. 7,
Xu, Yufan
Horn, Susanne
and
Aurnou, Jonathan M.
2023.
Transition from wall modes to multimodality in liquid gallium magnetoconvection.
Physical Review Fluids,
Vol. 8,
Issue. 10,
Chen, Zhao-Qi
Wang, Zeng-Hui
Chen, Long
and
Ahmed, Mahmoud
2023.
Heat Transfer Enhancement of Liquid Metal Thermal Convection Affected by the Seebeck Effect and Magnetic Field.
International Journal of Energy Research,
Vol. 2023,
Issue. ,
p.
1.
Xia, Ke-Qing
Huang, Shi-Di
Xie, Yi-Chao
and
Zhang, Lu
2023.
Tuning heat transport via coherent structure manipulation: recent advances in thermal turbulence.
National Science Review,
Vol. 10,
Issue. 6,
Huang, Ze-Lin
Guo, Xi-Li
Wu, Jian-Zhao
Wang, Bo-Fu
Chong, Kai Leong
and
Zhou, Quan
2023.
Rayleigh-number dependence of the critical vibration frequency in vibrating thermal turbulence.
Physical Review Fluids,
Vol. 8,
Issue. 11,
Bhattacharya, Shashwat
Boeck, Thomas
Krasnov, Dmitry
and
Schumacher, Jörg
2023.
Effects of strong fringing magnetic fields on turbulent thermal convection.
Journal of Fluid Mechanics,
Vol. 964,
Issue. ,
Zhu, Hai-Tao
Chen, Long
and
Ni, Ming-Jiu
2024.
Effects of wall conductivities on magnetoconvection in a cube.
Physical Review Fluids,
Vol. 9,
Issue. 4,
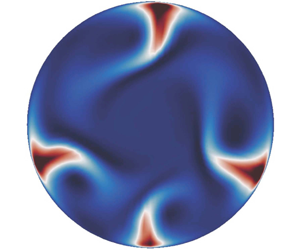
 $0.025$ and Rayleigh and Hartmann numbers up to
$0.025$ and Rayleigh and Hartmann numbers up to  $10^{9}$ and
$10^{9}$ and  $1400$, respectively, are considered. The results are consistent with those of earlier experimental and numerical data. As anticipated, the heat transfer rate and kinetic energy are suppressed by a strong magnetic field. At the same time, their growth with Rayleigh number is found to be faster in flows at high Hartmann numbers. This behaviour is attributed to the newly discovered flow regime characterized by prominent quasi-two-dimensional structures reminiscent of vortex sheets observed earlier in simulations of magnetohydrodynamic turbulence. Rotating wall modes similar to those in Rayleigh–Bénard convection with rotation are found in flows near the Chandrasekhar linear stability limit. A detailed analysis of the spatial structure of the flows and its effect on global transport properties is reported.
$1400$, respectively, are considered. The results are consistent with those of earlier experimental and numerical data. As anticipated, the heat transfer rate and kinetic energy are suppressed by a strong magnetic field. At the same time, their growth with Rayleigh number is found to be faster in flows at high Hartmann numbers. This behaviour is attributed to the newly discovered flow regime characterized by prominent quasi-two-dimensional structures reminiscent of vortex sheets observed earlier in simulations of magnetohydrodynamic turbulence. Rotating wall modes similar to those in Rayleigh–Bénard convection with rotation are found in flows near the Chandrasekhar linear stability limit. A detailed analysis of the spatial structure of the flows and its effect on global transport properties is reported.

