Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-28T09:31:53.259Z Has data issue: false hasContentIssue false
  • English
  • Français

Turbulent flow in the bulk of Rayleigh–Bénard convection: small-scale properties in a cubic cell

Published online by Cambridge University Press:  28 March 2013

Matthias Kaczorowski  and
Ke-Qing Xia
Matthias Kaczorowski
Affiliation:
Department of Physics, The Chinese University of Hong Kong, Shatin, Hong Kong, China
Ke-Qing Xia*
Affiliation:
Department of Physics, The Chinese University of Hong Kong, Shatin, Hong Kong, China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The Rayleigh number ($\mathit{Ra}$) scaling of the global Bolgiano length scale ${L}_{B, global} $ and the local Bolgiano length scale ${L}_{B, centre} $ in the centre region of turbulent Rayleigh–Bénard convection are investigated for Prandtl numbers 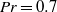$\mathit{Pr}= 0. 7$ and $4. 38$ and 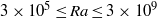$3\times 1{0}^{5} \leq \mathit{Ra}\leq 3\times 1{0}^{9} $. It is found that 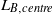${L}_{B, centre} $ does not necessarily exhibit the same scaling as 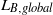${L}_{B, global} $. While 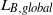${L}_{B, global} $ is monotonically deceasing as 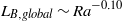${L}_{B, global} \sim {\mathit{Ra}}^{- 0. 10} $ for both 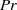$\mathit{Pr}$, ${L}_{B, centre} $ shows a steep increase beyond a certain 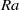$\mathit{Ra}$ value. The complex scaling of the local Bolgiano length scale in the centre is a result of the different behaviour of the temperature-variance dissipation rate, 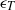${\epsilon }_{T} $, and the turbulent-kinetic-energy dissipation rate, 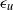${\epsilon }_{u} $. This shows that for sufficiently high 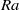$\mathit{Ra}$ the flow is well-mixed and hence temperature is passively advected. It is also observed that the 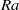$\mathit{Ra}$-range in which 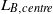${L}_{B, centre} $ exhibits the same scaling as the global Bolgiano length scale is increasing with increasing 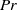$\mathit{Pr}$. It is further observed that for 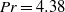$\mathit{Pr}= 4. 38$ and 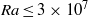$\mathit{Ra}\leq 3\times 1{0}^{7} $ the local vertical heat flux in the centre region is balanced by the turbulent-kinetic-energy dissipation rate. For higher 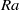$\mathit{Ra}$ we find that the local heat flux is decreasing. At 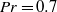$\mathit{Pr}= 0. 7$ we do not observe such a balance, as the measured heat flux is between the heat fluxes estimated through the turbulent-kinetic-energy dissipation rate and the temperature-variance dissipation rate. We therefore suggest that the balance of the local heat flux might be Prandtl-number dependent. The conditional average of the local vertical heat flux 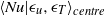$\mathop{\langle \mathit{Nu}\vert {\epsilon }_{u} , {\epsilon }_{T} \rangle }\nolimits_{\mathit{centre}} $ in the core region of the flow reveals that the highest vertical heat flux occurs for rare events with very high dissipation rates, while the joint most probable dissipation rates are associated with very low values of vertical heat flux. It is also observed that high values of 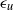${\epsilon }_{u} $ and 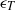${\epsilon }_{T} $ tend to occur together. It is further observed that the longitudinal velocity structure functions approach Kolmogorov K41 scaling. The temperature structure functions appear to approach Bolgiano–Obukhov BO59 scaling for 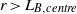$r\gt {L}_{B, centre} $, while a scaling exponent smaller than the BO59 scaling is observed for separations 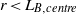$r\lt {L}_{B, centre} $. The mixed velocity and temperature structure function for 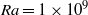$\mathit{Ra}= 1\times 1{0}^{9} $ and 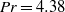$\mathit{Pr}= 4. 38$ shows a short $4/ 5$-scaling for $r\gt {L}_{B, centre} $. Our results suggest that BO59 scaling might be more clearly observable at higher Prandtl and moderate Rayleigh numbers.

JFM classification

Turbulent Flows: Isotropic turbulence Turbulent Flows: Turbulent convection
Type
Papers
Information
Journal of Fluid Mechanics , Volume 722 , 10 May 2013 , pp. 596 - 617
Copyright
©2013 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large-scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81 (2), 503–537.CrossRefGoogle Scholar
Benzi, R., Toschi, F. & Tripiccione, R. 1998 On the heat transfer in Rayleigh–Bénard systems. J. Stat. Phys. 93 (3/4).Google Scholar
Boffetta, G., De Lillo, F., Mazzino, A. & Musacchio, S. 2012 Bolgiano scale in confined Rayleigh–Taylor turbulence. J. Fluid Mech. 690, 426–440.CrossRefGoogle Scholar
Bolgiano, R. 1959 Turbulent spectra in a stably stratified atmosphere. J. Geophys. Res. 64 (12), 2226–2229.CrossRefGoogle Scholar
Calzavarini, E., Toschi, F. & Tripiccione, R. 2002 Evidences of Bolgiano scaling in 3D Rayleigh–Bénard convection. Phys. Rev. E 66, 016304.Google Scholar
Chillà, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh–Bénard convection. Eur. Phys. J. E 35 (58).Google Scholar
Daya, Z. A. & Ecke, R. E. 2001 Does turbulent convection feel the shape of the container? Phys. Rev. Lett. 87 (18), 184501.Google Scholar
Funfschilling, D., Brown, E., Nikolaenko, A. & Ahlers, G. 2005 Heat transport by turbulent Rayleigh–Bénard convection in cylindrical samples with aspect ratio one and larger. J. Fluid Mech. 536, 145–154.Google Scholar
Gasteuil, Y., Shew, W. L., Gibert, M., Chillà, F., Castaing, B. & Pinton, J.-F. 2007 Lagrangian temperature, velocity, and local heat flux measurement in Rayleigh–Bénard convection. Phys. Rev. Lett. 99 (234302).Google Scholar
Grossmann, S. & Lohse, D. 2000 Scaling in thermal convection: a unifying theory. J. Fluid Mech. 407, 27–56.Google Scholar
Grossmann, S. & Lohse, D. 2004 Fluctuations in turbulent Rayleigh–Bénard convection: the role of plumes. Phys. Fluids 16 (12), 4462–4472.Google Scholar
Grossmann, S. & Lohse, D. 2011 Multiple scaling in the ultimate regime of thermal convection. Phys. Fluids 23, 045108.CrossRefGoogle Scholar
He, X., Tong, P. & Xia, K.-Q. 2007 Measured dissipation field in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 98 (14), 144501.Google Scholar
Kaczorowski, M., Shishkin, A., Shishkina, O. & Wagner, C. 2008 New Results in Numerical and Experimental Fluid Mechanics VI, vol. 96, pp. 381–388. Springer.CrossRefGoogle Scholar
Kunnen, R. P. J., Clerx, H. J. H., Geurts, B. J., Bokhoven, L. J. A., van, Akkermans, R. A. D. & Verzicco, R. 2008 Numerical and experimental investigation of structure-function scaling in turbulent Rayleigh–Bénard convection. Phys. Rev. E 77, 016302.Google Scholar
Lohse, D. & Xia, K.-Q. 2010 Small-scale properties of turbulent Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 42, 335–364.Google Scholar
Ni, R., Huang, S.-D. & Xia, K.-Q. 2011 Local energy dissipation rate balances local heat flux in the centre of turbulent thermal convection. Phys. Rev. Lett. 107, 174503.CrossRefGoogle ScholarPubMed
Shang, X.-D., Qiu, X.-L., Tong, P. & Xia, K.-Q. 2004 Measurements of the local convective heat flux in turbulent Rayleigh–Bénard convection. Phys. Rev. E 70, 026308.Google Scholar
Shang, X.-D., Tong, P. & Xia, K.-Q. 2005 Test of steady-state fluctuation theorem in turbulent Rayleigh–Bénard convection. Phys. Rev. E 72, 015301.CrossRefGoogle ScholarPubMed
Shang, X.-D., Tong, P. & Xia, K.-Q. 2008 Scaling of the local convective heat flux in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 100, 244503.Google Scholar
Shishkina, O., Shishkin, A. & Wagner, C. 2009 Simulation of turbulent thermal convection in complicated domains. J. Comput. Appl. Maths 226, 336–344.Google Scholar
Shishkina, O., Stevens, R. J. A. M., Grossmann, S. & Lohse, D. 2010 Boundary layer structure in turbulent thermal convection and consequences for the required numerical resolution. New J. Phys. 12, 075022.Google Scholar
Sun, C., Zhou, Q. & Xia, K.-Q. 2006 Cascades of velocity and temperature fluctuations in buoyancy-driven thermal turbulence. Phys. Rev. Lett. 97, 144504.CrossRefGoogle ScholarPubMed
Wagner, S., Shishkina, O. & Wagner, C. 2012 Boundary layers and wind in cylindrical Rayleigh–Bénard cells. J. Fluid Mech. 697, 336–366.CrossRefGoogle Scholar
Xia, K.-Q., Lam, S. & Zhou, S.-Q. 2002 Heat-flux measurement in high-Prandtl-number turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 88 (6), 064501.Google Scholar
Zhou, Q., Sun, C. & Xia, K. Q. 2008 Experimental investigation of homogeneity, isotropy, and circulation of the velocity field in buoyancy-driven turbulence. J. Fluid Mech. 598, 361–372.CrossRefGoogle Scholar