Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-28T06:53:26.075Z Has data issue: false hasContentIssue false
  • English
  • Français

Turbulent drag reduction by compliant lubricating layer

Published online by Cambridge University Press:  24 January 2019

Alessio Roccon Open the ORCID record for Alessio Roccon [Opens in a new window] ,
Francesco Zonta Open the ORCID record for Francesco Zonta [Opens in a new window]  and
Alfredo Soldati Open the ORCID record for Alfredo Soldati [Opens in a new window]
Alessio Roccon
Affiliation:
Institute of Fluid Mechanics and Heat Transfer, TU-Wien, 1060 Vienna, Austria Polytechnic Department, University of Udine, 33100 Udine, Italy
Francesco Zonta
Affiliation:
Institute of Fluid Mechanics and Heat Transfer, TU-Wien, 1060 Vienna, Austria
Alfredo Soldati*
Affiliation:
Institute of Fluid Mechanics and Heat Transfer, TU-Wien, 1060 Vienna, Austria Polytechnic Department, University of Udine, 33100 Udine, Italy
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We propose a physically sound explanation for the drag reduction mechanism in a lubricated channel, a flow configuration in which an interface separates a thin layer of less-viscous fluid (viscosity $\unicode[STIX]{x1D702}_{1}$) from a main layer of a more-viscous fluid (viscosity 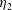$\unicode[STIX]{x1D702}_{2}$). To single out the effect of surface tension, we focus initially on two fluids having the same density and the same viscosity (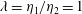$\unicode[STIX]{x1D706}=\unicode[STIX]{x1D702}_{1}/\unicode[STIX]{x1D702}_{2}=1$), and we lower the viscosity of the lubricating layer down to 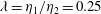$\unicode[STIX]{x1D706}=\unicode[STIX]{x1D702}_{1}/\unicode[STIX]{x1D702}_{2}=0.25$, which corresponds to a physically realizable experimental set-up consisting of light oil and water. A database comprising original direct numerical simulations of two-phase flow channel turbulence is used to study the physical mechanisms driving drag reduction, which we report between 20 and 30 percent. The maximum drag reduction occurs when the two fluids have the same viscosity (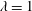$\unicode[STIX]{x1D706}=1$), and corresponds to the relaminarization of the lubricating layer. Decreasing the viscosity of the lubricating layer (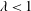$\unicode[STIX]{x1D706}<1$) induces a marginally decreased drag reduction, but also helps sustaining strong turbulence in the lubricating layer. This led us to infer two different mechanisms for the two drag-reduced systems, each of which is ultimately controlled by the outcome of the competition between viscous, inertial and surface tension forces.

JFM classification

Flow Control: Drag reduction Multiphase and Particle-laden Flows: Multiphase flow
Type
JFM Rapids
Information
Journal of Fluid Mechanics , Volume 863 , 25 March 2019 , R1
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahmadi, S., Roccon, A., Zonta, F. & Soldati, A. 2018a Turbulent drag reduction by a near wall surface tension active interface. Flow Turbul. Combust. 100 (4), 979–993.10.1007/s10494-018-9918-2Google Scholar
Ahmadi, S., Roccon, A., Zonta, F. & Soldati, A. 2018b Turbulent drag reduction in channel flow with viscosity stratified fluids. Comput. Fluids 176, 260–265.10.1016/j.compfluid.2016.11.007Google Scholar
Badalassi, V. E., Ceniceros, H. D. & Banerjee, S. 2003 Computation of multiphase systems with phase field models. J. Comput. Phys. 190 (2), 371–397.10.1016/S0021-9991(03)00280-8Google Scholar
Bai, R., Kelkar, K. & Joseph, D. D. 1996 Direct simulation of interfacial waves in a high-viscosity-ratio and axisymmetric coreannular flow. J. Fluid Mech. 327, 1–34.10.1017/S0022112096008440Google Scholar
Bannwart, A. C. 2001 Modeling aspects of oil–water core–annular flows. J. Petrol. Sci. Engng 32 (2–4), 127–143.10.1016/S0920-4105(01)00155-3Google Scholar
Brücker, C. 2015 Evidence of rare backflow and skin-friction critical points in near-wall turbulence using micropillar imaging. Phys. Fluids 27 (3), 031705.10.1063/1.4916768Google Scholar
Ding, H., Spelt, P. D. M. & Shu, C. 2007 Diffuse interface model for incompressible two-phase flows with large density ratios. J. Comput. Phys. 226 (2), 2078–2095.10.1016/j.jcp.2007.06.028Google Scholar
Ghosh, S., Mandal, T. K. & Das, P. K. 2009 Review of oil water core annular flow. Renew. Sust. Energy Rev. 13, 1957–1965.10.1016/j.rser.2008.09.034Google Scholar
Hu, Z., Morfey, C. L. & Sandham, N. D. 2006 Wall pressure and shear stress spectra from direct simulations of channel flow. AIAA J. 44 (7), 1541–1549.10.2514/1.17638Google Scholar
Isaac, J. D. & Speed, J. B.1904 Method of piping fluids. US Patent 759,374.Google Scholar
Jacqmin, D. 1999 Calculation of two-phase Navier–Stokes flows using phase-field modelling. J. Comput. Phys. 155 (1), 96–127.10.1006/jcph.1999.6332Google Scholar
Jiménez, J. 2013 Near-wall turbulence. Phys. Fluids 25, 101302.10.1063/1.4824988Google Scholar
Jiménez, J. & Moin, P. 1991 The minimal flow unit in near-wall turbulence. J. Fluid Mech. 225, 213–240.10.1017/S0022112091002033Google Scholar
Joseph, D. D., Bai, R., Chen, K. P. & Renardy, Y. Y. 1997 Core-annular flows. Annu. Rev. Fluid Mech. 29 (1), 65–90.10.1146/annurev.fluid.29.1.65Google Scholar
Joseph, D. D., Renardy, M. & Renardy, Y. 1984 Instability of the flow of two immiscible liquids with different viscosities in a pipe. J. Fluid Mech. 141, 309–317.10.1017/S0022112084000860Google Scholar
Joseph, D. D. & Renardy, Y. Y. 1993 Fundamentals of Two-fluid Dynamics: Lubricated Transport, Drops, and Miscible Liquids. Springer.Google Scholar
Kim, J. 2012 Phase-field models for multi-component fluid flows. Commun. Comput. Phys. 12 (3), 613–661.10.4208/cicp.301110.040811aGoogle Scholar
Lenaers, P., Li, Q., Brethouwer, G., Schlatter, P. & Örlü, R. 2012 Rare backflow and extreme wall-normal velocity fluctuations in near-wall turbulence. Phys. Fluids. 24 (3), 035110.10.1063/1.3696304Google Scholar
Looman, M. D.1916 Method of conveying oil. US Patent 1,192,438.Google Scholar
Oliemans, R. V. A. & Ooms, G. 1986 Multiphase Science and Technology (ed. Hewitt, G. F., Delhaye, J. M. & Zuber, N.), vol. 2, pp. 427–476. Springer.10.1007/978-3-662-01657-2_6Google Scholar
Pecnik, R. & Patel, A. 2017 Scaling and modelling of turbulence in variable property channel flows. J. Fluid Mech. 823.10.1017/jfm.2017.348Google Scholar
Roccon, A., De Paoli, M., Zonta, F. & Soldati, A. 2017 Viscosity-modulated breakup and coalescence of large drops in bounded turbulence. Phys. Rev. Fluids 2, 083603.10.1103/PhysRevFluids.2.083603Google Scholar
Schoppa, W. & Hussain, F. 2002 Coherent structure generation in near-wall turbulence. J. Fluid Mech. 453, 57–108.10.1017/S002211200100667XGoogle Scholar
Soldati, A. & Banerjee, S. 1998 Turbulence modification by large-scale organized electrohydrodynamic flows. Phys. Fluids 10 (7), 1742–1756.10.1063/1.869691Google Scholar
Soligo, G., Roccon, A. & Soldati, A. 2019 Coalescence of surfactant-laden drops by phase field method. J. Comput. Phys. 376, 1292–1311.10.1016/j.jcp.2018.10.021Google Scholar
Zonta, F., Marchioli, C. & Soldati, A. 2012 Modulation of turbulence in forced convection by temperature-dependent viscosity. J. Fluid Mech. 697, 150–174.10.1017/jfm.2012.67Google Scholar