Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-29T01:41:56.619Z Has data issue: false hasContentIssue false
  • English
  • Français

Thin-sheet flow between coalescing bubbles

Published online by Cambridge University Press:  20 May 2015

James P. Munro ,
Christopher R. Anthony ,
Osman A. Basaran  and
John R. Lister
James P. Munro*
Affiliation:
Institute of Theoretical Geophysics, Department of Applied Mathematics and Theoretical Physics, CMS, Wilberforce Road, Cambridge CB3 0WA, UK
Christopher R. Anthony
Affiliation:
School of Chemical Engineering, Purdue University, West Lafayette, IN 47907-1283, USA
Osman A. Basaran
Affiliation:
School of Chemical Engineering, Purdue University, West Lafayette, IN 47907-1283, USA
John R. Lister
Affiliation:
Institute of Theoretical Geophysics, Department of Applied Mathematics and Theoretical Physics, CMS, Wilberforce Road, Cambridge CB3 0WA, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

When two spherical bubbles touch, a hole is formed in the fluid sheet between them, and capillary pressure acting on its tightly curved edge drives an outward radial flow which widens the hole joining the bubbles. Recent images of the early stages of this process (Paulsen et al., Nat. Commun., vol. 5, 2014) show that the radius of the hole $r_{\!E}$ at time $t$ grows proportional to 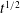$t^{1/2}$, and that the rate is dependent on the fluid viscosity. Here, we explain this behaviour in terms of similarity solutions to a third-order system of radial extensional-flow equations for the thickness and velocity of the sheet of fluid between the bubbles, and determine the growth rate as a function of the Ohnesorge number 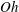$\mathit{Oh}$. The initially quadratic sheet profile allows the ratio of viscous and inertial effects to be independent of time. We show that the sheet is slender for $r_{\!E}\ll a$ if $\mathit{Oh}\gg 1$, where $a$ is the bubble radius, but only slender for 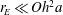$r_{\!E}\ll \mathit{Oh}^{2}a$ if 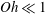$\mathit{Oh}\ll 1$ due to a compressional boundary layer of length $L\propto \mathit{Oh}\,r_{\!E}$, after which there is a change in the structure but not the speed of the retracting sheet. For 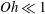$\mathit{Oh}\ll 1$, the detailed analysis justifies a simple momentum-balance argument, which gives the analytic prediction $r_{\!E}\sim (32a{\it\gamma}/3{\it\rho})^{1/4}t^{1/2}$, where 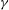${\it\gamma}$ is the surface tension and 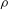${\it\rho}$ is the density.

JFM classification

Drops and Bubbles: Breakup/coalescence Interfacial Flows (free surface): Capillary flows Drops and Bubbles: Drops and Bubbles
Type
Rapids
Information
Journal of Fluid Mechanics , Volume 773 , 25 June 2015 , R3
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aarts, D. G. A. L., Lekkerkerker, H. N. W., Guo, H., Wegdam, G. H. & Bonn, D. 2005 Hydrodynamics of droplet coalescence. Phys. Rev. Lett. 95, 164503.Google Scholar
Billingham, J. 2005 The initial surface tension-driven flow of a wedge of viscous fluid. SIAM J. Appl. Maths 66, 510–532.Google Scholar
Blanchard, D. C. 1989 The ejection of drops from the sea and their enrichment with bacteria and other materials: a review. Estuaries 12 (3), 127–137.Google Scholar
Brenner, M. P. & Gueyffier, D. 1999 On the bursting of viscous films. Phys. Fluids 11 (3), 737–739.Google Scholar
Burton, J. C. & Taborek, P. 2007 Role of dimensionality and axisymmetry in fluid pinch-off and coalescence. Phys. Rev. Lett. 98, 224502.Google Scholar
Christodoulou, K. N. & Scriven, L. E. 1992 Discretization of free surface flows and other moving boundary problems. J. Comput. Phys. 99 (1), 39–55.Google Scholar
Culick, F. E. C. 1960 Comments on a ruptured soap film. J. Appl. Phys. 31, 1128–1129.Google Scholar
Davis, R. H., Schonberg, J. A. & Rallison, J. M. 1989 The lubrication force between two viscous drops. Phys. Fluids A 1 (1), 77–81.Google Scholar
Debrégeas, G., Martin, P. & Brochard-Wyart, F. 1995 Viscous bursting of suspended films. Phys. Rev. Lett. 75, 3886–3889.Google Scholar
Eggers, J. 2014 Post-breakup solutions of Navier–Stokes and Stokes threads. Phys. Fluids 26, 072104.Google Scholar
Eggers, J., Lister, J. R. & Stone, H. A. 1999 Coalescence of liquid drops. J. Fluid Mech. 401, 293–310.Google Scholar
Hopper, R. W. 1984 Coalescence of two equal cylinders: exact results for creeping viscous plane flow driven by capillarity. J. Am. Ceram. Soc. 67 (12), 262–264.Google Scholar
Keller, J. B. 1983 Breaking of liquid films and threads. Phys. Fluids 26 (12), 3451–3453.Google Scholar
Keller, J. B. & Miksis, M. J. 1983 Surface tension driven flows. SIAM J. Appl. Maths 43, 268–277.Google Scholar
Leal, L. G. 1990 Computational studies of drop and bubble dynamics in a viscous fluid. AIP Conf. Proc. 197 (1), 147–168.Google Scholar
Miksis, M. J. & Vanden-Broeck, J.-M. 1999 Self-similar dynamics of a viscous wedge of fluid. Phys. Fluids 11 (11), 3227–3231.Google Scholar
Notz, P. K. & Basaran, O. A. 2004 Dynamics and breakup of a contracting liquid filament. J. Fluid Mech. 512, 223–256.Google Scholar
Notz, P. K., Chen, A. U. & Basaran, O. A. 2001 Satellite drops: unexpected dynamics and change of scaling during pinch-off. Phys. Fluids 13 (3), 549–552.CrossRefGoogle Scholar
Paulsen, J. D., Burton, J. C., Nagel, S. R., Appathurai, S., Harris, M. T. & Basaran, O. A. 2012 The inexorable resistance of inertia determines the initial regime of drop coalescence. Proc. Natl Acad. Sci. USA 109 (18), 6857–6861.Google Scholar
Paulsen, J. D., Carmigniani, R., Burton, A. K. J. C. & Nagel, S. R. 2014 Coalescence of bubbles and drops in an outer fluid. Nat. Commun. 5, 3182.Google Scholar
Pegler, S. S. & Worster, M. G. 2012 Dynamics of a viscous layer flowing radially over an inviscid ocean. J. Fluid Mech. 696, 152–174.Google Scholar
Savva, N. & Bush, J. W. M. 2009 Viscous sheet retraction. J. Fluid Mech. 626, 211–240.Google Scholar
Sierou, A. & Lister, J. R. 2004 Self-similar recoil of inviscid drops. Phys. Fluids 16 (5), 1379–1394.Google Scholar
Sparks, R. S. J. 1978 The dynamics of bubble formation and growth in magmas: a review and analysis. J. Volcanol. Geotherm. Res. 3 (1–2), 1–37.Google Scholar
Taylor, G. I. 1959 The dynamics of thin sheets of fluid. III. Disintegration of fluid sheets. Proc. R. Soc. Lond. A 253, 313–321.Google Scholar
Ting, L. & Keller, J. B. 1990 Slender jets and thin sheets with surface tension. SIAM J. Appl. Maths 50, 1533–1546.Google Scholar