Hostname: page-component-669899f699-tzmfd Total loading time: 0 Render date: 2025-04-24T16:18:23.813Z Has data issue: false hasContentIssue false
  • English
  • Français

Supergranule aggregation: a Prandtl number-independent feature of constant heat flux-driven convection flows

Published online by Cambridge University Press:  06 February 2024

Philipp P. Vieweg Open the ORCID record for Philipp P. Vieweg [Opens in a new window]
Philipp P. Vieweg*
Affiliation:
Institute of Thermodynamics and Fluid Mechanics, Technische Universität Ilmenau, Postfach 100565, D-98684 Ilmenau, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

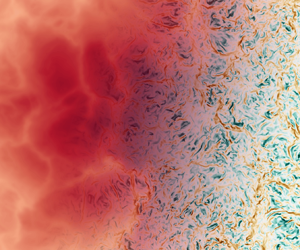
Supergranule aggregation, i.e. the gradual aggregation of convection cells to horizontally extended networks of flow structures, is a unique feature of constant heat flux-driven turbulent convection. In the present study, we address the question if this mechanism of self-organisation of the flow is present for any fluid. Therefore, we analyse three-dimensional Rayleigh–Bénard convection at a fixed Rayleigh number ${Ra} \approx 2.0 \times 10^{5}$ across $4$ orders of Prandtl numbers ${Pr} \in [10^{-2}, 10^{2}]$ by means of direct numerical simulations in horizontally extended periodic domains with aspect ratio $\varGamma = 60$. Our study confirms the omnipresence of the mechanism of supergranule aggregation for the entire range of investigated fluids. Moreover, we analyse the effect of ${Pr}$ on the global heat and momentum transport, and clarify the role of a potential stable stratification in the bulk of the fluid layer. The ubiquity of the investigated mechanism of flow self-organisation underlines its relevance for pattern formation in geophysical and astrophysical convection flows, the latter of which are often driven by prescribed heat fluxes.

JFM classification

Turbulent Flows: Turbulent convection Nonlinear Dynamical Systems: Pattern formation Convection: Buoyancy-driven instability
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 980 , 10 February 2024 , A46
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Atkinson, B.W. & Wu Zhang, J. 1996 Mesoscale shallow convection in the atmosphere. Rev. Geophys. 34, 403–431.Google Scholar
Batchelor, G.K. 1959 Small-scale variation of convected quantities like temperature in turbulent fluid. Part 1. General discussion and the case of small conductivity. J. Fluid Mech. 5, 113.Google Scholar
Boussinesq, J.V. 1903 Théorie Analytique de La Chaleur, vol. 2. Gauthier-Villars.Google Scholar
Busse, F.H. 1978 Non-linear properties of thermal convection. Rep. Prog. Phys. 41, 1929–1967.Google Scholar
Busse, F.H. 2003 The sequence-of-bifurcations approach towards understanding turbulent fluid flow. Surv. Geophys. 24, 269–288.Google Scholar
Chapman, C.J., Childress, S. & Proctor, M.R.E. 1980 Long wavelength thermal convection between non-conducting boundaries. Earth Planet. Sci. Lett. 51, 362–369.CrossRefGoogle Scholar
Chapman, C.J. & Proctor, M.R.E. 1980 Nonlinear Rayleigh–Bénard convection between poorly conducting boundaries. J. Fluid Mech. 101, 759–782.CrossRefGoogle Scholar
Chillà, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh–Bénard convection. Eur. Phys. J. E 35, 58.CrossRefGoogle ScholarPubMed
Christensen, U. 1995 Effects of phase transitions on mantle convection. Annu. Rev. Earth Planet. Sci. 23, 65–87.Google Scholar
Corrsin, S. 1951 On the spectrum of isotropic temperature fluctuations in an isotropic turbulence. J. Appl. Phys. 22, 469–473.Google Scholar
Dowling, T.E. 1988 Rotating Rayleigh–Bénard convection with fixed flux boundaries. In 1988 Summer Study Program in Geophysical Fluid Dynamics: The Influence of Convection on Large-Scale Circulations (ed. P.J. Goulart), pp. 230–247. Woods Hole Oceanographic Institution.Google Scholar
Fischer, P.F. 1997 An overlapping Schwarz method for spectral element solution of the incompressible Navier–Stokes equations. J. Comput. Phys. 133, 84–101.Google Scholar
Foroozani, N., Krasnov, D. & Schumacher, J. 2021 Turbulent convection for different thermal boundary conditions at the plates. J. Fluid Mech. 907, A27.Google Scholar
Hanson, C.S., Duvall, T.L., Birch, A.C., Gizon, L. & Sreenivasan, K.R. 2020 Solar east–west flow correlations that persist for months at low latitudes are dominated by active region inflows. Astron. Astrophys. 644, A103.Google Scholar
Hurle, D.T.J., Jakeman, R. & Pike, E.R. 1967 On the solution of the Bénard problem with boundaries of finite conductivity. Proc. R. Soc. Lond. A 296, 469–475.Google Scholar
Käufer, T., Vieweg, P.P., Schumacher, J. & Cierpka, C. 2023 Thermal boundary condition studies in large aspect ratio Rayleigh–Bénard convection. Eur. J. Mech. B-Fluids 101, 283–293.Google Scholar
Kolmogorov, A.N. 1991 The local structure of turbulence in incompressible viscous fluid for very large Reynolds numbers. Proc. Math. Phys. Sci. 434, 9–13.Google Scholar
Krug, D., Lohse, D. & Stevens, R.J.A.M. 2020 Coherence of temperature and velocity superstructures in turbulent Rayleigh–Bénard flow. J. Fluid Mech. 887, A2.Google Scholar
Mapes, B.E. & Houze, R.A. 1993 Cloud clusters and superclusters over the oceanic warm pool. Mon. Weath. Rev. 121, 1398–1416.Google Scholar
Maxworthy, T. & Narimousa, S. 1994 Unsteady, turbulent convection into a homogeneous, rotating fluid, with oceanographic applications. J. Phys. Oceanogr. 24, 865–887.2.0.CO;2>CrossRefGoogle Scholar
Oberbeck, A. 1879 Ueber die Wärmeleitung der Flüssigkeiten bei Berücksichtigung der Strömungen infolge von Temperaturdifferenzen. Ann. Phys. Chem. 243, 271–292.Google Scholar
Otero, J., Wittenberg, R.W., Worthing, R.A. & Doering, C.R. 2002 Bounds on Rayleigh–Bénard convection with an imposed heat flux. J. Fluid Mech. 473, 191–199.Google Scholar
Pandey, A., Krasnov, D., Sreenivasan, K.R. & Schumacher, J. 2022 Convective mesoscale turbulence at very low Prandtl numbers. J. Fluid Mech. 948, A23.Google Scholar
Pandey, A., Scheel, J.D. & Schumacher, J. 2018 Turbulent superstructures in Rayleigh–Bénard convection. Nat. Commun. 9, 2118.CrossRefGoogle ScholarPubMed
Parodi, A., von Hardenberg, J., Passoni, G., Provenzale, A. & Spiegel, E.A. 2004 Clustering of plumes in turbulent convection. Phys. Rev. Lett. 92, 194503.Google Scholar
Pellew, A. & Southwell, R.V. 1940 On maintained convective motion in a fluid heated from below. Proc. R. Soc. Lond. A 176, 312–343.Google Scholar
Rayleigh, Lord 1916 On convection currents in a horizontal layer of fluid, when the higher temperature is on the under side. Lond. Edinb. Dublin Philos. Mag. J. Sci. 32, 529–546.Google Scholar
Rincon, F. & Rieutord, M. 2018 The Sun's supergranulation. Living Rev. Sol. Phys. 15, 6.CrossRefGoogle Scholar
Scheel, J.D., Emran, M.S. & Schumacher, J. 2013 Resolving the fine-scale structure in turbulent Rayleigh–Bénard convection. New J. Phys. 15, 113063.Google Scholar
Schneide, C., Vieweg, P.P., Schumacher, J. & Padberg-Gehle, K. 2022 Evolutionary clustering of Lagrangian trajectories in turbulent Rayleigh–Bénard convection flows. Chaos 32, 013123.Google Scholar
Schumacher, J. & Sreenivasan, K.R. 2020 Colloquium: unusual dynamics of convection in the Sun. Rev. Mod. Phys. 92, 041001.Google Scholar
Sreenivasan, K.R. 2004 Possible effects of small-scale intermittency in turbulent reacting flows. Flow Turbul. Combust. 72, 115–131.CrossRefGoogle Scholar
Stevens, R.J.A.M., Blass, A., Zhu, X., Verzicco, R. & Lohse, D. 2018 Turbulent thermal superstructures in Rayleigh–Bénard convection. Phys. Rev. Fluids 3, 041501.Google Scholar
Takehiro, S.-I., Ishiwatari, M., Nakajima, K. & Hayashi, Y.-Y. 2002 Linear stability of thermal convection in rotating systems with fixed heat flux boundaries. Geophys. Astrophys. Fluid Dyn. 96, 439–459.Google Scholar
Vieweg, P.P. 2023 Large-scale flow structures in turbulent Rayleigh–Bénard convection: dynamical origin, formation, and role in material transport. PhD thesis, TU Ilmenau.Google Scholar
Vieweg, P.P., Klünker, A., Schumacher, J. & Padberg-Gehle, K. 2024 Lagrangian studies of coherent sets and heat transport in constant heat flux-driven turbulent Rayleigh–Bénard convection. Eur. J. Mech. B-Fluids 103, 69–85.Google Scholar
Vieweg, P.P., Scheel, J.D. & Schumacher, J. 2021 a Supergranule aggregation for constant heat flux-driven turbulent convection. Phys. Rev. Res. 3, 013231.Google Scholar
Vieweg, P.P., Scheel, J.D., Stepanov, R. & Schumacher, J. 2022 Inverse cascades of kinetic energy and thermal variance in three-dimensional horizontally extended turbulent convection. Phys. Rev. Res. 4, 043098.CrossRefGoogle Scholar
Vieweg, P.P., Schneide, C., Padberg-Gehle, K. & Schumacher, J. 2021 b Lagrangian heat transport in turbulent three-dimensional convection. Phys. Rev. Fluids 6, L041501.Google Scholar