Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Mousavi, Seyed Mahmood
Kamali, Reza
Sotoudeh, Freshteh
Karimi, Nader
and
Jeung, In-Seuck
2020.
Numerical Investigation of the Effects of Swirling Hot Co-Flow on MILD Combustion of a Hydrogen–Methane Blend.
Journal of Energy Resources Technology,
Vol. 142,
Issue. 11,
Habib, Rabeeah
Karimi, Nader
Yadollahi, Bijan
Doranehgard, Mohammad Hossein
and
Li, Larry K.B.
2020.
A pore-scale assessment of the dynamic response of forced convection in porous media to inlet flow modulations.
International Journal of Heat and Mass Transfer,
Vol. 153,
Issue. ,
p.
119657.
Habib, Rabeeah
Yadollahi, Bijan
and
Karimi, Nader
2020.
A Pore-Scale Investigation of the Transient Response of Forced Convection in Porous Media to Inlet Ramp Inputs.
Journal of Energy Resources Technology,
Vol. 142,
Issue. 11,
Mostafa Hosseinalipour, S.
Rahmani, Ebrahim
Fattahi, Abolfazl
and
Karimi, Nader
2020.
Experimental investigation of the hydrodynamic effects upon convecting entropy waves in nozzle flows.
Aerospace Science and Technology,
Vol. 107,
Issue. ,
p.
106301.
Habib, Rabeeah
Yadollahi, Bijan
Karimi, Nader
and
Doranegard, Mohammad Hossein
2020.
On the unsteady forced convection in porous media subject to inlet flow disturbances-A pore-scale analysis.
International Communications in Heat and Mass Transfer,
Vol. 116,
Issue. ,
p.
104639.
Weilenmann, Markus
Xiong, Yuan
and
Noiray, Nicolas
2020.
On the dispersion of entropy waves in turbulent flows.
Journal of Fluid Mechanics,
Vol. 903,
Issue. ,
Fattahi, Abolfazl
Karimi, Nader
and
Hajialigol, Najmeh
2020.
Dynamics of entropy wave generation in a simplified model of gas turbine combustor: A theoretical investigation.
Physics of Fluids,
Vol. 32,
Issue. 10,
Weilenmann, Markus
and
Noiray, Nicolas
2021.
Experiments on sound reflection and production by choked nozzle flows subject to acoustic and entropy waves.
Journal of Sound and Vibration,
Vol. 492,
Issue. ,
p.
115799.
Mesgarpour, Mehrdad
Abad, Javad Mohebbi Najm
Alizadeh, Rasool
Wongwises, Somchai
Doranehgard, Mohammad Hossein
Ghaderi, Saeidreza
and
Karimi, Nader
2021.
Prediction of the spread of Corona-virus carrying droplets in a bus - A computational based artificial intelligence approach.
Journal of Hazardous Materials,
Vol. 413,
Issue. ,
p.
125358.
Mohebbi Najm Abad, Javad
Alizadeh, Rasool
and
Mesgarpour, Mehrdad
2021.
Prediction of the spread of Corona-virus carrying droplets in a metro wagon - A computational based artificial intelligence approach.
Journal of Control,
Vol. 14,
Issue. 5,
p.
15.
Notaristefano, Andrea
and
Gaetani, Paolo
2021.
Transport of Swirling Entropy Waves through an Axial Turbine Stator.
International Journal of Turbomachinery, Propulsion and Power,
Vol. 6,
Issue. 4,
p.
45.
Gong, Y.
Jones, W. P.
and
Marquis, A. J.
2021.
Numerical analysis of indirect noise generated by compositional inhomogeneities using large eddy simulation.
AIP Advances,
Vol. 11,
Issue. 11,
Mousavi, Seyed Mahmood
Kamali, Reza
Sotoudeh, Freshteh
Karimi, Nader
and
Lee, Bok Jik
2021.
Numerical Investigation of the Plasma-Assisted MILD Combustion of a CH4/H2 Fuel Blend Under Various Working Conditions.
Journal of Energy Resources Technology,
Vol. 143,
Issue. 6,
Guzmán-Iñigo, Juan
Durán, Ignacio
and
Morgans, A.S.
2021.
Scattering of entropy waves into sound by isolated aerofoils.
Journal of Fluid Mechanics,
Vol. 923,
Issue. ,
Jahanbakhshi, Akram
Nadooshan, Afshin Ahmadi
and
Bayareh, Morteza
2022.
Multi-objective optimization of microchannel heatsink with wavy microtube by combining response surface method and genetic algorithm.
Engineering Analysis with Boundary Elements,
Vol. 140,
Issue. ,
p.
12.
Mesgarpour, Mehrdad
Abad, Javad Mohebbi Najm
Alizadeh, Rasool
Wongwises, Somchai
Doranehgard, Mohammad Hossein
Jowkar, Saeed
and
Karimi, Nader
2022.
Predicting the effects of environmental parameters on the spatio-temporal distribution of the droplets carrying coronavirus in public transport – A machine learning approach.
Chemical Engineering Journal,
Vol. 430,
Issue. ,
p.
132761.
Mustafa, Jawed
Alqaed, Saeed
and
Sharifpur, Mohsen
2022.
Numerical study on performance of double-fluid parabolic trough solar collector occupied with hybrid non-Newtonian nanofluids: Investigation of effects of helical absorber tube using deep learning.
Engineering Analysis with Boundary Elements,
Vol. 140,
Issue. ,
p.
562.
Saeed, Ali
Karimi, Nader
and
Paul, Manosh C.
2022.
Computational assessment of the thermal response of a Li-ion battery module to transient loads.
Journal of Power Sources,
Vol. 552,
Issue. ,
p.
232217.
Jahanbakhshi, Akram
Nadooshan, Afshin Ahmadi
and
Bayareh, Morteza
2022.
Cooling of a lithium-ion battery using microchannel heatsink with wavy microtubes in the presence of nanofluid.
Journal of Energy Storage,
Vol. 49,
Issue. ,
p.
104128.
Öztop, Mesut
and
Şahinaslan, Apdulmutalip
2022.
Control of temperature distribution for Li-ion battery modules via longitudinal fins.
Journal of Energy Storage,
Vol. 52,
Issue. ,
p.
104760.
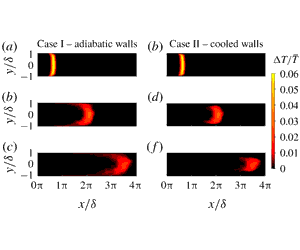
 $12.5\,\%$ of the total trace of entropy wave advection to predict the wave dynamics accurately. The results further reveal that the existing linear low-order models are truly predictive only for the entropy waves with less than
$12.5\,\%$ of the total trace of entropy wave advection to predict the wave dynamics accurately. The results further reveal that the existing linear low-order models are truly predictive only for the entropy waves with less than  $2\,\%$ increase in the gas temperature compared to that of the surrounding flow. Yet, in agreement with the assumption of existing models, it is shown that entropy waves travel with the mean flow speed.
$2\,\%$ increase in the gas temperature compared to that of the surrounding flow. Yet, in agreement with the assumption of existing models, it is shown that entropy waves travel with the mean flow speed.