Article contents
Stability of the Prandtl model for katabatic slope flows
Published online by Cambridge University Press: 28 February 2019
Abstract
We investigate the stability of the Prandtl model for katabatic slope flows using both linear stability theory and direct numerical simulations. Starting from Prandtl’s analytical solution for uniformly cooled laminar slope flows, we use linear stability theory to identify the onset of instability and features of the most unstable modes. Our results show that the Prandtl model for parallel katabatic slope flows is prone to transverse and longitudinal modes of instability. The transverse mode of instability manifests itself as stationary vortical flow structures aligned in the along-slope direction, whereas the longitudinal mode of instability emerges as waves propagating in the base-flow direction. Beyond the stability limits, these two modes of instability coexist and form a complex flow structure crisscrossing the plane of flow. The emergence of a particular form of these instabilities depends strongly on three dimensionless parameters, which are the slope angle, the Prandtl number and a newly introduced stratification perturbation parameter, which is proportional to the relative importance of the disturbance to the background stratification due to the imposed surface buoyancy flux. We demonstrate that when this parameter is sufficiently large, then the stabilising effect of the background stratification can be overcome. For shallow slopes, the transverse mode of instability emerges despite meeting the Miles–Howard stability criterion of 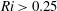 $Ri>0.25$. At steep slope angles, slope flow can remain linearly stable despite attaining Richardson numbers as low as
$Ri>0.25$. At steep slope angles, slope flow can remain linearly stable despite attaining Richardson numbers as low as 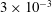 $3\times 10^{-3}$.
$3\times 10^{-3}$.
- Type
- JFM Rapids
- Information
- Copyright
- © 2019 Cambridge University Press
References
- 12
- Cited by


