Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-25T15:54:08.708Z Has data issue: false hasContentIssue false
  • English
  • Français

Sources and fluxes of scale energy in the overlap layer of wall turbulence

Published online by Cambridge University Press:  20 April 2015

A. Cimarelli ,
E. De Angelis ,
P. Schlatter ,
G. Brethouwer ,
A. Talamelli  and
C. M. Casciola
A. Cimarelli
Affiliation:
CIRI – Aeronautica, Università di Bologna, Via Fontanelle 40, 47121 Forlì FC, Italy
E. De Angelis*
Affiliation:
Dipartimento di Ingegneria Industriale, Università di Bologna, Via Fontanelle 40, 47121 Forlì FC, Italy
P. Schlatter
Affiliation:
Linné FLOW Centre, KTH Mechanics, SE-100 44 Stockholm, Sweden
G. Brethouwer
Affiliation:
Linné FLOW Centre, KTH Mechanics, SE-100 44 Stockholm, Sweden
A. Talamelli
Affiliation:
Dipartimento di Ingegneria Industriale, Università di Bologna, Via Fontanelle 40, 47121 Forlì FC, Italy
C. M. Casciola
Affiliation:
Dipartimento di Ingegneria Meccanica e Aerospaziale, Università di Roma ‘La Sapienza’, Piazzale Aldo Moro 5, 00185 Rome, Italy
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Direct numerical simulations of turbulent channel flows at friction Reynolds numbers (Re) of 550, 1000 and 1500 are used to analyse the turbulent production, transfer and dissipation mechanisms in the compound space of scales and wall distances by means of the Kolmogorov equation generalized to inhomogeneous anisotropic flows. Two distinct peaks of scale-energy source are identified. The first, stronger one, belongs to the near-wall cycle. Its location in the space of scales and physical space is found to scale in viscous units, while its intensity grows slowly with 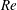$\mathit{Re}$, indicating a near-wall modulation. The second source peak is found further away from the wall in the putative overlap layer, and it is separated from the near-wall source by a layer of significant scale-energy sink. The dynamics of the second outer source appears to be strongly dependent on the Reynolds number. The detailed scale-by-scale analysis of this source highlights well-defined features that are used to make the properties of the outer turbulent source independent of Reynolds number and wall distance by rescaling the problem. Overall, the present results suggest a strong connection of the observed outer scale-energy source with the presence of an outer region of turbulence production whose mechanisms are well separated from the near-wall region and whose statistical features agree with the hypothesis of an overlap layer dominated by attached eddies. Inner–outer interactions between the near-wall and outer source region in terms of scale-energy fluxes are also analysed. It is conjectured that the near-wall modulation of the statistics at increasing Reynolds number can be related to a confinement of the near-wall turbulence production due to the presence of increasingly large production scales in the outer scale-energy source region.

JFM classification

Turbulent Flows: Turbulent boundary layers Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 771 , 25 May 2015 , pp. 407 - 423
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

del Alamo, J. C., Jiménez, J., Zandonade, P. & Moser, R. D. 2004 Scaling of the energy spectra of turbulent channels. J. Fluid Mech. 500, 135–144.CrossRefGoogle Scholar
del Alamo, J. C., Jiménez, J., Zandonade, P. & Moser, R. D. 2006 Self-similar vortex clusters in the turbulent logarithmic region. J. Fluid Mech. 561, 329–358.CrossRefGoogle Scholar
Casciola, C. M., Gualtieri, P., Jacob, B. & Piva, R. 2005 Scaling properties in the production range of shear dominated flows. Phys. Rev. Lett. 95, 024503.Google Scholar
Chevalier, M., Schlatter, P., Lundbladh, A. & Henningson, D. S.(2007) SIMSON – a pseudo-spectral solver for incompressible boundary layer flows. Tech. Rep. TRITA-MEK 2007:07. KTH Mechanics, Stockholm, Sweden.Google Scholar
Cimarelli, A. & De Angelis, E. 2011 Analysis of the Kolmogorov equation for filtered wall-turbulent flows. J. Fluid Mech. 676, 376–395.Google Scholar
Cimarelli, A. & De Angelis, E. 2012 Anisotropic dynamics and sub-grid energy transfer in wall-turbulence. Phys. Fluids 24 (1), 015102.Google Scholar
Cimarelli, A. & De Angelis, E. 2014 The physics of energy transfer toward improved subgrid-scale models. Phys. Fluids 26, 055103.CrossRefGoogle Scholar
Cimarelli, A., De Angelis, E. & Casciola, C. M. 2013 Paths of energy in turbulent channel flows. J. Fluid Mech. 715, 436–451.Google Scholar
Davidson, P. A., Nickels, T. B. & Krogstad, P. A. 2006 The logarithmic structure function law in wall-layer turbulence. J. Fluid Mech. 550, 51–60.Google Scholar
DeGraaff, D. B. & Eaton, J. K. 2000 Reynolds-number scaling of the flat-plate turbulent boundary layer. J. Fluid Mech. 422, 319–346.Google Scholar
Flores, O. & Jiménez, J. 2006 Effect of wall-boundary disturbances on turbulent channel flows. J. Fluid Mech. 566, 357–376.CrossRefGoogle Scholar
Hamilton, J. M., Kim, J. & Waleffe, F. 1995 Regeneration mechanisms of near-wall turbulence structures. J. Fluid Mech. 287, 317–348.CrossRefGoogle Scholar
Hill, R. J. 2002 Exact second-order structure–function relationship. J. Fluid Mech. 468, 317–326.CrossRefGoogle Scholar
Hutchins, N. & Marusic, I. 2007a Evidence of very long meandering streamwise structures in the logarithmic region of turbulent boundary layers. J. Fluid Mech. 579, 1–28.Google Scholar
Hutchins, N. & Marusic, I. 2007 b Large-scale influences in near-wall turbulence. Phil. Trans. R. Soc. Lond. A 365, 647–664.Google ScholarPubMed
Hwang, Y. & Cossu, C. 2010 Self-sustained process at large scales in turbulent channel flow. Phys. Rev. Lett. 105, 044505.CrossRefGoogle ScholarPubMed
Jacob, B., Casciola, C. M., Talamelli, A. & Alfredsson, H. P. 2008 Scaling of mixed structure functions in turbulent boundary layers. Phys. Fluids 20, 045101.Google Scholar
Jeong, J., Hussain, F., Schoppa, W. & Kim, J. 1997 Coherent structures near the wall in a turbulent channel flow. J. Fluid Mech. 332, 185–214.CrossRefGoogle Scholar
Jiménez, J. 2012 Cascades in wall-bounded turbulence. Annu. Rev. Fluid Mech. 44, 27–45.CrossRefGoogle Scholar
Jiménez, J. & Hoyas, S. 2008 Turbulent fluctuations above the buffer layer of wall-bounded flows. J. Fluid Mech. 611, 215–236.Google Scholar
Jiménez, J. & Pinelli, A. 1999 The autonomous cycle of near-wall turbulence. J. Fluid Mech. 389, 335–359.CrossRefGoogle Scholar
Kim, H. T., Kline, S. J. & Reynolds, W. C. 1971 The production of turbulence near a smooth wall in a turbulent boundary layer. J. Fluid Mech. 50, 133–160.CrossRefGoogle Scholar
Lenaers, P., Li, Q., Brethouwer, G., Schlatter, P. & Örlü, R. 2012 Rare backflow and extreme wall-normal velocity fluctuations in near-wall turbulence. Phys. Fluids 24, 035110.Google Scholar
Lozano-Duran, A. & Jiménez, J. 2014 Time-resolved evolution of coherent structures in turbulent channels: characterization of eddies and cascades. J. Fluid Mech. 759, 432–471.Google Scholar
Lumley, J. L. 1965 Interpretation of time spectra measured in high-intensity shear flows. Phys. Fluids 8, 1056–1062.Google Scholar
Marati, N., Casciola, C. M. & Piva, R. 2004 Energy cascade and spatial fluxes in wall turbulence. J. Fluid Mech. 521, 191–215.Google Scholar
Marusic, I., Mathis, R. & Hutchins, N. 2010a Predictive model for wall-bounded turbulent flow. Science 329, 193–196.Google Scholar
Marusic, I., McKeon, B. J., Monkewitz, P. A., Nagib, H. M., Smits, A. J. & Sreenivasan, K. R. 2010b Wall-bounded turbulent flows at high Reynolds numbers: recent advances and key issues. Phys. Fluids 22, 065103.Google Scholar
Mathis, R., Hutchins, N. & Marusic, I. 2009 Large-scale amplitude modulation of the small-scale structures of turbulent boundary layers. J. Fluid Mech. 628, 311–337.Google Scholar
Mizuno, Y. & Jiménez, J. 2013 Wall turbulence without walls. J. Fluid Mech. 723, 429–455.Google Scholar
Moarref, R., Sharma, A. S., Tropp, J. A. & McKeon, B. J. 2013 Model-based scaling of the streamwise energy density in high-Reynolds-number turbulent channels. J. Fluid Mech. 734, 275–316.CrossRefGoogle Scholar
Monty, J. P., Stewart, J. A., Williams, R. C. & Chong, M. S. 2007 Large-scale features of turbulent pipe and channel flows. J. Fluid Mech. 589, 147–156.Google Scholar
Nagib, H. M. & Chauhan, K. A. 2008 Variations of von Karman coefficient in canonical flows. Phys. Fluids 20, 101518.Google Scholar
Nikora, V. 1999 Origin of the ‘ $-1$ ’ spectral law in wall-bounded turbulence. Phys. Rev. Lett. 83, 734–736.Google Scholar
Perry, A. E., Hanbest, S. M. & Chong, M. S. 1986 A theoretical and experimental study of wall turbulence. J. Fluid Mech. 165, 163–199.Google Scholar
Piomelli, U. & Balaras, E. 2002 Wall-layer models for large-eddy simulations. Annu. Rev. Fluid Mech. 34, 349–374.CrossRefGoogle Scholar
Robinson, S. K. 1991 Coherent motions in the turbulent boundary layer. Annu. Rev. Fluid Mech. 23, 601–639.Google Scholar
Saikrishnan, N., De Angelis, E., Longmire, E. K., Marusic, I., Casciola, C. M. & Piva, R. 2012 Reynolds number effects on scale energy balance in wall turbulence. Phys. Fluids 24, 015101.Google Scholar
Schoppa, W. & Hussain, F. 2002 Coherent structure generation in near-wall turbulence. J. Fluid Mech. 453, 57–108.CrossRefGoogle Scholar
Smith, C. R. & Metzler, S. P. 1983 The characteristics of low speed streaks in the near wall region of a turbulent boundary layer. J. Fluid Mech. 129, 27–54.Google Scholar
Smits, A. J., McKeon, B. J. & Marusic, I. 2011 High-Reynolds number wall turbulence. Annu. Rev. Fluid Mech. 43, 353–375.Google Scholar
Townsend, A. A. 1976 The Structure of Turbulent Shear Flow. Cambridge University Press.Google Scholar