Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-27T03:03:16.409Z Has data issue: false hasContentIssue false
  • English
  • Français

Shock-induced heating and transition to turbulence in a hypersonic boundary layer

Published online by Cambridge University Press:  21 December 2020

Lin Fu Open the ORCID record for Lin Fu [Opens in a new window] ,
Michael Karp Open the ORCID record for Michael Karp [Opens in a new window] ,
Sanjeeb T. Bose ,
Parviz Moin Open the ORCID record for Parviz Moin [Opens in a new window]  and
Javier Urzay Open the ORCID record for Javier Urzay [Opens in a new window]
Lin Fu
Affiliation:
Center for Turbulence Research, Stanford University, Stanford, CA94305, USA
Michael Karp
Affiliation:
Center for Turbulence Research, Stanford University, Stanford, CA94305, USA
Sanjeeb T. Bose
Affiliation:
Cascade Technologies Inc., Palo Alto, CA94303, USA
Parviz Moin
Affiliation:
Center for Turbulence Research, Stanford University, Stanford, CA94305, USA
Javier Urzay*
Affiliation:
Center for Turbulence Research, Stanford University, Stanford, CA94305, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

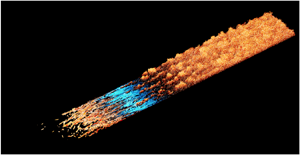
The interaction between an incident shock wave and a Mach-6 undisturbed hypersonic laminar boundary layer over a cold wall is addressed using direct numerical simulations (DNS) and wall-modelled large-eddy simulations (WMLES) at different angles of incidence. At sufficiently high shock-incidence angles, the boundary layer transitions to turbulence via breakdown of near-wall streaks shortly downstream of the shock impingement, without the need of any inflow free-stream disturbances. The transition causes a localized significant increase in the Stanton number and skin-friction coefficient, with high incidence angles augmenting the peak thermomechanical loads in an approximately linear way. Statistical analyses of the boundary layer downstream of the interaction for each case are provided that quantify streamwise spatial variations of the Reynolds analogy factors and indicate a breakdown of the Morkovin's hypothesis near the wall, where velocity and temperature become correlated. A modified strong Reynolds analogy with a fixed turbulent Prandtl number is observed to perform best. Conventional transformations fail at collapsing the mean velocity profiles on the incompressible log law. The WMLES prompts transition and peak heating, delays separation and advances reattachment, thereby shortening the separation bubble. When the shock leads to transition, WMLES provides predictions of DNS peak thermomechanical loads within $\pm 10\,\%$ at a computational cost lower than DNS by two orders of magnitude. Downstream of the interaction, in the turbulent boundary layer, the WMLES agrees well with DNS results for the Reynolds analogy factor, the mean profiles of velocity and temperature, including the temperature peak, and the temperature/velocity correlation.

JFM classification

Aerodynamics: Aerodynamics Compressible Flows: Shock waves Instability: Transition to turbulence
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 909 , 25 February 2021 , A8
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Adams, N. A. 2000 Direct simulation of the turbulent boundary layer along a compression ramp at $M=3$ and $Re_\theta = 1685$. J. Fluid Mech. 420, 47–83.CrossRefGoogle Scholar
Adler, M. C. & Gaitonde, D. V. 2018 Dynamic linear response of a shock/turbulent-boundary-layer interaction using constrained perturbations. J. Fluid Mech. 840, 291–341.10.1017/jfm.2018.70CrossRefGoogle Scholar
Bermejo-Moreno, I., Campo, L., Larsson, J., Bodart, J., Helmer, D. & Eaton, J. K. 2014 Confinement effects in shock wave/turbulent boundary layer interactions through wall-modelled large-eddy simulations. J. Fluid Mech. 758, 5–62.10.1017/jfm.2014.505CrossRefGoogle Scholar
Berridge, D. C., McKiernan, G., Wadhams, T. P., Holden, M., Wheaton, B. M., Wolf, T. D. & Schneider, S. P. 2018 Hypersonic ground tests in support of the boundary layer transition (bolt) flight experiment. In 2018 Fluid Dynamics Conference, p. 2893.Google Scholar
Bose, S. T. & Park, G. I. 2018 Wall-modeled large-eddy simulation for complex turbulent flows. Annu. Rev. Fluid Mech. 50, 535–561.CrossRefGoogle ScholarPubMed
Bradshaw, P. 1977 Compressible turbulent shear layers. Annu. Rev. Fluid Mech. 9, 33–54.10.1146/annurev.fl.09.010177.000341CrossRefGoogle Scholar
Bres, G. A., Bose, S. T., Emory, M., Ham, F. E., Schmidt, O. T., Rigas, G. & Colonius, T. 2018 Large-eddy simulations of co-annular turbulent jet using a Voronoi-based mesh generation framework. In 2018 AIAA/CEAS Aeroacoustics Conference, p. 3302.Google Scholar
Busemann, A. 1931 Handbuch der Experimentalphysik, vol. 4. Geest und Port.Google Scholar
Candler, G. V. 2019 Rate effects in hypersonic flows. Annu. Rev. Fluid Mech. 51 (1), 379–402.10.1146/annurev-fluid-010518-040258CrossRefGoogle Scholar
Cary, A. M. 1970 Summary of available information on Reynolds analogy for zero pressure gradient, compressible, turbulent boundary-layer flow. Tech. Rep. NASA TN D-5560.Google Scholar
Chandrashekar, P. 2013 Kinetic energy preserving and entropy stable finite volume schemes for compressible Euler and Navier–Stokes equations. Commun. Comput. Phys. 14 (5), 1252–1286.10.4208/cicp.170712.010313aCrossRefGoogle Scholar
Chi, S. W. & Spalding, D. B. 1966 Influence of temperature ratio on heat transfer to a flat plate through a turbulent boundary layer in air. In International Heat Transfer Conference, Chicago, IL, pp. 41–49.Google Scholar
Crocco, L. 1932 Sulla trasmissione del calore da una lamina piana a un fluido scorrente ad alta velocita. L'Aerotecnica 12, 181–197.Google Scholar
Currao, G. M. D., Choudhury, R., Gai, S. L., Neely, A. J. & Buttsworth, D. R. 2020 Hypersonic transitional shock-wave–boundary-layer interaction on a flat plate. AIAA J. 58 (2), 814–829.CrossRefGoogle Scholar
Davidson, T. S. C. & Babinsky, H. 2015 Transition location effects on normal shock wave-boundary layer interactions. AIAA Paper 2015-1975. American Institute of Aeronautics and Astronautics.10.2514/6.2015-1975CrossRefGoogle Scholar
Di Renzo, M. & Urzay, J. 2019 An a priori study of the accuracy of an equilibrium wall model for dissociating air in supersonic channel flows. In Annual Research Briefs, Center for Turbulence Research, pp. 29–40.Google Scholar
van Driest, E. R. 1956 The problem of aerodynamic heating. Aeronaut. Eng. Rev. 15 (10), 26–41.Google Scholar
Duan, L., Beekman, I. & Martin, M. P. 2010 Direct numerical simulation of hypersonic turbulent boundary layers. Part 2. Effect of wall temperature. J. Fluid Mech. 655, 419–445.10.1017/S0022112010000959CrossRefGoogle Scholar
Duan, L. & Martin, M. P. 2011 Direct numerical simulation of hypersonic turbulent boundary layers. Part 4. Effect of high enthalpy. J. Fluid Mech. 684, 25–59.10.1017/jfm.2011.252CrossRefGoogle Scholar
Dupont, P., Haddad, C., Ardissone, J. P. & Debiève, J. F. 2005 Space and time organisation of a shock wave/turbulent boundary layer interaction. Aerosp. Sci. Technol. 9 (7), 561–572.CrossRefGoogle Scholar
Dupont, P., Haddad, C. & Debiève, J. F. 2006 Space and time organization in a shock-induced separated boundary layer. J. Fluid Mech. 559, 255–277.CrossRefGoogle Scholar
Dupont, P., Piponniau, S., Sidorenko, A. & Debiève, J.-F. 2008 Investigation by particle image velocimetry measurements of oblique shock reflection with separation. AIAA J. 46 (6), 1365–1370.CrossRefGoogle Scholar
Dussauge, J.-P., Dupont, P. & Debiève, J.-F. 2006 Unsteadiness in shock wave boundary layer interactions with separation. Aerosp. Sci. Technol. 10 (2), 85–91.10.1016/j.ast.2005.09.006CrossRefGoogle Scholar
Fernholz, H. H. & Finley, P. J. 1980 A critical commentary on mean flow data for two-dimensional compressible turbulent boundary layers. Tech. Rep. AGARD-AG-253.Google Scholar
Gaitonde, D. V. 2013 Progress in shock wave/boundary layer interactions. AIAA Paper 2013-2607. American Institute of Aeronautics and Astronautics.CrossRefGoogle Scholar
Gatski, T. B. & Erlebacher, G. 2002 Numerical simulation of a spatially evolving supersonic turbulent boundary layer. NASA Tech. Rep. NASA/TM-2002-211934.Google Scholar
Gaviglio, J. 1987 Reynolds analogies and experimental study of heat transfer in the supersonic boundary layer. Intl J. Heat Mass Transfer 30 (5), 911–926.10.1016/0017-9310(87)90010-XCrossRefGoogle Scholar
Gottlieb, S., Shu, C.-W. & Tadmor, E. 2001 Strong stability-preserving high-order time discretization methods. SIAM Rev. 43 (1), 89–112.CrossRefGoogle Scholar
Guarini, S. E., Moser, R. D., Shariff, K. & Wray, A. 2000 Direct numerical simulation of a supersonic turbulent boundary layer at Mach 2.5. J. Fluid Mech. 414, 1–33.CrossRefGoogle Scholar
Hildebrand, N., Dwivedi, A., Nichols, J. W., Jovanović, M. R. & Candler, G. V. 2018 Simulation and stability analysis of oblique shock-wave/boundary-layer interactions at Mach 5.92. Phys. Rev. Fluids 3 (1), 013906.10.1103/PhysRevFluids.3.013906CrossRefGoogle Scholar
Huang, P. G., Coleman, G. N. & Bradshaw, P. 1995 Compressible turbulent channel flows: DNS results and modelling. J. Fluid Mech. 305, 185–218.CrossRefGoogle Scholar
Iyer, P. S. & Malik, M. R. 2019 Analysis of the equilibrium wall model for high-speed turbulent flows. Phys. Rev. Fluids 25, 074604.10.1103/PhysRevFluids.4.074604CrossRefGoogle Scholar
Kawai, S. & Larsson, J. 2012 Wall-modeling in large eddy simulation: length scales, grid resolution, and accuracy. Phys. Fluids 24 (1), 015105.10.1063/1.3678331CrossRefGoogle Scholar
Knight, D. & Mortazavi, M. 2017 Hypersonic shock wave transitional boundary layer interactions - a review. AIAA Paper 2017-3124. American Institute of Aeronautics and Astronautics.10.2514/6.2017-3124CrossRefGoogle Scholar
Lakebrink, M. T., Mani, M., Rolfe, E. N., Spyropoulos, J. T., Philips, D. A., Bose, S. T. & Mace, J. L. 2019 Toward improved turbulence-modeling techniques for internal-flow applications. AIAA Paper 2019-3703. American Institute of Aeronautics and Astronautics.CrossRefGoogle Scholar
Larsson, J., Laurence, S., Bermejo-Moreno, I., Bodart, J., Karl, S. & Vicquelin, R. 2015 Incipient thermal choking and stable shock-train formation in the heat-release region of a scramjet combustor. Part II: large eddy simulations. Combust. Flame 162, 907–920.CrossRefGoogle Scholar
Lash, E., Combs, C., Kreth, P., Beckman, E. & Schmisseur, J. 2016 Image-based analysis of the dynamics of transitional shock wave-boundary layer interactions. AIAA Paper 2016-4320. American Institute of Aeronautics and Astronautics.10.2514/6.2016-4320CrossRefGoogle Scholar
Lehmkuhl, O., Park, G. I., Bose, S. T. & Moin, P. 2018 Large-eddy simulation of practical aeronautical flows at stall conditions. In Proceedings of the 2018 Summer Program, Center for Turbulence Research, Stanford University, pp. 87–96.Google Scholar
Leyva, I. A. 2017 The relentless pursuit of hypersonic flight. Phys. Today 70 (11), 30–36.CrossRefGoogle Scholar
Lighthill, M. J. 1950 Contributions to the theory of heat transfer through a laminar boundary layer. Proc. R. Soc. A Math. Phys. 202 (1070), 359–377.Google Scholar
Loginov, M. S., Adams, N. A. & Zheltovodov, A. A. 2006 Large-eddy simulation of shock-wave/turbulent-boundary-layer interaction. J. Fluid Mech. 565, 135–169.10.1017/S0022112006000930CrossRefGoogle Scholar
Lozano-Durán, A., Bose, S. T. & Moin, P. 2020 Prediction of trailing edge separation on the NASA juncture flow using wall-modeled LES. AIAA Paper 2020-1776. American Institute of Aeronautics and Astronautics.CrossRefGoogle Scholar
Mack, L. M. 1984 Boundary-layer linear stability theory. Tech. Rep.. California Institute of Technology, Pasadena Jet Propulsion Lab.Google Scholar
Marco, N. & Komives, J. R. 2018 Wall-modeled large eddy simulation of a three-dimensional shock-boundary layer interaction. AIAA Paper 2018-1298. American Institute of Aeronautics and Astronautics.10.2514/6.2018-1298.c1CrossRefGoogle Scholar
Mettu, B. R. & Subbareddy, P. K. 2018 Wall modeled LES of compressible flows at non-equilibrium conditions. In 2018 AIAA Fluid Dynamics Conference, Atlanta, GA.CrossRefGoogle Scholar
Modesti, D. & Pirozzoli, S. 2016 Reynolds and Mach number effects in compressible turbulent channel flow. Intl J. Heat Fluid Flow 59, 33–49.CrossRefGoogle Scholar
Morkovin, M. V. 1962 Effects of compressibility on turbulent flows. In Mecanique de la Turbulence (ed. A. Favre), pp. 367–380.Google Scholar
Pirozzoli, S. & Bernardini, M. 2011 Turbulence in supersonic boundary layers at moderate Reynolds number. J. Fluid Mech. 688, 120–168.CrossRefGoogle Scholar
Pirozzoli, S., Bernardini, M. & Grasso, F. 2010 Direct numerical simulation of transonic shock/boundary layer interaction under conditions of incipient separation. J. Fluid Mech. 657, 361–393.CrossRefGoogle Scholar
Pirozzoli, S. & Grasso, F. 2006 Direct numerical simulation of impinging shock wave/turbulent boundary layer interaction at $M= 2.25$. Phys. Fluids 18 (6), 065113.CrossRefGoogle Scholar
Pirozzoli, S., Grasso, F. & Gatski, T. B. 2004 Direct numerical simulation and analysis of a spatially evolving supersonic turbulent boundary layer at $M= 2.25$. Phys. Fluids 16 (3), 530–545.CrossRefGoogle Scholar
Polivanov, P. A., Sidorenko, A. A. & Maslov, A. A. 2015 Transition effect on shock wave / boundary layer interaction at $M=1.47$. AIAA Paper 2015-1974. American Institute of Aeronautics and Astronautics.CrossRefGoogle Scholar
Robinet, J.-C. 2007 Bifurcations in shock-wave/laminar-boundary-layer interaction: global instability approach. J. Fluid Mech. 579, 85–112.10.1017/S0022112007005095CrossRefGoogle Scholar
Sandham, N. D. & Lüdeke, H. 2009 Numerical study of Mach 6 boundary-layer stabilization by means of a porous surface. AIAA J. 47 (9), 2243–2252.CrossRefGoogle Scholar
Sandham, N. D., Schülein, E., Wagner, A., Willems, S. & Steelant, J. 2014 Transitional shock-wave/boundary-layer interactions in hypersonic flow. J. Fluid Mech. 752, 349–382.CrossRefGoogle Scholar
Schneider, S. P. 2008 Development of hypersonic quiet tunnels. J. Spacecr. Rockets 45 (4), 641–664.CrossRefGoogle Scholar
Schülein, E. 2014 Effects of laminar-turbulent transition on the shock-wave/boundary-layer interaction. AIAA Paper 2014-3332. American Institute of Aeronautics and Astronautics.CrossRefGoogle Scholar
Tadmor, E. 2003 Entropy stability theory for difference approximations of nonlinear conservation laws and related time-dependent problems. Acta Numerica 12, 451–512.10.1017/S0962492902000156CrossRefGoogle Scholar
Thome, J., Knutson, A. & Candler, G. V. 2019 Boundary layer instabilities on BoLT subscale geometry. In AIAA Scitech 2019 Forum, p. 0092.Google Scholar
Touber, E. & Sandham, N. D. 2009 Large-eddy simulation of low-frequency unsteadiness in a turbulent shock-induced separation bubble. Theor. Comput. Fluid Dyn. 23 (2), 79–107.10.1007/s00162-009-0103-zCrossRefGoogle Scholar
Trettel, A. 2019 Transformations for variable-property turbulent boundary layers. PhD thesis, UCLA.Google Scholar
Trettel, A. & Larsson, J. 2016 Mean velocity scaling for compressible wall turbulence with heat transfer. Phys. Fluids 28 (2), 026102.CrossRefGoogle Scholar
Urzay, J. 2018 Supersonic combustion in air-breathing propulsion systems for hypersonic flight. Annu. Rev. Fluid Mech. 50, 593–627.CrossRefGoogle Scholar
Vanstone, L., Estruch-Samper, E., Hillier, R. & Ganapathisubramani, B. 2013 Shock-induced separation of transitional hypersonic boundary layers. AIAA Paper 2013-2736. American Institute of Aeronautics and Astronautics.CrossRefGoogle Scholar
Volpiani, P. S., Bernardini, M. & Larsson, J. 2018 Effects of a nonadiabatic wall on supersonic shock/boundary-layer interactions. Phys. Rev. Fluids 3 (8), 083401.CrossRefGoogle Scholar
Vreman, A. W. 2004 An eddy-viscosity subgrid-scale model for turbulent shear flow: algebraic theory and applications. Phys. Fluids 16 (10), 3670–3681.10.1063/1.1785131CrossRefGoogle Scholar
Walz, A. 1962 Compressible turbulent boundary layers, pp. 299–350. CNRS.Google Scholar
Walz, A. 1966 Strömungs-und Temperaturgrenzschichten. Braun.Google Scholar
Wheaton, B. M., Berridge, D. C., Wolf, T. D., Stevens, R. T. & McGrath, B. E. 2018 Boundary layer transition (BOLT) flight experiment overview. In 2018 Fluid Dynamics Conference, p. 2892.Google Scholar
Willems, S., Gülhan, A. & Steelant, J. 2015 Experiments on the effect of laminar–turbulent transition on the SWBLI in H2K at Mach 6. Exp. Fluids 56 (3), 49.CrossRefGoogle Scholar
Yang, X. I. A., Park, G. I. & Moin, P. 2017 a Log-layer mismatch and modeling of the fluctuating wall stress in wall-modeled large-eddy simulations. Phys. Rev. Fluids 2 (10), 104601.CrossRefGoogle ScholarPubMed
Yang, X. I. A., Urzay, J., Bose, S. T. & Moin, P. 2017 b Aerodynamic heating in wall-modeled large-eddy simulation of high-speed flows. AIAA J. 56, 731–742.CrossRefGoogle Scholar
Zhang, Y.-S., Bi, W.-T., Hussain, F. & She, Z.-S. 2014 A generalized Reynolds analogy for compressible wall-bounded turbulent flows. J. Fluid Mech. 739, 392–420.CrossRefGoogle Scholar