Hostname: page-component-586b7cd67f-r5fsc Total loading time: 0 Render date: 2024-12-01T09:12:45.702Z Has data issue: false hasContentIssue false
  • English
  • Français

Shear/rotation competition during the roll-up of acoustically excited shear layers

Published online by Cambridge University Press:  12 April 2018

Abbas Ghasemi Open the ORCID record for Abbas Ghasemi [Opens in a new window] ,
Burak Ahmet Tuna  and
Xianguo Li Open the ORCID record for Xianguo Li [Opens in a new window]
Abbas Ghasemi
Affiliation:
Department of Mechanical and Mechatronics Engineering, University of Waterloo 200 University Avenue West, Waterloo, Ontario, Canada N2L 3G1
Burak Ahmet Tuna
Affiliation:
Department of Mechanical and Mechatronics Engineering, University of Waterloo 200 University Avenue West, Waterloo, Ontario, Canada N2L 3G1
Xianguo Li*
Affiliation:
Department of Mechanical and Mechatronics Engineering, University of Waterloo 200 University Avenue West, Waterloo, Ontario, Canada N2L 3G1
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Naturally developing and acoustically excited shear layers at the Reynolds numbers 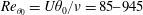$Re_{\unicode[STIX]{x1D703}_{0}}=U\unicode[STIX]{x1D703}_{0}/\unicode[STIX]{x1D708}=85{-}945$ are studied using the hot-wire (HW) anemometry and particle image velocimetry (PIV), with a focus on the shear/rotation competition during the initial Kelvin–Helmholtz (KH) roll-up. Velocity spectra and the spatial linear stability (LST) analysis characterize the fundamental (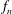$f_{n}$) and its subharmonic (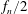$f_{n}/2$) mode interacting due to the vortex pairing. For 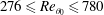$276\leqslant Re_{\unicode[STIX]{x1D703}_{0}}\leqslant 780$, the root-mean-square (r.m.s.) of the streamwise turbulence intensity shows a double-peaking phenomenon, i.e. major and minor peaks of the 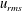$u_{rms}$ coexist towards the high-speed (HS) and the low-speed (LS) sides, respectively. The single/double-peaked $u_{rms}$ profiles are found to be correlated with the scattered/organized distribution of the shear/rotation, demonstrating a transitioning character with the downstream distance, 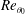$Re_{\unicode[STIX]{x1D703}_{0}}$ and the upstream turbulence levels. The rotating vortex cores and the corresponding peripheral shear regions, demonstrate the phase reversal of the velocity fluctuations with respect to the HS and the LS sides. Excitation at 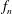$f_{n}$ increases the vortex count by 21 %, advances the location of the first KH roll-up and hence also the minor peak formation location. Due to the enhanced pairing at the 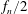$f_{n}/2$ forcing, the vortex count reduces by 23 %. Before merging into the downstream rotation core, the upstream vortex is shifted towards the HS side and the major peak is accordingly augmented. Actuation advances the transition to the nonlinear state, as well as the saturation of the amplification factor. The volumetric topologies of the shear/rotation loops tracked in consecutive phases during the period of the acoustic excitation, separate from the edge and grow in time–space due to the viscous diffusion. The shearing and rotating loops are found to be associated with the thinning (elongation) and expansion (accumulation) of the vorticity, respectively.

JFM classification

Flow Control: Instability control Turbulent Flows: Shear layer turbulence Vortex Flows: Vortex Flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 844 , 10 June 2018 , pp. 831 - 854
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aider, J.-L., Danet, A. & Lesieur, M. 2007 Large-eddy simulation applied to study the influence of upstream conditions on the time-dependant and averaged characteristics of a backward-facing step flow. J. Turbul. 8 (8), N51.10.1080/14685240701701000Google Scholar
Amitay, M., Tuna, B. A. & DellOrso, H. 2016 Identification and mitigation of ts waves using localized dynamic surface modification. Phys. Fluids 28 (6), 064103.10.1063/1.4953844Google Scholar
Aref, H. & Siggia, E. D. 1980 Vortex dynamics of the two-dimensional turbulent shear layer. J. Fluid Mech. 100 (4), 705–737.10.1017/S0022112080001371Google Scholar
Ayoub, A. & Karamcheti, K. 1982 An experiment on the flow past a finite circular cylinder at high subcritical and supercritical Reynolds numbers. J. Fluid Mech. 118, 1–26.10.1017/S0022112082000937Google Scholar
Betchov, R. 2012 Stability of Parallel Flows. Elsevier.Google Scholar
Bridges, T. J. & Morris, P. J. 1984 Differential eigenvalue problems in which the parameter appears nonlinearly. J. Comput. Phys. 55 (3), 437–460.10.1016/0021-9991(84)90032-9Google Scholar
Broadwell, J. E. & Breidenthal, R. E. 1982 A simple model of mixing and chemical reaction in a turbulent shear layer. J. Fluid Mech. 125, 397–410.10.1017/S0022112082003401Google Scholar
Browand, F. K. 1966 An experimental investigation of the instability of an incompressible, separated shear layer. J. Fluid Mech. 26 (2), 281–307.10.1017/S0022112066001241Google Scholar
Dini, P., Seligt, M. S. & Maughmert, M. D. 1992 Simplified linear stability transition prediction method for separated boundary layers. AIAA J. 3 (8), 1953–1961.10.2514/3.11165Google Scholar
Drazin, P. G. & Reid, W. H. 2004 Hydrodynamic Stability. Cambridge University Press.10.1017/CBO9780511616938Google Scholar
Freymuth, P. 1966 On transition in a separated laminar boundary layer. J. Fluid Mech. 25 (4), 683–704.10.1017/S002211206600034XGoogle Scholar
Ghasemi, A., Pereira, A. & Li, X. 2017 Large eddy simulation of compressible subsonic turbulent jet starting from a smooth contraction nozzle. Flow Turbul. Combust. 98 (1), 83–108.10.1007/s10494-016-9749-yGoogle Scholar
Ghasemi, A., Roussinova, V., Barron, R. M. & Balachandar, R. 2016 Large eddy simulation of the near-field vortex dynamics in starting square jet transitioning into steady state. Phys. Fluids 28 (8), 085104.10.1063/1.4961199Google Scholar
Graftieaux, L., Michard, M. & Grosjean, N. 2001 Combining piv, pod and vortex identification algorithms for the study of unsteady turbulent swirling flows. Meas. Sci. Technol. 12 (9), 1422–1429.10.1088/0957-0233/12/9/307Google Scholar
Hamelin, J. & Alving, A. E. 1996 A low-shear turbulent boundary layer. Phys. Fluids 8 (3), 789–804.10.1063/1.868862Google Scholar
Ho, C.-M. & Huerre, P. 1984 Perturbed free shear layers. Annu. Rev. Fluid Mech. 16 (1), 365–422.10.1146/annurev.fl.16.010184.002053Google Scholar
Hunt, J. C. R., Wray, A. A. & Moin, P.1988 Eddies, streams, and convergence zones in turbulent flows. Center for Turbulence Research Report CTR-S88, pp. 193–208.Google Scholar
Husain, H. S. & Hussain, F. 1995 Experiments on subharmonic resonance in a shear layer. J. Fluid Mech. 304, 343–372.10.1017/S0022112095004459Google Scholar
Hussain, A. K. M. F. & Zaman, K. B. M. Q. 1980 Vortex pairing in a circular jet under controlled excitation. Part 2. Coherent structure dynamics. J. Fluid Mech. 101 (3), 493–544.10.1017/S0022112080001772Google Scholar
Hussain, A. K. M. F. & Zaman, K. B. M. Q. 1985 An experimental study of organized motions in the turbulent plane mixing layer. J. Fluid Mech. 159, 85–104.10.1017/S0022112085003111Google Scholar
Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.10.1017/S0022112095000462Google Scholar
Kim, J. & Choi, H. 2009 Large eddy simulation of a circular jet: effect of inflow conditions on the near field. J. Fluid Mech. 620, 383–411.10.1017/S0022112008004722Google Scholar
Liepmann, D. & Gharib, M. 1992 The role of streamwise vorticity in the near-field entrainment of round jets. J. Fluid Mech. 245, 643–668.10.1017/S0022112092000612Google Scholar
Marusic, I. & Kunkel, G. J. 2003 Streamwise turbulence intensity formulation for flat-plate boundary layers. Phys. Fluids 15 (8), 2461–2464.10.1063/1.1589014Google Scholar
Marxen, O., Kotapati, R. B., Mittal, R. & Zaki, T. 2015 Stability analysis of separated flows subject to control by zero-net-mass-flux jet. Phys. Fluids 27 (2), 024107.10.1063/1.4907362Google Scholar
Meiron, D. I., Baker, G. R. & Orszag, S. A. 1982 Analytic structure of vortex sheet dynamics. Part 1. Kelvin–Helmholtz instability. J. Fluid Mech. 114, 283–298.10.1017/S0022112082000159Google Scholar
Michalke, A. 1965 On spatially growing disturbances in an inviscid shear layer. J. Fluid Mech. 23 (3), 521–544.10.1017/S0022112065001520Google Scholar
Michalke, A. 1972 The instability of free shear layers. Prog. Aerosp. Sci. 12, 213–216.10.1016/0376-0421(72)90005-XGoogle Scholar
Monkewitz, P. A. 1983 On the nature of the amplitude modulation of jet shear layer instability waves. Phys. Fluids 26 (11), 3180–3184.10.1063/1.864089Google Scholar
Monkewitz, P. A. & Huerre, P. 1982 Influence of the velocity ratio on the spatial instability of mixing layers. Phys. Fluids 25 (7), 1137–1143.10.1063/1.863880Google Scholar
Morris, S. C. & Foss, J. F. 2003 Turbulent boundary layer to single-stream shear layer: the transition region. J. Fluid Mech. 494, 187–221.10.1017/S0022112003006049Google Scholar
Orszag, S. A. 1971 Accurate solution of the Orr–Sommerfeld stability equation. J. Fluid Mech. 50 (4), 689–703.10.1017/S0022112071002842Google Scholar
Philip, J. & Marusic, I. 2012 Large-scale eddies and their role in entrainment in turbulent jets and wakes. Phys. Fluids 24 (5), 055108.10.1063/1.4719156Google Scholar
Saffman, P. G. 1992 Vortex Dynamics, Cambridge Monographs on Mechanics and Applied Mathematics, vol. 311, p. 368. Cambridge University Press.Google Scholar
Saffman, P. G. & Baker, G. R. 1979 Vortex interactions. Annu. Rev. Fluid Mech. 11 (1), 95–121.10.1146/annurev.fl.11.010179.000523Google Scholar
Sato, H. 1956 Experimental investigation on the transition of laminar separated layer. J. Phys. Soc. Japan 11 (6), 702–709.10.1143/JPSJ.11.702Google Scholar
Sato, H. 1959 Further investigation on the transition of two-dimensional separated layer at subsonic speeds. J. Phys. Soc. Japan 14 (12), 1797–1810.10.1143/JPSJ.14.1797Google Scholar
Sato, H. 1960 The stability and transition of a two-dimensional jet. J. Fluid Mech. 7 (1), 53–80.10.1017/S0022112060000049Google Scholar
Schmid, P. J. & Henningson, D. S. 2012 Stability and Transition in Shear Flows, vol. 142. Springer Science & Business Media.Google Scholar
Sciacchitano, A., Neal, D. R., Smith, B. L., Warner, S. O., Vlachos, P. P., Wieneke, B. & Scarano, F. 2015 Collaborative framework for piv uncertainty quantification: comparative assessment of methods. Meas. Sci. Technol. 26 (7), 074004.10.1088/0957-0233/26/7/074004Google Scholar
Slessor, M. D., Bond, C. L. & Dimotakis, P. E. 1998 Turbulent shear-layer mixing at high Reynolds numbers: effects of inflow conditions. J. Fluid Mech. 376, 115–138.10.1017/S0022112098002857Google Scholar
Smith, A. M. O. & Gamberoni, N.1956 Transition, pressure gradient, and stability theory. Report no. es. 26388, douglas aircraft co. Inc., El Segundo, CA.Google Scholar
Townsend, A. A. 1980 The Structure of Turbulent Shear Flow. Cambridge University Press.Google Scholar
Van Ingen, J. L.1956 A suggested semi-empirical method for the calculation of the boundary layer transition region. Technische Hogeschool Delft, Vliegtuigbouwkunde, Rapport VTH-74.Google Scholar
Van Ingen, J. L. 2008 The eN Method for Transition Prediction: Historical Review of Work at TU Delft, vol. 3830. AIAA.Google Scholar
Vassilicos, J. C., Laval, J.-P., Foucaut, J.-M. & Stanislas, M. 2015 The streamwise turbulence intensity in the intermediate layer of turbulent pipe flow. J. Fluid Mech. 774, 324–341.10.1017/jfm.2015.241Google Scholar
Westerweel, J. & Scarano, F. 2005 Universal outlier detection for piv data. Exp. Fluids 39 (6), 1096–1100.10.1007/s00348-005-0016-6Google Scholar
Winant, C. D. & Browand, F. K. 1974 Vortex pairing: the mechanism of turbulent mixing-layer growth at moderate Reynolds number. J. Fluid Mech. 63 (2), 237–255.10.1017/S0022112074001121Google Scholar
Yarusevych, S. & Kotsonis, M. 2017 Steady and transient response of a laminar separation bubble to controlled disturbances. J. Fluid Mech. 813, 955–990.10.1017/jfm.2016.848Google Scholar
Yule, A. J. 1978 Large scale structure in the mixing layer of a round jet. J. Fluid Mech. 89 (3), 413–432.10.1017/S0022112078002670Google Scholar
Zabusky, N. J. & Deem, G. S. 1971 Dynamical evolution of two-dimensional unstable shear flows. J. Fluid Mech. 47 (2), 353–379.10.1017/S0022112071001101Google Scholar
Zaman, K. B. M. Q. & Hussain, A. K. M. F. 1980 Vortex pairing in a circular jet under controlled excitation. Part 1. General jet response. J. Fluid Mech. 101 (3), 449–491.10.1017/S0022112080001760Google Scholar

Ghasemi et al. supplementary movie

Movie 1. Shear/rotation loops obtained from the planar phase-locked PIV measurements interpolated into volumetric topologies. The phases ϕ=00, 600, 1200, 1800, 2400, 3000 during the acoustic actuation of the fundamental mode animate the shear (blue) and rotation (red) loops of the <Q>p-criterion passed through the vorticity cut-planes (green). The shear loops are found correlated with the thinning in the stretched vorticity zones while the rotating loops intersect with the expansion/accumulation of the vorticity.

Download Ghasemi et al. supplementary movie(Video)
Video 8.7 MB