Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-29T06:50:11.583Z Has data issue: false hasContentIssue false
  • English
  • Français

Self-similar vortex-induced vibrations of a hanging string

Published online by Cambridge University Press:  08 May 2013

C. Grouthier ,
S. Michelin ,
Y. Modarres-Sadeghi  and
E. de Langre
C. Grouthier*
Affiliation:
LadHyX, Department of Mechanics, Ecole Polytechnique, 91128 Palaiseau, France
S. Michelin
Affiliation:
LadHyX, Department of Mechanics, Ecole Polytechnique, 91128 Palaiseau, France
Y. Modarres-Sadeghi
Affiliation:
Department of Mechanical and Industrial Engineering, University of Massachusetts, Amherst, MA 01003, USA
E. de Langre
Affiliation:
LadHyX, Department of Mechanics, Ecole Polytechnique, 91128 Palaiseau, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

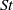An experimental analysis of the vortex-induced vibrations of a hanging string with variable tension along its length is presented in this paper. It is shown that standing waves develop along the hanging string. First, the evolution of the Strouhal number $\mathit{St}$ with the Reynolds number $\mathit{Re}$ follows a trend similar to what is observed for a circular cylinder in a flow for relatively low Reynolds numbers (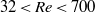$32\lt \mathit{Re}\lt 700$). Second, the extracted mode shapes are self-similar: a rescaling of the spanwise coordinate by a self-similarity coefficient allows all of them to collapse onto a unique function. The self-similar behaviour of the spatial distribution of the vibrations along the hanging string is then explained theoretically by performing a linear stability analysis of an adapted wake-oscillator model. This linear stability analysis finally provides an accurate description of the mode shapes and of the evolution of the self-similarity coefficient with the flow speed.

JFM classification

Vortex Flows: Vortex shedding
Type
Rapids
Information
Journal of Fluid Mechanics , Volume 724 , 10 June 2013 , R2
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Baarholm, G. S., Larsen, C. M. & Lie, H. 2006 On fatigue damage accumulation from in-line and cross-flow vortex-induced vibrations on risers. J. Fluids Struct. 22, 109–127.Google Scholar
Blevins, R. D. 1990 Flow-Induced Vibration, 2nd edn. Van Nostrand Reinhold.Google Scholar
Bourguet, R., Karniadakis, G. E. & Triantafyllou, M. S. 2011a Vortex-induced vibrations of a long flexible cylinder in shear flow. J. Fluid Mech. 677, 342–382.Google Scholar
Bourguet, R., Modarres-Sadeghi, Y., Karniadakis, G. E. & Triantafyllou, M. S. 2011b Wake–body resonance of long flexible structures is dominated by counterclockwise orbits. Phys. Rev. Lett. 107, 134502.Google Scholar
Chaplin, J. R., Bearman, P. W., Huera-Huarte, F. J. & Pattenden, R. J. 2005 Laboratory measurements of vortex-induced vibrations of a vertical tension riser in a stepped current. J. Fluids Struct. 21, 3–24.Google Scholar
Evangelinos, C. & Karniadakis, G. E. 1999 Dynamics and flow structures in the turbulent wake of rigid and flexible cylinders subject to vortex-induced vibrations. J. Fluid Mech. 400, 91–124.Google Scholar
Facchinetti, M. L., de Langre, E. & Biolley, F. 2004 Coupling of structure and wake oscillators in vortex-induced vibrations. J. Fluids Struct. 19, 123–140.Google Scholar
Fey, U., König, M. & Eckelmann, H. 1998 A new Strouhal–Reynolds-number relationship for the circular cylinder in the range $47\lt Re\lt 2\times 1{0}^{5} $ . Phys. Fluids 10 (7), 1547–1549.Google Scholar
Hartlen, R. T. & Currie, I. G. 1970 Lift-oscillator model for vortex-induced vibration. J. Engng Mech. ASCE 96, 577–591.Google Scholar
Huera-Huarte, F. J. & Bearman, P. W. 2009 Wake structures and vortex-induced vibrations of a long flexible cylinder – Part 1: Dynamic response. J. Fluids Struct. 25, 979–990.Google Scholar
Mathelin, L. & de Langre, E. 2005 Vortex-induced vibrations and waves unders shear flow with a wake oscillator model. Eur. J. Mech. (B/Fluids) 24, 478–490.CrossRefGoogle Scholar
Modarres-Sadeghi, Y., Chasparis, F., Triantafyllou, M. S., Tognarelli, M. & Beynet, P. 2011 Chaotic response is a generic feature of vortex-induced vibrations of flexible risers. J. Sound Vib. 330, 2565–2579.Google Scholar
Modarres-Sadeghi, Y., Mukundan, H., Dahl, J. M., Hover, F. S. & Triantafyllou, M. S. 2010 The effect of higher harmonic forces on fatigue life of marine risers. J. Sound Vib. 329, 43–55.Google Scholar
Naudascher, E. & Rockwell, D. 1990 Flow-Induced Vibration – an Engineering Guide. A.A. Balkema.Google Scholar
Newman, D. J. & Karniadakis, G. E. 1997 A direct numerical simulation study of flow past a freely vibrating cable. J. Fluid Mech. 344, 95–136.Google Scholar
Norberg, C. 2003 Fluctuating lift on a circular cylinder: review and new measurements. J. Fluids Struct. 17, 57–96.Google Scholar
Park, H. I., Hong, Y. P., Nakamura, M. & Koterayama, W. 2002 An experimental study on transverse vibrations of a highly flexibe free-hanging pipe in water. In Proceedings of the Twelfth International Offshore and Polar Engineering Conference, ISOPE, Kitakyushu, Japan, pp. 199–206. International Society of Offshore and Polar Engineers.Google Scholar
Srinil, N. 2010 Multi-mode interactions in vortex-induced vibrations of flexible curved/straight structures with geometric nonlinearities. J. Fluids Struct. 26, 1098–1122.Google Scholar
Srinil, N. 2011 Analysis and prediction of vortex-induced vibrations of variable-tension vertical risers in linearly sheared currents. Appl. Ocean Res. 33, 41–53.CrossRefGoogle Scholar
Triantafyllou, M. S. & Triantafyllou, G. S. 1991 The paradox of the hanging string: an explanation using singular perturbations. J. Sound Vib. 148 (2), 343–351.Google Scholar
Trim, A. D., Braaten, H., Lie, H. & Tognarelli, M. A. 2005 Experimental investigation of vortex-induced vibration of long marine risers. J. Fluids Struct. 21, 335–361.Google Scholar
Vandiver, J. K., Jaiswal, V. & Jhingran, V. 2009 Insights on vortex-induced, traveling waves on long risers. J. Fluids Struct. 25, 641–653.Google Scholar
Violette, R., de Langre, E. & Szydlowski, J. 2007 Computations of vortex-induced vibrations of long structures using a wake oscilator model: comparison with DNS and experiments. Comput. Struct. 85, 1134–1141.Google Scholar
Violette, R., de Langre, E. & Szydlowski, J. 2010 A linear stability approach to vortex-induced vibrations and waves. J. Fluids Struct. 26 (3), 442–466.Google Scholar
Williamson, C. H. K. & Brown, G. L. 1998 A series in $1/ \sqrt{Re} $ to represent the Strouhal–Reynolds number relationship of the cylinder wake. J. Fluids Struct. 12, 1073–1085.Google Scholar
Williamson, C. H. K. & Govardhan, R. 2004 Vortex-induced vibrations. Annu. Rev. Fluid Mech. 36, 413–455.CrossRefGoogle Scholar
Wu, X., Ge, F. & Hong, Y. 2012 A review of recent studies on vortex-induced vibrations of long slender cylinders. J. Fluids Struct. 28, 292–308.Google Scholar