Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-24T21:19:34.640Z Has data issue: false hasContentIssue false
  • English
  • Français

Role of natural convection in the dissolution of sessile droplets

Published online by Cambridge University Press:  30 March 2016

Erik Dietrich ,
Sander Wildeman ,
Claas Willem Visser ,
Kevin Hofhuis ,
E. Stefan Kooij ,
Harold J. W. Zandvliet  and
Detlef Lohse
Erik Dietrich
Affiliation:
Physics of Fluids Group, Department of Science and Technology, J. M. Burgers Center for Fluid Dynamics, and Mesa+ Institute for Nanotechnology, University of Twente, PO Box 217, 7500 AE Enschede, The Netherlands Physics of Interfaces and Nanomaterials, Department of Science and Technology, Mesa+ Institute for Nanotechnology, University of Twente, PO Box 217, 7500 AE Enschede, The Netherlands
Sander Wildeman
Affiliation:
Physics of Fluids Group, Department of Science and Technology, J. M. Burgers Center for Fluid Dynamics, and Mesa+ Institute for Nanotechnology, University of Twente, PO Box 217, 7500 AE Enschede, The Netherlands
Claas Willem Visser
Affiliation:
Physics of Fluids Group, Department of Science and Technology, J. M. Burgers Center for Fluid Dynamics, and Mesa+ Institute for Nanotechnology, University of Twente, PO Box 217, 7500 AE Enschede, The Netherlands
Kevin Hofhuis
Affiliation:
Physics of Interfaces and Nanomaterials, Department of Science and Technology, Mesa+ Institute for Nanotechnology, University of Twente, PO Box 217, 7500 AE Enschede, The Netherlands
E. Stefan Kooij
Affiliation:
Physics of Interfaces and Nanomaterials, Department of Science and Technology, Mesa+ Institute for Nanotechnology, University of Twente, PO Box 217, 7500 AE Enschede, The Netherlands
Harold J. W. Zandvliet
Affiliation:
Physics of Interfaces and Nanomaterials, Department of Science and Technology, Mesa+ Institute for Nanotechnology, University of Twente, PO Box 217, 7500 AE Enschede, The Netherlands
Detlef Lohse*
Affiliation:
Physics of Fluids Group, Department of Science and Technology, J. M. Burgers Center for Fluid Dynamics, and Mesa+ Institute for Nanotechnology, University of Twente, PO Box 217, 7500 AE Enschede, The Netherlands Max Planck Institute for Dynamics and Self-Organization, 37077 Goettingen, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

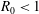The dissolution process of small (initial (equivalent) radius $R_{0}<1$  mm) long-chain alcohol (of various types) sessile droplets in water is studied, disentangling diffusive and convective contributions. The latter can arise for high solubilities of the alcohol, as the density of the alcohol–water mixture is then considerably less than that of pure water, giving rise to buoyancy-driven convection. The convective flow around the droplets is measured, using micro-particle image velocimetry (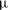${\rm\mu}$PIV) and the schlieren technique. When non-dimensionalizing the system, we find a universal $Sh\sim Ra^{1/4}$ scaling relation for all alcohols (of different solubilities) and all droplets in the convective regime. Here 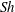$Sh$ is the Sherwood number (dimensionless mass flux) and $Ra$ is the Rayleigh number (dimensionless density difference between clean and alcohol-saturated water). This scaling implies the scaling relation 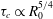${\it\tau}_{c}\propto R_{0}^{5/4}$ of the convective dissolution time 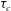${\it\tau}_{c}$, which is found to agree with experimental data. We show that in the convective regime the plume Reynolds number (the dimensionless velocity) of the detaching alcohol-saturated plume follows 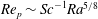$Re_{p}\sim Sc^{-1}Ra^{5/8}$, which is confirmed by the 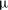${\rm\mu}$PIV data. Here, 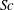$Sc$ is the Schmidt number. The convective regime exists when 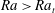$Ra>Ra_{t}$, where $Ra_{t}=12$ is the transition $Ra$ number as extracted from the data. For $Ra\leqslant Ra_{t}$ and smaller, convective transport is progressively overtaken by diffusion and the above scaling relations break down.

JFM classification

Convection: Convection Drops and Bubbles: Drops and Bubbles Convection: Plumes/thermals
Type
Papers
Information
Journal of Fluid Mechanics , Volume 794 , 10 May 2016 , pp. 45 - 67
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bejan, A. 1993 Heat Transfer. John Wiley & Sons.Google Scholar
van der Bos, A., van der Meulen, M.-J., Driessen, T., van den Berg, M., Reinten, H., Wijshoff, H., Versluis, M. & Lohse, D. 2014 Velocity profile inside piezoacoustic inkjet droplets in flight: comparison between experiment and numerical simulation. Phys. Rev. Appl. 1, 014004.Google Scholar
Cazabat, A.-M. & Guéna, G. 2010 Evaporation of macroscopic sessile droplets. Soft Matt. 6, 2591–2612.CrossRefGoogle Scholar
Crittenden, E. D. Jr & Hixson, A. N. 1954 Extraction of hydrogen chloride from aqueous solutions. Ind. Engng Chem. 46, 265–274.CrossRefGoogle Scholar
Dehaeck, S., Rednikov, A. & Colinet, P. 2014 Vapor-based interferometric measurement of local evaporation rate and interfacial temperature of evaporating droplets. Langmuir 30, 2002–2008.CrossRefGoogle ScholarPubMed
Demond, A. H. & Lindner, A. S. 1993 Estimation of interfacial tension between organic liquids and water. Environ. Sci. Technol. 27, 2318–2331.CrossRefGoogle Scholar
Dietrich, E., Kooij, E. S., Zhang, X., Zandvliet, H. J. W. & Lohse, D. 2015 Stick–jump mode in surface droplet dissolution. Langmuir 31, 4696–4703.CrossRefGoogle ScholarPubMed
Enríquez, O. R., Sun, C., Lohse, D., Prosperetti, A. & van der Meer, D. 2014 The quasi-static growth of bubbles. J. Fluid Mech. 741, R1.CrossRefGoogle Scholar
Epstein, P. S. & Plesset, M. S. 1950 On the stability of gas bubbles in liquid–gas solutions. J. Chem. Phys. 18, 1505–1509.CrossRefGoogle Scholar
Erbil, H. Y. 2012 Evaporation of pure liquid sessile and spherical suspended drops: a review. Adv. Colloid Interface Sci. 170, 67–86.CrossRefGoogle ScholarPubMed
Fujii, T. 1963 Theory of the steady laminar natural convection above a horizontal line heat source and a point heat source. Intl J. Heat Mass Transfer 6, 597–606.CrossRefGoogle Scholar
Gelderblom, H., Marín, Á. G., Nair, H., van Houselt, A., Lefferts, L., Snoeijer, J. H. & Lohse, D. 2011 How water droplets evaporate on a superhydrophobic substrate. Phys. Rev. E 83, 026306.Google ScholarPubMed
Guéna, G., Poulard, C. & Cazabat, A.-M. 2006 The leading edge of evaporating droplets. J. Colloid Interface Sci. 312, 164–171.CrossRefGoogle Scholar
Hao, L. & Leaist, D. G. 1996 Binary mutual diffusion coefficients of aqueous alcohols. Methanol to 1-heptanol. J. Chem. Engng Data 41, 210–213.CrossRefGoogle Scholar
Høiland, H. & Vikingstad, E. 1976 Partial molal volumes and additivity of group partial molal volumes of alcohols in aqueous solution at $25\,^{\circ }\text{C}$ and $35\,^{\circ }\text{C}$ . Acta Chem. Scand. A 30, 182–186.CrossRefGoogle Scholar
Hu, H. & Larson, R. G. 2002 Evaporation of a sessile droplet on a substrate. J. Phys. Chem. B 106, 1334–1344.CrossRefGoogle Scholar
Karpitschka, S.2012 Dynamics of liquid interfaces with compositional gradients. PhD thesis, Universität Potsdam.Google Scholar
Kelly-Zion, P. L., Batra, J. & Pursell, C. J. 2013a Correlation for the convective and diffusive evaporation of a sessile drop. Intl J. Heat Mass Transfer 64, 278–285.CrossRefGoogle Scholar
Kelly-Zion, P. L., Pursell, C. J., Hasbamrer, N., Cardozo, B., Gaughan, K. & Nickels, K. 2013b Vapor distribution above an evaporating sessile drop. Intl J. Heat Mass Transfer 65, 165–172.CrossRefGoogle Scholar
Kinoshita, K., Ishikawa, H. & Shinoda, K. 1958 Solubility of alcohols in water determined the surface tension measurements. Bull. Chem. Soc. Japan 31, 1081–1082.CrossRefGoogle Scholar
Kostarev, K., Zuev, A. & Viviani, A. 2004 Oscillatory Marangoni convection around the air bubble in a vertical surfactant stratification. C. R. Méc. 332, 1–7.CrossRefGoogle Scholar
Lohse, D. & Zhang, X. 2015 Surface nanobubbles and nanodroplets. Rev. Mod. Phys. 87, 981–1035.CrossRefGoogle Scholar
Peñas López, P., Parrales, M. A. & Rodríguez-Rodríguez, J. 2015 Dissolution of a spherical cap bubble adhered to a flat surface in air-saturated water. J. Fluid Mech. 775, 53–76.CrossRefGoogle Scholar
Picknett, R. G. & Bexon, R. 1977 The evaporation of sessile or pendant drops in still air. J. Colloid Interface Sci. 61, 336–350.CrossRefGoogle Scholar
Popov, Y. O. 2005 Evaporative deposition patterns: spatial dimensions of the deposit. Phys. Rev. E 71, 036313.CrossRefGoogle ScholarPubMed
Raffel, M., Willert, C. E., Wereley, S. T. & Kompenhans, J. 2007 Particle Image Velocimetry. Springer.CrossRefGoogle Scholar
Romero, C. M., Suárez, A. F. & Jiménez, E. 2007 Effect of temperature on the volumetric properties of aliphatic alcohols in dilute aqueous solutions. Rev. Colomb. Quím. 36, 377–386.Google Scholar
Settles, G. S. 2001 Schlieren and Shadowgraph Techniques. Springer.CrossRefGoogle Scholar
Shahidzadeh-Bonn, N., Rafaï, S., Azouni, A. & Bonn, D. 2006 Evaporating droplets. J. Fluid Mech. 549, 307–313.CrossRefGoogle Scholar
Somasundaram, S., Anand, T. N. C. & Bakshi, S. 2015 Evaporation-induced flow around a pendant droplet and its influence on evaporation. Phys. Fluids 27, 112105.CrossRefGoogle Scholar
Stauber, J. M., Wilson, S. K. & Duffy, B. R. 2015a Evaporation of droplets on strongly hydrophobic substrates. Langmuir 31, 3653–3660.CrossRefGoogle ScholarPubMed
Stauber, J. M., Wilson, S. K., Duffy, B. R. & Sefiane, K. 2014 On the lifetimes of evaporating droplets. J. Fluid Mech. 744, R2.CrossRefGoogle Scholar
Stauber, J. M., Wilson, S. K., Duffy, B. R. & Sefiane, K. 2015b On the lifetimes of evaporating droplets with related initial and receding contact angles. Phys. Fluids 27, 122101.CrossRefGoogle Scholar
Stephenson, R., Stuart, J. & Tabak, M. 1984 Mutual solubility of water and aliphatic alcohols. J. Chem. Engng Data 29, 287–290.CrossRefGoogle Scholar
Vázquez, P. A., Pérez, A. T. & Castellanos, A. 1996 Thermal and electrohydrodynamic plumes: a comparative study. Phys. Fluids 8, 2091–2096.CrossRefGoogle Scholar
Yalkowsky, S. H., He, Y. & Jain, P. 2010 Handbook of Aqueous Solubility Data, 2nd edn. Taylor & Francis.Google Scholar
Zhang, X., Wang, J., Bao, L., Dietrich, E., van der Veen, R. C. A., Peng, S., Friend, J., Zandvliet, H. J. W., Yeo, L. & Lohse, D. 2015 Mixed mode of dissolving immersed nanodroplets at a solid–water interface. Soft Matt. 11, 1889–1900.CrossRefGoogle Scholar

Dietrich et al. supplementary movie

Movie of a 1-hexanol droplet (initial equivalent radius 0.7 mm) dissolving in clean water. The droplet dissolves in the so called stick-jump mode.

Download Dietrich et al. supplementary movie(Video)
Video 3.1 MB

Dietrich et al. supplementary movie

Short outtake of a μPIV measurement, visualizing the convective flow around a dissolving 1-pentanol droplet.

Download Dietrich et al. supplementary movie(Video)
Video 9.9 MB