Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-24T14:47:25.776Z Has data issue: false hasContentIssue false
  • English
  • Français

Rheology of a suspension of conducting particles in a magnetic field

Published online by Cambridge University Press:  17 May 2019

V. Kumaran Open the ORCID record for V. Kumaran [Opens in a new window]
V. Kumaran*
Affiliation:
Department of Chemical Engineering, Indian Institute of Science, Bangalore 560 012, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

When a suspension of conducting particles is sheared in a magnetic field, the fluid vorticity causes particle rotation. Eddy currents are induced in a conductor rotating in a magnetic field, resulting in magnetic moment, and a magnetic torque due to the external field. In the absence of inertia, the angular velocity of a particle is determined from the condition that the sum of the hydrodynamic and magnetic torques is zero. When the particle angular velocity is different from the fluid rotation rate, the torque exerted by the particles on the fluid results in an antisymmetric particle stress. The stress is of the form 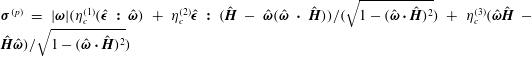$\unicode[STIX]{x1D748}^{(p)}=|\unicode[STIX]{x1D74E}|(\unicode[STIX]{x1D702}_{c}^{(1)}(\hat{\unicode[STIX]{x1D750}}\boldsymbol{ : }\hat{\unicode[STIX]{x1D74E}})+\unicode[STIX]{x1D702}_{c}^{(2)}\hat{\unicode[STIX]{x1D750}}\boldsymbol{ : }(\hat{\boldsymbol{H}}-\hat{\unicode[STIX]{x1D74E}}(\hat{\unicode[STIX]{x1D74E}}\boldsymbol{\cdot }\hat{\boldsymbol{H}}))/(\sqrt{1-(\hat{\unicode[STIX]{x1D74E}}\boldsymbol{\cdot }\hat{\boldsymbol{H}})^{2}})+\unicode[STIX]{x1D702}_{c}^{(3)}(\hat{\unicode[STIX]{x1D74E}}\hat{\boldsymbol{H}}-\hat{\boldsymbol{H}}\hat{\unicode[STIX]{x1D74E}})/\sqrt{1-(\hat{\unicode[STIX]{x1D74E}}\boldsymbol{\cdot }\hat{\boldsymbol{H}})^{2}})$, where $\unicode[STIX]{x1D74E}$ is the fluid vorticity at the centre of the particle, 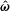$\hat{\unicode[STIX]{x1D74E}}$ and 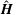$\hat{\boldsymbol{H}}$ are the unit vectors in the direction of the fluid vorticity and the magnetic field, 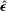$\hat{\unicode[STIX]{x1D750}}$ is the third order Levi-Civita antisymmetric tensor and 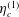$\unicode[STIX]{x1D702}_{c}^{(1)}$, $\unicode[STIX]{x1D702}_{c}^{(2)}$ and $\unicode[STIX]{x1D702}_{c}^{(3)}$ are called the first, second and third couple stress coefficients. The stress proportional to 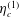$\unicode[STIX]{x1D702}_{c}^{(1)}$ is in the plane perpendicular to 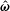$\hat{\unicode[STIX]{x1D74E}}$, that proportional to 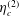$\unicode[STIX]{x1D702}_{c}^{(2)}$ is in the plane perpendicular to the unit normal to 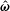$\hat{\unicode[STIX]{x1D74E}}$ in the 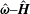$\hat{\unicode[STIX]{x1D74E}}{-}\hat{\boldsymbol{H}}$ plane, and that proportional to 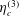$\unicode[STIX]{x1D702}_{c}^{(3)}$ is in the 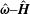$\hat{\unicode[STIX]{x1D74E}}{-}\hat{\boldsymbol{H}}$ plane. A relation $\unicode[STIX]{x1D702}_{c}^{(2)}=-(\hat{\unicode[STIX]{x1D74E}}\boldsymbol{\cdot }\hat{\boldsymbol{H}}\unicode[STIX]{x1D702}_{c}^{(1)}/\sqrt{1-(\hat{\unicode[STIX]{x1D74E}}\boldsymbol{\cdot }\hat{\boldsymbol{H}})^{2}})$ results from the condition that the component of the eddy current torque along the magnetic field is zero. 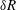The couple stress coefficients are obtained for two geometries, a uniform spherical particle of radius $R$ and a thin spherical shell of radius $R$ and thickness $\unicode[STIX]{x1D6FF}R$ with 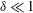$\unicode[STIX]{x1D6FF}\ll 1$, in the dilute (non-interacting) limit in the absence of fluid inertia. These couple stress coefficients are functions of two dimensionless parameters, 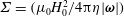$\unicode[STIX]{x1D6F4}=(\unicode[STIX]{x1D707}_{0}H_{0}^{2}/4\unicode[STIX]{x03C0}\unicode[STIX]{x1D702}|\unicode[STIX]{x1D74E}|)$, the ratio of the characteristic magnetic and hydrodynamic torques, and 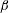$\unicode[STIX]{x1D6FD}$, the product of the vorticity and current relaxation time. Here 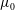$\unicode[STIX]{x1D707}_{0}$ is the magnetic permeability, 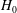$H_{0}$ is the magnetic field and 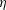$\unicode[STIX]{x1D702}$ is the fluid viscosity. The parameter 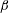$\unicode[STIX]{x1D6FD}$ has the form $\unicode[STIX]{x1D6FD}_{p}=(|\unicode[STIX]{x1D74E}|\unicode[STIX]{x1D707}_{0}R^{2}/2\unicode[STIX]{x1D71A})$ for a uniform particle and 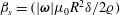$\unicode[STIX]{x1D6FD}_{s}=(|\unicode[STIX]{x1D74E}|\unicode[STIX]{x1D707}_{0}R^{2}\unicode[STIX]{x1D6FF}/2\unicode[STIX]{x1D71A})$ for a thin shell, where 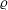$\unicode[STIX]{x1D71A}$ is the electrical resistivity. Scaled couple stress coefficients are defined, $\unicode[STIX]{x1D702}_{1}^{\ast }=(\unicode[STIX]{x1D702}_{c}^{(1)}/((3\unicode[STIX]{x1D702}\unicode[STIX]{x1D719}/2)(1-(\hat{\unicode[STIX]{x1D74E}}\boldsymbol{\cdot }\hat{\boldsymbol{H}})^{2})))$ and 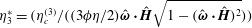$\unicode[STIX]{x1D702}_{3}^{\ast }=(\unicode[STIX]{x1D702}_{c}^{(3)}/((3\unicode[STIX]{x1D719}\unicode[STIX]{x1D702}/2)\hat{\unicode[STIX]{x1D74E}}\boldsymbol{\cdot }\hat{\boldsymbol{H}}\sqrt{1-(\hat{\unicode[STIX]{x1D74E}}\boldsymbol{\cdot }\hat{\boldsymbol{H}})^{2}}))$, which are independent of the fluid viscosity and the particle volume fraction, and which do not depend on 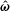$\hat{\unicode[STIX]{x1D74E}}$ and $\hat{\boldsymbol{H}}$ in the limits $\unicode[STIX]{x1D6F4}\ll 1$ and $\unicode[STIX]{x1D6F4}\gg 1$. Here, $\unicode[STIX]{x1D719}$ is the volume fraction of the particles. 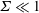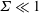Asymptotic analysis is used to determine the couple stress coefficients in the limits $\unicode[STIX]{x1D6F4}\ll 1$ and $\unicode[STIX]{x1D6F4}\gg 1$, and a numerical solution procedure is formulated for $\unicode[STIX]{x1D6F4}\sim 1$. For 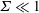$\unicode[STIX]{x1D6F4}\ll 1$, the particle angular velocity is aligned close to the fluid vorticity, and the scaled couple stress coefficients are 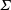$\unicode[STIX]{x1D6F4}$ times a function of $\unicode[STIX]{x1D6FD}$. For 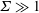$\unicode[STIX]{x1D6F4}\gg 1$, the particle angular velocity is aligned close to the magnetic field, $\unicode[STIX]{x1D702}_{1}^{\ast }\rightarrow 1$ and $\unicode[STIX]{x1D702}_{3}^{\ast }\propto \unicode[STIX]{x1D6F4}^{-1}$. When the magnetic field is perpendicular to the fluid vorticity, 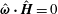$\hat{\unicode[STIX]{x1D74E}}\boldsymbol{\cdot }\hat{\boldsymbol{H}}=0$, the particle angular velocity is aligned along the vorticity, and only the first couple stress coefficient is non-zero. For high 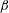$\unicode[STIX]{x1D6FD}$, there are multiple solutions for the couple stress coefficient. Multiple steady states are also observed for a near perpendicular magnetic field, 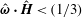$\hat{\unicode[STIX]{x1D74E}}\boldsymbol{\cdot }\hat{\boldsymbol{H}}<(1/3)$, for a reason different from that for a perpendicular magnetic field. Asymptotic analysis is used to explain the existence of multiple steady states in both cases.

JFM classification

MHD and Electrohydrodynamics: Magnetic fluids Non-Newtonian Flows: Rheology Complex Fluids: Suspensions
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 871 , 25 July 2019 , pp. 139 - 185
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anupama, A. V., Kumaran, V. & Sahoo, B. 2018 Magnetorheological fluids containing rod-shaped lithium–zinc ferrite particles: the steady-state shear response. Soft Matt. 14, 5407–5419.Google Scholar
Batchelor, G. K. 1970 The stress in a suspension of force-free particles. J. Fluid Mech. 41, 545–570.Google Scholar
Batchelor, G. K. 1972 Sedimentation in a dilute dispersion of spheres. J. Fluid Mech. 52, 245–268.Google Scholar
Bolcato, R., Etay, J., Fautrelle, Y. & Moffatt, H. K. 1993 Electromagnetic billiards. Phys. Fluids A 5, 1852–1853.Google Scholar
Bossis, G., Lemaire, E., Volkova, O. & Clercx, H. 1997 Yield stress in magnetorheological and electrorheological fluids: a comparison between microscopic and macroscopic structural models. J. Rheol. 41, 687–704.Google Scholar
Caflisch, R. E. & Luke, J. H. C. 1985 Variance in the sedimentation speed of a suspension. Phys. Fluids 28, 759–760.Google Scholar
Guazzelli, E. & Hinch, J. 2011 Fluctuations and instability in sedimentation. Annu. Rev. Fluid Mech. 43 (1), 97–116.Google Scholar
Habib, S., Holz, D. E., Kheyfets, A., Matzner, R. A., Miller, W. A. & Tolman, B. W. 1994 Spin dynamics of the LAGEOS satellite in support of a measurement of the Earth’s gravitomagnetism. Phys. Rev. D 50, 6068–6079.Google Scholar
Halverson, R. P. & Cohen, H. 1964 Torque on a spinning hollow sphere in a uniform magnetic field. IEEE Trans. Aerosp. Navig. Electron. ANE‐11, 118–122.Google Scholar
Hinch, E. J. 1977 An averaged equation approach to particle interactions in a fluid suspension. J. Fluid Mech. 83, 695–720.Google Scholar
Hjortstam, O., Isberg, P., Söderholm, S. & Dai, H. 2004 Can we achieve ultra-low resistivity in carbon nanotube-based metal composites? Appl. Phys. A 78, 1175–1179.Google Scholar
Ho, B. P. & Leal, L. G. 1974 Inertial migration of rigid spheres in two dimensional unidirectional flows. J. Fluid Mech. 65, 365–400.Google Scholar
Hogg, A. J. 1994 The inertial migration of non-neutrally buoyant spherical particles in two-dimensional shear flows. J. Fluid Mech. 272, 285–318.Google Scholar
Holdeman, L. B.1975 Magnetic torque on a rotating superconducting sphere. NASA Tech. Rep. TR R-443. National Aeronautics and Space Administration.Google Scholar
Jansons, K. M. 1983 Determination of the constitutive equations for a magnetic fluid. J. Fluid Mech. 137, 187–216.Google Scholar
Klingenberg, D. J. 2001 Magnetorheology: applications and challenges. AIChE J. 47, 246–249.Google Scholar
Klingenberg, D. J. & Zukoski, C. F. 1990 Studies on the steady-shear behavior of electrorheological suspensions. Langmuir 6, 15–24.Google Scholar
Koch, D. L. & Shaqfeh, E. S. G. 1991 Screening in sedimenting suspensions. J. Fluid Mech. 224, 275–303.Google Scholar
Kuzhir, P., Lopez-Lopez, M. T. & Bossis, G. 2009 Magnetorheology of fiber suspensions. II. Theory. J. Rheol. 53, 127–151.Google Scholar
Landau, L. D., Lifshitz, E. M. & Pitaevskii, L. P. 2014 Electrodynamics of Continuous Media. Butterworth-Heinemann.Google Scholar
Lopez-Lopez, M. T., Kuzhir, P. & Bossis, G. 2009 Magnetorheology of fiber suspensions. I. Experimental. J. Rheol. 53, 115–126.Google Scholar
Mindlin, R. D. & Tiersten, H. F. 1962 Effects of couple-stresses in linear elasticity. Arch. Rat. Mech. Anal. 11, 415–448.Google Scholar
Moffat, H. K. 1990 On the behaviour of a suspension of conducting particles subjected to a time-periodic magnetic field. J. Fluid Mech. 218, 509–529.Google Scholar
Saffman, P. G. 1965 The lift on a small sphere in slow shear flow. J. Fluid Mech. 22, 384–400.Google Scholar
Schafer, R. & Heiden, C. 1978 A study of the flux density distribution in type II superconductors rotating in a magnetic field. J. Low Temp. Phys. 30, 357–387.Google Scholar
Schumacher, K. R., Riley, J. J. & Finlayson, B. A. 2008 Homogeneous turbulence in ferrofluids with a steady magnetic field. J. Fluid Mech. 599, 1–28.Google Scholar
Seric, I., Afkhami, S. & Kondic, L. 2014 Interfacial instability of thin ferrofluid films under a magnetic field. J. Fluid Mech. 755, R1–1–R1–12.Google Scholar
Sherman, S. G., Becnel, A. C. & Wereley, N. M. 2015 Relating mason number to bingham number in magnetorheological fluids. J. Magn. Magn. Mater. 380, 98–104.Google Scholar
Stokes, V. K. 1966 Couple stresses in fluids. Phys. Fluids 9, 1709–1715.Google Scholar
Truesdell, C. & Toupin, R. A. 1960 The classical field theories. In Handbuch der Physik (ed. Flugge, S.), pp. 545–609. Springer.Google Scholar
Vagberg, D. & Tighe, B. P. 2017 On the apparent yield stress in non-Brownian magnetorheological fluids. Soft Matt. 13, 7207–7221.Google Scholar
de Vicente, J., Klingenberg, D. J. & Hidalgo-Alvarez, R. 2011 Magnetorheological fluids: a review. Soft Matt. 7, 3701–3710.Google Scholar
de Vicente, J., Segovia-Gutierrez, J. P., Andablo-Reyes, E., Vereda, F. & Hidalgo-Alvarez, R. 2009 Dynamic rheology of sphere- and rod-based magnetorheological fluids. J. Chem. Phys. 131 (19), 194902.Google Scholar
Zheng, L., Zheng, H., Huo, D., Wu, F., Shao, L., Zheng, Y., Zheng, X., Qui, X., Liu, Y. & Zhang, Y. 2018 N-doped graphene-based copper nanocomposite with ultralow electrical resistivity and high thermal conductivity. Sci. Rep. 8, 9248.Google Scholar