Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-12-01T01:31:15.328Z Has data issue: false hasContentIssue false
  • English
  • Français

Related self-similar statistics of the turbulent/non-turbulent interface and the turbulence dissipation

Published online by Cambridge University Press:  25 May 2017

Y. Zhou  and
J. C. Vassilicos Open the ORCID record for J. C. Vassilicos [Opens in a new window]
Y. Zhou
Affiliation:
Turbulence, Mixing and Flow Control Group, Department of Aeronautics, Imperial College London, London SW7 2AZ, UK
J. C. Vassilicos*
Affiliation:
Turbulence, Mixing and Flow Control Group, Department of Aeronautics, Imperial College London, London SW7 2AZ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

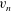The scalings of the local entrainment velocity $v_{n}$ of the turbulent/non-turbulent interface and of the turbulence dissipation rate are closely related to each other in an axisymmetric and self-similar turbulent wake. The turbulence dissipation scaling implied by the Kolmogorov equilibrium cascade phenomenology is consistent with a Kolmogorov scaling of 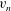$v_{n}$ whereas the non-equilibrium dissipation scaling reported for various turbulent flows in Vassilicos (Annu. Rev. Fluid Mech., vol. 47, 2015, pp. 95–114), Dairay et al. (J. Fluid Mech., vol. 781, 2015, pp. 166–195), Goto & Vassilicos (Phys. Lett. A, vol. 379 (16), 2015, pp. 1144–1148) and Obligado et al. (Phys. Rev. Fluids, vol. 1 (4), 2016, 044409) is consistent with a different scaling of $v_{n}$. We present results from a direct numerical simulation of a spatially developing axisymmetric and self-similar turbulent wake which supports this conclusion and the assumptions that it is based on.

JFM classification

Turbulent Flows: Turbulence simulation Turbulent Flows: Turbulence theory Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 821 , 25 June 2017 , pp. 440 - 457
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Attili, A., Cristancho, J. C. & Bisetti, F. 2014 Statistics of the turbulent/non-turbulent interface in a spatially developing mixing layer. J. Turbul. 15 (9), 555–568.Google Scholar
Bisset, D. K., Hunt, J. C. R. & Rogers, M. M. 2002 The turbulent/non-turbulent interface bounding a far wake. J. Fluid Mech. 451, 383–410.CrossRefGoogle Scholar
Borrell, G. & Jiménez, J. 2016 Properties of the turbulent/non-turbulent interface in boundary layers. J. Fluid Mech. 801, 554–596.CrossRefGoogle Scholar
Chauhan, K., Philip, J., de Silva, C. M., Hutchins, N. & Marusic, I. 2014 The turbulent/non-turbulent interface and entrainment in a boundary layer. J. Fluid Mech. 742, 119–151.Google Scholar
Corrsin, S. & Kistler, A. L. 1955 Free-stream boundaries of turbulent flows. NACA Tech. Rep. 1224, 1033–1064.Google Scholar
Dairay, T., Obligado, M. & Vassilicos, J. C. 2015 Non-equilibrium scaling laws in axisymmetric turbulent wakes. J. Fluid Mech. 781, 166–195.Google Scholar
Dairay, T. & Vassilicos, J. C. 2016 Direct numerical simulation of a turbulent wake: the non-equilibrium dissipation law. Intl J. Heat Fluid Flow 62, 68–74.Google Scholar
Gampert, M., Kleinheinz, K., Peters, N. & Pitsch, H. 2014 Experimental and numerical study of the scalar turbulent/non-turbulent interface layer in a jet flow. Flow Turbul. Combust. 92 (1–2), 429–449.Google Scholar
George, W. K. 1989 The self-preservation of turbulent flows and its relation to initial conditions and coherent structures. In Advances in Turbulence (ed. George, W. K. & Arndt, R.), pp. 39–73. Hemisphere.Google Scholar
Goto, S. & Vassilicos, J. C. 2015 Energy dissipation and flux laws for unsteady turbulence. Phys. Lett. A 379 (16), 1144–1148.Google Scholar
Goto, S. & Vassilicos, J. C. 2016 Usteady turbulence cascades. Phys. Rev. E 94 (5), 053108.Google Scholar
Holzner, M. & Lüthi, B. 2011 Laminar superlayer at the turbulence boundary. Phys. Rev. Lett. 106 (13), 134503.CrossRefGoogle ScholarPubMed
LaRue, J. C. & Libby, P. A. 1976 Statistical properties of the interface in the turbulent wake of a heated cylinder. Phys. Fluids 19 (12), 1864–1875.Google Scholar
Mandelbrot, B. B. 1982 The Fractal Geometry of Nature. W. H. Freeman and Company.Google Scholar
Mistry, D., Philip, J., Dawson, J. R. & Marusic, I. 2016 Entrainment at multi-scales across the turbulent/non-turbulent interface in an axisymmetric jet. J. Fluid Mech. 802, 690–725.Google Scholar
Nedić, J., Vassilicos, J. C. & Ganapathisubramani, B. 2013 Axisymmetric turbulent wakes with new nonequilibrium similarity scalings. Phys. Rev. Lett. 111 (14), 144503.Google Scholar
Obligado, M., Dairay, T. & Vassilicos, J. C. 2016 Nonequilibrium scalings of turbulent wakes. Phys. Rev. Fluids 1 (4), 044409.CrossRefGoogle Scholar
Parnaudeau, P., Carlier, J., Heitz, D. & Lamballais, E. 2008 Experimental and numerical studies of the flow over a circular cylinder at Reynolds number 3900. Phys. Fluids 20 (8), 085101.CrossRefGoogle Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.Google Scholar
Redford, J. A., Castro, I. P. & Coleman, G. N. 2012 On the universality of turbulent axisymmetric wakes. J. Fluid Mech. 710, 419–452.Google Scholar
da Silva, C. B., Hunt, J. C. R., Eames, I. & Westerweel, J. 2014 Interfacial layers between regions of different turbulence intensity. Annu. Rev. Fluid Mech. 46, 567–590.Google Scholar
Sreenivasan, K. R., Ramshankar, R. & Meneveau, C. H. 1989 Mixing, entrainment and fractal dimensions of surfaces in turbulent flows. Proc. R. Soc. Lond. 421, 79–108.Google Scholar
Taveira, R. R. & da Silva, C. B. 2014 Characteristics of the viscous superlayer in shear free turbulence and in planar turbulent jets. Phys. Fluids 26, 021702.Google Scholar
Tennekes, H. & Lumley, J. L. 1972 A First Course in Turbulence. MIT Press.Google Scholar
Townsend, A. A. 1976 The Structure of Turbulent Shear Flow. Cambridge University Press.Google Scholar
Valente, P. C. & Vassilicos, J. C. 2012 Universal dissipation scaling for nonequilibrium turbulence. Phys. Rev. Lett. 108 (21), 214503.Google Scholar
Valente, P. C. & Vassilicos, J. C. 2014 The non-equilibrium region of grid-generated decaying turbulence. J. Fluid Mech. 744, 5–37.Google Scholar
Vassilicos, J. C. 2015 Dissipation in turbulent flows. Annu. Rev. Fluid Mech. 47, 95–114.CrossRefGoogle Scholar
Watanabe, T., Riley, J. J., de Bruyn Kops, S. M., Diamessis, P. J. & Zhou, Q. 2016 Turbulent/non-turbulent interfaces in wakes in stably-stratified fluids. J. Fluid Mech. 797, 567–590.Google Scholar
Watanabe, T., Sakai, Y., Nagata, K., Ito, Y. & Hayase, T. 2014 Enstrophy and passive scalar transport near the turbulent/non-turbulent interface in a turbulent planar jet flow. Phys. Fluids 26 (10), 105103.Google Scholar
Watanabe, T., Sakai, Y., Nagata, K., Ito, Y. & Hayase, T. 2015 Turbulent mixing of passive scalar near turbulent and non-turbulent interface in mixing layers. Phys. Fluids 27 (8), 085109.Google Scholar
Westerweel, J., Fukushima, C., Pedersen, J. M. & Hunt, J. C. R. 2009 Momentum and scalar transport at the turbulent/non-turbulent interface of a jet. J. Fluid Mech. 631, 199–230.Google Scholar