Reaction induced interfacial instability of miscible fluids in a channel

Abstract

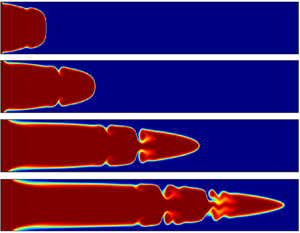
When a less viscous miscible fluid displaces a more-viscous one under a pressure-driven channel flow, unstable Kelvin–Helmholtz (K–H)-type billows are formed at the miscible interface. In this paper, we investigate whether such instability can be induced by a simple ($\textbf {A}+ \textbf {B} \rightarrow \textbf {C}$)-type chemical reaction. Here a miscible solution of one reactant $\textbf {A}$ is displacing another isoviscous reactant $\textbf {B}$ and producing a more-viscous product $\textbf {C}$ at the reactive front. It is found that because of a local increase in viscosity gradient due to the formation of more-viscous product $\textbf {C}$, K–H-type billows are formed at the $\textbf {A}$–$\textbf {C}$ interface. The changes in dynamical properties of such billows are examined by varying the governing parameters such as the mobility ratio $R_{c}$, Damköhler number $Da$, Péclet number $Pe$ and Reynolds number $Re$. Interestingly, we have found that even at high reaction rates (sufficiently large $Da$) for $R_{c}=1$, the interface remains stable and for larger values of $R_{c} (=3, 5)$ the K–H billows are observed. It is also noticed that a laminar horseshoe-type vortex develops near the wall at the channel inlet where the less-viscous reactant pushes the more-viscous product. We have computed numerically the onset time ($t_{on}$) of instability to understand the early-stage developments of the K–H billows. For different values of $Da$, we have shown the unstable and stable time zones in the ($t_{on}$–$R_{c}$) space. The bipartite ($t_{on}$–$R_{c}$) space also depicts the critical ($Da$-, $Pe$- and $Re$-dependent) $R_{c}$ value for which instability can be triggered in a finite desirable time. The delay in the onset of instability is observed with increasing $Pe$. Further it is shown that $t_{on}$ can be linearly scaled with $Pe$ to have a modified onset time ($t^{*}_{on}$), which establishes a proportionate dynamics with respect to $Pe$ in the early stages of the instability. Moreover, a reverse dependency of onset on lower $R_{c}$ values for higher Reynolds numbers is observed.