Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-28T07:42:27.278Z Has data issue: false hasContentIssue false
  • English
  • Français

Radial viscous fingering induced by an infinitely fast chemical reaction

Published online by Cambridge University Press:  20 July 2022

Priya Verma Open the ORCID record for Priya Verma [Opens in a new window] ,
Vandita Sharma Open the ORCID record for Vandita Sharma [Opens in a new window]  and
Manoranjan Mishra Open the ORCID record for Manoranjan Mishra [Opens in a new window]
Priya Verma
Affiliation:
Department of Mathematics, Indian Institute of Technology Ropar, 140001 Rupnagar, India
Vandita Sharma
Affiliation:
Department of Mathematics, Indian Institute of Technology Ropar, 140001 Rupnagar, India
Manoranjan Mishra*
Affiliation:
Department of Mathematics, Indian Institute of Technology Ropar, 140001 Rupnagar, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

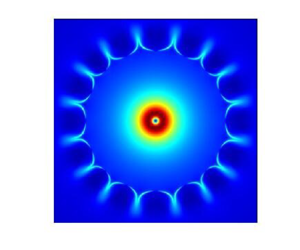
A numerical insight into the radial displacement of two viscously stable reactants undergoing an infinitely fast chemical reaction is obtained. This work broadens the numerical knowledge about the interaction between chemical reaction and hydrodynamic instability. A suitable transformation is utilised to deal with an infinite dimensionless parameter and reduce computational cost. Viscous fingering instability originates when the product has a different viscosity than the reactants. We calculate the onset time $t_{on}$ when the instability begins to appear for different reactants by varying the log mobility ratio $R_c$ and the Péclet number $Pe$. Based on $t_{on}$, we divide the time domain into stable and unstable zones with respect to instability. This helps to characterise reactants so as to have a flow with/without instability. For a fixed $Pe$ and $\vert R_c \vert$, it is found that $t_{on}$ is early for $R_c > 0$ in contrast to the result for rectilinear displacement. This results in a wider stable zone for $R_c < 0$. Interestingly, it is found that the stable zone can be made independent of $Pe$ by using a careful rescaling and we obtain the dependence of the onset time of instability on $R_c$ and $Pe$ as $t_{on} \propto (\vert R_c\vert Pe^{\beta _1}-\beta _2)^{\beta _3}$ where the constant of proportionality and $\beta _i$, $i=1,2,3$, depend upon the sign of $R_c$. In the unstable zone, we find that the length of the fingers depends upon the sign of $R_c$, which is not observed for any radial displacement of reactants undergoing a slow or moderately fast chemical reaction.

JFM classification

Interfacial Flows (free surface): Fingering instability Low-Reynolds-number flows: Hele-Shaw flows Low-Reynolds-number flows: Porous media
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 945 , 25 August 2022 , A19
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bogdan, M.J. & Savin, T. 2018 Fingering instabilities in tissue invasion: an active fluid model. R. S. Open Sci. 5 (12), 181579.CrossRefGoogle Scholar
Brau, F. & De Wit, A. 2020 Influence of rectilinear vs radial advection on the yield of $A+B \rightarrow C$ reaction fronts: a comparison. J. Chem. Phys. 152 (5), 054716.CrossRefGoogle Scholar
Burden, R.L., Faires, J.D. & Burden, A.M. 2015 Numerical Analysis. Cengage Learning.Google Scholar
Cardoso, E.M.L., Arregger, A.L., Tumilasci, O.R., Elbert, A. & Contreras, L.N. 2009 Assessment of salivary urea as a less invasive alternative to serum determinations. Scand. J. Clin. Lab. Invest. 69 (3), 330–334.CrossRefGoogle ScholarPubMed
Chen, C.Y., Huang, C.W., Gadêlha, H. & Miranda, J.A. 2008 Radial viscous fingering in miscible Hele-Shaw flows: a numerical study. Phys. Rev. E 78 (1), 016306.Google ScholarPubMed
Clingan, H., Rusk, D., Smith, K. & Garcia, A.A. 2018 Viscous fingering of miscible liquids in porous and swellable media for rapid diagnostic tests. Bioengineering 5 (4), 94.CrossRefGoogle ScholarPubMed
Cook, A.W. & Riley, J.J. 1994 A subgrid model for equilibrium chemistry in turbulent flows. Phys. Fluids 6 (8), 2868–2870.CrossRefGoogle Scholar
De Wit, A. 2016 Chemo-hydrodynamic patterns in porous media. Phil. Trans. R. Soc. A: Math. Phys. Engng Sci. 374 (2078), 20150419.CrossRefGoogle ScholarPubMed
De Wit, A., Eckert, K. & Kalliadasis, S. 2012 Introduction to the focus issue: chemo-hydrodynamic patterns and instabilities. Chaos: Interdisciplinary J. Nonlinear Sci. 22 (3), 037101.CrossRefGoogle Scholar
Ding, H., Spelt, P.D.M. & Shu, C. 2007 Diffuse interface model for incompressible two-phase flows with large density ratios. J. Comput. Phys. 226 (2), 2078–2095.CrossRefGoogle Scholar
Gérard, T. & De Wit, A. 2009 Miscible viscous fingering induced by a simple $A+B {\rightarrow }C$ chemical reaction. Phys. Rev. E 79 (1), 016308.Google Scholar
Hejazi, S.H. & Azaiez, J. 2010 Non-linear interactions of dynamic reactive interfaces in porous media. Chem. Engng Sci. 65 (2), 938–949.CrossRefGoogle Scholar
Hejazi, S.H., Trevelyan, P.M.J., Azaiez, J. & De Wit, A. 2010 Viscous fingering of a miscible reactive $A+B {\rightarrow }C$ interface: a linear stability analysis. J. Fluid Mech. 652, 501–528.CrossRefGoogle Scholar
Jain, S.S., Mani, A. & Moin, P. 2020 A conservative diffuse-interface method for compressible two-phase flows. J. Comput. Phys. 418, 109606.CrossRefGoogle Scholar
Jha, B., Cueto-Felgueroso, L. & Juanes, R. 2011 a Fluid mixing from viscous fingering. Phys. Rev. Lett. 106 (19), 194502.CrossRefGoogle ScholarPubMed
Jha, B., Cueto-Felgueroso, L. & Juanes, R. 2011 b Quantifying mixing in viscously unstable porous media flows. Phys. Rev. E 84 (6), 066312.CrossRefGoogle ScholarPubMed
Jha, B., Cueto-Felgueroso, L. & Juanes, R. 2013 Synergetic fluid mixing from viscous fingering and alternating injection. Phys. Rev. Lett. 111 (14), 144501.CrossRefGoogle ScholarPubMed
Lele, S.K. 1992 Compact finite difference schemes with spectral-like resolution. J. Comput. Phys. 103 (1), 16–42.CrossRefGoogle Scholar
Liu, H.-R. & Ding, H. 2015 A diffuse-interface immersed-boundary method for two-dimensional simulation of flows with moving contact lines on curved substrates. J. Comput. Phys. 294, 484–502.CrossRefGoogle Scholar
MATLAB 2020 MATLAB Version 9.3.0.713579 (R2017b). Mathworks, Inc.Google Scholar
Michioka, T. & Komori, S. 2004 Large-eddy simulation of a turbulent reacting liquid flow. AIChE J. 50 (11), 2705–2720.CrossRefGoogle Scholar
Mishra, M., Martin, M. & De Wit, A. 2008 Differences in miscible viscous fingering of finite width slices with positive or negative log-mobility ratio. Phys. Rev. E 78 (6), 066306.CrossRefGoogle ScholarPubMed
Nagatsu, Y. & De Wit, A. 2011 Viscous fingering of a miscible reactive $A+B {\rightarrow }C$ interface for an infinitely fast chemical reaction: nonlinear simulations. Phys. Fluids 23 (4), 043103.CrossRefGoogle Scholar
Nagatsu, Y., Kondo, Y., Kato, Y. & Tada, Y. 2009 Effects of moderate Damköhler number on miscible viscous fingering involving viscosity decrease due to a chemical reaction. J. Fluid Mech. 625, 97–124.CrossRefGoogle Scholar
Nagatsu, Y., Matsuda, K., Kato, Y. & Tada, Y. 2007 Experimental study on miscible viscous fingering involving viscosity changes induced by variations in chemical species concentrations due to chemical reactions. J. Fluid Mech. 571, 475–493.CrossRefGoogle Scholar
Nagatsu, Y. & Ueda, T. 2001 Effects of reactant concentrations on reactive miscible viscous fingering. AIChE J. 47 (8), 1711–1720.CrossRefGoogle Scholar
Nagatsu, Y. & Ueda, T. 2004 Analytical study of effects of finger-growth velocity on reaction characteristics of reactive miscible viscous fingering by using a convection–diffusion–reaction model. Chem. Engng Sci. 59 (18), 3817–3826.CrossRefGoogle Scholar
Podgorski, T., Sostarecz, M.C., Zorman, S. & Belmonte, A. 2007 Fingering instabilities of a reactive micellar interface. Phys. Rev. E 76 (1), 016202.CrossRefGoogle ScholarPubMed
Riolfo, L.A., Nagatsu, Y., Iwata, S., Maes, R., Trevelyan, P.M.J. & De Wit, A. 2012 Experimental evidence of reaction-driven miscible viscous fingering. Phys. Rev. E 85 (1), 015304.CrossRefGoogle ScholarPubMed
Sett, A., Ayushman, M., Dasgupta, S. & DasGupta, S. 2018 Analysis of the distinct pattern formation of globular proteins in the presence of micro- and nanoparticles. J. Phys. Chem. B 122 (38), 8972–8984.CrossRefGoogle ScholarPubMed
Sharma, V., Nand, S., Pramanik, S., Chen, C.-Y. & Mishra, M. 2020 Control of radial miscible viscous fingering. J. Fluid Mech. 884, A16.CrossRefGoogle Scholar
Sharma, V., Pramanik, S., Chen, C.-Y. & Mishra, M. 2019 A numerical study on reaction-induced radial fingering instability. J. Fluid Mech. 862, 624–638.CrossRefGoogle Scholar
Sudarshan Kumar, K., Praveen, C. & Veerappa Gowda, G.D. 2014 A finite volume method for a two-phase multicomponent polymer flooding. J. Comput. Phys. 275, 667–695.Google Scholar
Tan, C.T. & Homsy, G.M. 1986 Stability of miscible displacements in porous media: rectilinear flow. Phys. Fluids 29 (11), 3549–3556.CrossRefGoogle Scholar
Tan, C.T. & Homsy, G.M. 1987 Stability of miscible displacements in porous media: radial source flow. Phys. Fluids 30 (5), 1239–1245.CrossRefGoogle Scholar