Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-12-01T07:20:44.990Z Has data issue: false hasContentIssue false
  • English
  • Français

Parametrically forced stably stratified flow in a three-dimensional rectangular container

Published online by Cambridge University Press:  05 August 2020

Jason Yalim Open the ORCID record for Jason Yalim [Opens in a new window] ,
Juan M. Lopez Open the ORCID record for Juan M. Lopez [Opens in a new window]  and
Bruno D. Welfert Open the ORCID record for Bruno D. Welfert [Opens in a new window]
Jason Yalim
Affiliation:
School of Mathematical and Statistical Sciences, Arizona State University, Tempe, AZ85287, USA
Juan M. Lopez*
Affiliation:
School of Mathematical and Statistical Sciences, Arizona State University, Tempe, AZ85287, USA
Bruno D. Welfert
Affiliation:
School of Mathematical and Statistical Sciences, Arizona State University, Tempe, AZ85287, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

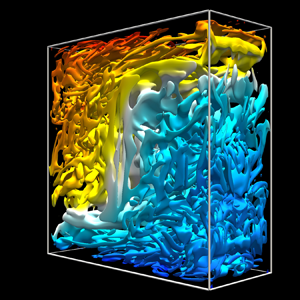
The dynamics of a stably and thermally stratified fluid-filled cavity harmonically forced in the vertical direction, resulting in a periodic gravity modulation, is studied numerically. Prior simulations in a two-dimensional cavity showed a myriad of complex dynamic behaviours near the onset of instabilities, and here we address the extent to which these persist in three dimensions. Focusing on a parameter regime where the primary subharmonic mode is resonantly driven, we demonstrate comprehensive qualitative agreement between the dynamics in two and three dimensions; the quantitative difference is due to the larger forcing amplitudes needed in three dimensions to overcome the additional viscous damping from the spanwise walls. Using a small detuning of the forcing frequency, together with a relatively large forcing amplitude, leads to a wave-breaking regime where the qualitative agreement between two and three dimensions breaks down.

JFM classification

Geophysical and Geological Flows: Internal waves Instability: Parametric instability
Type
JFM Rapids
Information
Journal of Fluid Mechanics , Volume 900 , 10 October 2020 , R3
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Benielli, D. & Sommeria, J. 1998 Excitation and breaking of internal gravity waves by parametric instability. J. Fluid Mech. 374, 117–144.CrossRefGoogle Scholar
Bouruet-Aubertot, P., Sommeria, J. & Staquet, C. 1995 Breaking of standing internal gravity waves through two-dimensional instabilities. J. Fluid Mech. 285, 265–301.CrossRefGoogle Scholar
Dauxois, T., Joubaud, S., Odier, P. & Venaille, A. 2018 Instabilities of internal gravity wave beams. Annu. Rev. Fluid Mech. 50, 1–28.CrossRefGoogle Scholar
Hunt, J. C., Wray, A. A. & Moin, P. 1988 Eddies, streams, and convergence zones in turbulent flows. In Proceedings of the Summer Program, pp. 193–208. Center for Turbulence Research.Google Scholar
Joubaud, S., Munroe, J., Odier, P. & Dauxois, T. 2012 Experimental parametric subharmonic instability in stratified fluids. Phys. Fluids 24, 041703.CrossRefGoogle Scholar
Liu, C., Wang, Y., Tang, Y. & Duan, Z. 2016 New omega vortex identification method. Sci. China Phys. Mech. Astron. 59, 684711.CrossRefGoogle Scholar
Lopez, J. M., Welfert, B. D., Wu, K. & Yalim, J. 2017 Transition to complex dynamics in the cubic lid-driven cavity. Phys. Rev. Fluids 2, 074401.CrossRefGoogle Scholar
McEwan, A. D. 1971 Degeneration of resonantly-excited standing internal gravity waves. J. Fluid Mech. 50, 431–448.CrossRefGoogle Scholar
McEwan, A. D., Mander, D. W. & Smith, R. K. 1972 Forced resonant second-order interaction between damped internal waves. J. Fluid Mech. 55, 589–608.CrossRefGoogle Scholar
McEwan, A. D. & Robinson, R. M. 1975 Parametric instability of internal gravity waves. J. Fluid Mech. 67, 667–687.CrossRefGoogle Scholar
Mercader, I., Batiste, O. & Alonso, A. 2010 An efficient spectral code for incompressible flows in cylindrical geometries. Comput. Fluids 39, 215–224.CrossRefGoogle Scholar
Staquet, C. & Sommeria, J. 2002 Internal gravity waves: from instabilities to turbulence. Annu. Rev. Fluid Mech. 34, 559–593.CrossRefGoogle Scholar
Sutherland, B. R. 2013 The wave instability pathway to turbulence. J. Fluid Mech. 724, 1–4.CrossRefGoogle Scholar
Thorpe, S. A. 1968 On standing internal gravity waves of finite amplitude. J. Fluid Mech. 32, 489–528.CrossRefGoogle Scholar
Wu, K., Welfert, B. D. & Lopez, J. M. 2018 Complex dynamics in a stratified lid-driven square cavity flow. J. Fluid Mech. 855, 43–66.CrossRefGoogle Scholar
Yalim, J., Lopez, J. M. & Welfert, B. D. 2018 Vertically forced stably stratified cavity flow: instabilities of the basic state. J. Fluid Mech. 851, R6.CrossRefGoogle Scholar
Yalim, J., Welfert, B., Lopez, J. & Wu, K. 2017 V0066: resonant collapse in a harmonically forced stratified cavity. In American Physical Society Division of Fluid Dynamics 2017 Gallery of Fluid Motion, doi:10.1103/APS.DFD.2017.GFM.V0066. American Physical Society.CrossRefGoogle Scholar
Yalim, J., Welfert, B. D. & Lopez, J. M. 2019 a Modal reduction of a parametrically forced confined viscous flow. Phys. Rev. Fluids 4, 103903.CrossRefGoogle Scholar
Yalim, J., Welfert, B. D. & Lopez, J. M. 2019 b Parametrically forced stably stratified cavity flow: complicated nonlinear dynamics near the onset of instability. J. Fluid Mech. 871, 1067–1096.CrossRefGoogle Scholar

Yalim et al. supplementary movie 1

Animations over two forcing periods of the spanwise component of vorticity, the normalized Q-criterion, and isotherms for the four subharmonic limit cycles shown in figure 4.
Download Yalim et al. supplementary movie 1(Video)
Video 9.7 MB

Yalim et al. supplementary movie 2

Animation of the normalized Q-criterion for a quasiperiodic state in figure 5, strobed every two forcing periods.

Download Yalim et al. supplementary movie 2(Video)
Video 1.4 MB

Yalim et al. supplementary movie 3

Animation of the normalized Q-criterion for a quasiperiodic state in figure 6, strobed every two forcing periods.

Download Yalim et al. supplementary movie 3(Video)
Video 6.2 MB

Yalim et al. supplementary movie 4

Isotherms during a ten-forcing period transient wave-breaking event at forcing frequency 1.34 and forcing amplitude 0.3; see figure 7.

Download Yalim et al. supplementary movie 4(Video)
Video 29 MB

Yalim et al. supplementary movie 5

Normalized Q-criterion colored by temperature during a ten-forcing period transient wave-breaking event at forcing frequency 1.34 and forcing amplitude 0.3; see figure 7.

Download Yalim et al. supplementary movie 5(Video)
Video 45.1 MB

Yalim et al. supplementary movie 6

Isotherms and normalized Q-criterion during a ten-forcing period transient wave-breaking event at forcing frequency 1.34 and forcing amplitude 0.3; see figure 8.

Download Yalim et al. supplementary movie 6(Video)
Video 48.9 MB