Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-28T10:13:46.090Z Has data issue: false hasContentIssue false
  • English
  • Français

Pair interaction of catalytically active colloids: from assembly to escape

Published online by Cambridge University Press:  16 June 2016

Nima Sharifi-Mood ,
Ali Mozaffari  and
Ubaldo M. Córdova-Figueroa
Nima Sharifi-Mood
Affiliation:
Chemical and Biomolecular Engineering, University of Pennsylvania, Philadelphia, PA 19104, USA
Ali Mozaffari
Affiliation:
Benjamin Levich Institute and Department of Chemical Engineering, City College of the City University of New York, New York, NY 10031, USA
Ubaldo M. Córdova-Figueroa
Affiliation:
Department of Chemical Engineering, University of Puerto Rico – Mayagüez, Mayagüez, PR 00681, USA
Get access Rights & Permissions [Opens in a new window]

Abstract

The dynamics and pair trajectories of two self-propelled (active) colloids in a quiescent fluid for both axisymmetric and asymmetric cases are reported. The autonomous motions of the colloids are due to a catalytic chemical reaction taking place asymmetrically on their surfaces that generates a concentration gradient of interactive solutes around the particles and actuates particle propulsion. A combined analytical–numerical technique was developed to solve the coupled mass conservation equation for the solute and the hydrodynamics between the colloids in the Stokes flow regime. For axisymmetric motions, the translational swimming velocities of the particles in the near field can be enhanced or weakened (compared to their motions when they are far apart) depending on the relative orientations of their active sections. Moreover, it can be shown that different surface activities of two symmetric particles, e.g. an inert versus a catalytic particle or two catalytic particles, can also lead to a propulsion where the far-field swimming velocity for the inert and the catalytic particle attenuate as 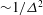${\sim}1/{\it\Delta}^{2}$ and 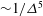${\sim}1/{\it\Delta}^{5}$ respectively, ${\it\Delta}$ is the non-dimensional centre-to-centre distance. For asymmetric motions, our analysis indicates two possible scenarios for pair trajectories of catalytically active particles: either the particles approach, come into contact and assemble or they interact and move away from each other (escape). It is found that the direction of particle rotations is the key factor in determining the escape or assembly scenario due to an interplay between both hydrodynamic and phoretic effects. Based on the analysis, a phase diagram is sketched for the pair trajectory of the catalytically active particles as a function of reacting surface coverages and their initial relative orientations with respect to each other. We believe this study has important implications in elucidation of collective behaviours of autophoretically self-propelled colloids and would be certainly a guide for experimentalists to design and control active systems.

JFM classification

Complex Fluids: Complex Fluids Low-Reynolds-number flows: Low-Reynolds-number flows Biological Fluid Dynamics: Propulsion
Type
Papers
Information
Journal of Fluid Mechanics , Volume 798 , 10 July 2016 , pp. 910 - 954
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anderson, J. 1989 Colloid transport by interfacial forces. Annu. Rev. Fluid Mech. 21, 61–99.Google Scholar
Anderson, J. & Prieve, D. 1984 Diffusiophoresis: migration of colloidal particles in gradients of solute concentration. Sep. Purif. Methods 13, 67.Google Scholar
Aranson, I. 2013 Collective behavior in out-of-equilibrium colloidal suspensions. C. R. Phys. 14, 518–527.Google Scholar
Asakura, S. & Oosawa, F. 1954 On interaction between two bodies immersed in a solution of macromolecules. J. Chem. Phys. 22, 1255–1256.CrossRefGoogle Scholar
Batchelor, G. 1983 Diffusion in a dilute polydisperse system of interacting spheres. J. Fluid Mech. 131, 155–175.Google Scholar
Bialké, J., Speck, T. & Löwen, H. 2015 Active colloidal suspensions: clustering and phase behavior. J. Non-Cryst. Solids 407, 367–375.Google Scholar
Bird, R., Stewart, W. & Lightfoot, E. 2006 Transport Phenomena, 2nd edn. Wiley.Google Scholar
Blake, J. 1971 A spherical envelope approach to ciliary propulsion. J. Fluid Mech. 46, 199–208.Google Scholar
Brady, J. 2011 Particle motion driven by solute gradients with application to autonomous motion: continuum and colloidal perspectives. J. Fluid Mech. 667, 216–259.Google Scholar
Brady, J. & Bossis, G. 1988 Stokesian dynamics. Annu. Rev. Fluid Mech. 20, 111–157.Google Scholar
Buttinoni, I., Bialké, J., Kümmel, F., Löwen, H., Bechinger, C. & Speck, T. 2013 Dynamical clustering and phase separation in suspensions of self-propelled colloidal particles. Phys. Rev. Lett. 110, 238301.Google Scholar
Cates, M. & Tailleur, J. 2015 Motility-induced phase separation. Annu. Rev. Condens. Matter Phys. 6 (1), 219–244.Google Scholar
Cooley, M. & O’Neill, M. 1969 On the slow motion of two spheres in contact along their line of centres through a viscous fluid. Math. Proc. Camb. Phil. Soc. 66, 407–415.Google Scholar
Córdova-Figueroa, U. & Brady, J. 2008 Osmotic propulsion: the osmotic motor. Phys. Rev. Lett. 100, 158303.CrossRefGoogle ScholarPubMed
Córdova-Figueroa, U., Brady, J. & Shklyaev, S. 2013 Osmotic propulsion of colloidal particles via constant surface flux. Soft Matt. 9, 6382–6390.Google Scholar
Coti, K., Belowich, M., Liong, M., Ambrogio, M., Lau, Y., Khatib, H., Zink, J., Khashab, N. & Stoddart, J. 2009 Mechanised nanoparticles for drug delivery. Nanoscale 1, 16–39.Google Scholar
Davis, M. 1969 The slow translation and rotation of two unequal spheres in a viscous fluid. Chem. Engng Sci. 24, 1769–1776.CrossRefGoogle Scholar
Ebbens, S., Tu, M.-H., Howse, J. & Golestanian, R. 2012 Size dependence of the propulsion velocity for catalytic janus-sphere swimmers. Phys. Rev. E 85, 020401.Google Scholar
Elfring, G. 2015 A note on the reciprocal theorem for the swimming of simple bodies. Phys. Fluids 27, 023101.Google Scholar
Elfring, G. & Lauga, E. 2015 Complex fluids in biological systems: experiment, theory, and computation. In Theory of Locomotion Through Complex Fluids, pp. 283–317. Springer.Google Scholar
Frankel, A. & Khair, A. 2014 Dynamics of a self-diffusiophoretic particle in shear flow. Phys. Rev. E 90, 013030.Google Scholar
Gao, W., Kagan, D., Pak, O. S., Clawson, C., Campuzano, S., Chuluun-Erdene, E., Shipton, E., Fullerton, E. E., Zhang, L., Lauga, E. et al. 2012 Cargo-towing fuel-free magnetic nanomotors for targeted drug delivery. Small 8, 460.Google Scholar
Gao, W. & Wang, J. 2014 The environmental impact of micro/nanomachines: a review. ACS Nano 8, 3170–3180.Google Scholar
Goldman, A., Cox, R. & Brenner, H. 1966 The slow motion of two identical arbitrarily oriented spheres through a viscous fluid. Chem. Engng Sci. 21, 1151–1170.Google Scholar
Golestanian, R., Liverpool, T. & Ajdari, A. 2007 Designing phoretic micro- and nano-swimmers. New J. Phys. 9, 126.CrossRefGoogle Scholar
Grima, R. 2005 Strong-coupling dynamics of a multicellular chemotactic system. Phys. Rev. Lett. 95, 128103.Google Scholar
Happel, J. & Brenner, H. 1983 Low Reynolds Number Hydrodynamics with Special Applications to Particulate Media. Martinus Nijhoff Publishers.Google Scholar
Hong, Y., Blackman, N., Kopp, N., Sen, A. & Velegol, D. 2007 Chemotaxis of nonbiological colloidal rods. Phys. Rev. Lett. 99, 178103.CrossRefGoogle ScholarPubMed
Howse, J., Jones, R., Ryan, A., Gough, T., Vafabakhsh, R. & Golestanian, R. 2007 Self-motile colloidal particles: from directed propulsion to random walk. Phys. Rev. Lett. 99, 048102.Google Scholar
Ibele, M., Mallouk, T. & Sen, A. 2009 Schooling behavior of light-powered autonomous micromotors in water. Angew. Chem. Intl Ed. Engl. 48 (18), 3308–3312.Google Scholar
Ishikawa, T., Simmonds, M. & Pedley, T. 2006 Hydrodynamic interaction of two swimming model micro-organisms. J. Fluid Mech. 568, 119–160.Google Scholar
Jeffery, G. 1915 On the steady rotation of a solid of revolution in a viscous fluid. Proc. Lond. Math. Soc. 14, 327–338.CrossRefGoogle Scholar
Jeffrey, D. & Chen, H. S. 1977 The virtual mass of a sphere moving toward a plane wall. Trans. ASME J. Appl. Mech. 44, 166–167.Google Scholar
Jenkins, P. & Snowden, M. 1996 Depletion flocculation in colloidal dispersions. Adv. Colloid Interface Sci. 68, 57–96.Google Scholar
Jiang, H.-R., Yoshinaga, N. & Sano, M. 2010 Active motion of a janus particle by self-thermophoresis in a defocused laser beam. Phys. Rev. Lett. 105, 268302.CrossRefGoogle Scholar
Kagan, D., Laocharoensuk, R., Zimmerman, M., Clawson, C., Balasubramanian, S., Kang, D., Bishop, D., Sattayasamitsathit, S., Zhang, L. & Wang, J. 2010 Rapid delivery of drug carriers propelled and navigated by catalytic nanoshuttles. Small 6, 2741.Google Scholar
Keh, H. & Chen, S. 1988 Electrophoresis of a colloidal sphere parallel to a dielectric plane. J. Fluid Mech. 194, 377–390.Google Scholar
Khair, A. 2013 Diffusiophoresis of colloidal particles in neutral solute gradients at finite Péclet number. J. Fluid Mech. 731, 64–94.CrossRefGoogle Scholar
Kim, S. & Karrila, S. 2005 Microhydrodynamics: Principles and Selected Applications. Dover.Google Scholar
Lee, S. & Leal, G. 1980 Motion of a sphere in the presence of a plane interface. Part 2. An exact solution in bipolar co-ordinates. J. Fluid Mech. 98, 193.Google Scholar
Li, J., Singh, V., Sattayasamitsathit, S., Orozco, J., Kaufmann, K., Dong, R., Gao, W., Jurado-Sanchez, B., Fedorak, Y. & Wang, J. 2014 Water-driven micromotors for rapid photocatalytic degradation of biological and chemical warfare agents. ACS Nano 8, 11118–11125.CrossRefGoogle ScholarPubMed
Lighthill, M. 1952 On the squirming motion of nearly spherical deformable bodies through liquids at very small reynolds numbers. Commun. Pure Appl. Maths 5, 109–118.Google Scholar
Masoud, H. & Stone, H. 2014 A reciprocal theorem for marangoni propulsion. J. Fluid Mech. 741, R4.Google Scholar
Maude, A. 1961 End effects in a falling-sphere viscometer. Br. J. Appl. Phys. 12, 293.Google Scholar
Michelin, S. & Lauga, E. 2014 Phoretic self-propulsion at finite Péclet numbers. J. Fluid Mech. 747, 572–604.CrossRefGoogle Scholar
Michelin, S. & Lauga, E. 2015 Autophoretic locomotion from geometric asymmetry. Eur. Phys. J. E 38, 7.Google Scholar
Michelin, S., Lauga, E. & Bartolo, D. 2013 Spontaneous autophoretic motion of isotropic particles. Phys. Fluids 25 (6), 061701.Google Scholar
Moran, J. & Posner, J. 2011 Electrokinetic locomotion due to reaction induced charge electrophoresis. J. Fluid Mech. 680, 31.Google Scholar
Moran, J., Wheat, P. & Posner, J. 2010 Locomotion of electrocatalytic nanomotors due to reaction induced charge autoelectrophoresis. Phys. Rev. E 81, 065302.Google Scholar
Mozaffari, A., Sharifi-Mood, N., Koplik, J. & Maldarelli, C. 2016 Self-diffusiophoretic colloidal propulsion near a solid boundary. Phys. Fluids 28 (5), 053107.Google Scholar
O’Neill, M. 1969 Exact solutions of the equations of slow viscous flow generated by the asymmetrical motion of two equal spheres. Appl. Sci. Res. 21, 452–466.Google Scholar
O’Neill, M. & Majumdar, S. 1970a Asymmetrical slow viscous fluid motions caused by the translation or rotation of two spheres. Part I: the determination of exact solutions for any values of the ratio of radii and separation parameters. Z. Angew. Math. Phys. 21, 164–179.CrossRefGoogle Scholar
O’Neill, M. & Majumdar, S. 1970b Asymmetrical slow viscous fluid motions caused by the translation or rotation of two spheres. Part II: asymptotic forms of the solutions when the minimum clearance between the spheres approaches zero. Z. Angew. Math. Phys. 21 (2), 180–187.Google Scholar
Orozco, J., Jurado-Sánchez, B., Wagner, G., Gao, W., Vazquez-Duhalt, R., Sattayasamitsathit, S., Galarnyk, M., Cortés, A., Saintillan, D. & Wang, J. 2014 Bubble-propelled micromotors for enhanced transport of passive tracers. Langmuir 30, 5082–5087.Google Scholar
Palacci, J., Sacanna, S., Steinberg, A., Pine, D. & Chaikin, P. 2013 Living crystals of light-activated colloidal surfers. Science 339, 936–940.Google Scholar
Paxton, W., Baker, P., Kline, T., Wang, Y., Mallouk, T. & Sen, A. 2006 Catalytically induced electrokinetics for motors and micropumps. J. Am. Chem. Soc. 128, 14881.Google Scholar
Paxton, W., Kistler, K., Olmeda, C., Sen, A., St. Angelo, S., Cao, Y., Mallouk, T., Lammert, P. & Crespi, V. 2004 Catalytic nanomotors: Autonomous movement of striped nanorods. J. Am. Chem. Soc. 126, 13424.Google Scholar
Popescu, M., Dietrich, S., Tasinkevych, M. & Ralston, J. 2010 Phoretic motion of spheroidal particles due to self-generated solute gradients. Eur. Phys. J. E 31, 351.Google Scholar
Popescu, M., Tasinkevych, M. & Dietrich, S. 2011 Pulling and pushing a cargo with a catalytically active carrier. Eur. Phys. Lett. 95 (2), 28004.Google Scholar
Redner, G., Hagan, M. & Baskaran, A. 2013 Structure and dynamics of a phase-separating active colloidal fluid. Phys. Rev. Lett. 110, 055701.Google Scholar
Reigh, S. & Kapral, R. 2015 Catalytic dimer nanomotors: continuum theory and microscopic dynamics. Soft Matt. 11, 3149–3158.Google Scholar
Sanchez, A., Solovev, A., Schulze, S. & Schmidt, O. 2011 Controlled manipulation of multiple cells using catalytic microbots. Chem. Commun. 47, 698.Google Scholar
Sengupta, A., van Teeffelen, S. & Löwen, H. 2009 Dynamics of a microorganism moving by chemotaxis in its own secretion. Phys. Rev. E 80, 031122.Google Scholar
Sharifi-Mood, N. 2013 The diffusiophoretic motion of colloids. PhD thesis, The City College of New York.Google Scholar
Sharifi-Mood, N., Koplik, J. & Maldarelli, C. 2013a Diffusiophoretic self-propulsion of colloids driven by a surface reaction: the sub-micron particle regime for exponential and van der waals interactions. Phys. Fluids 25, 012001.Google Scholar
Sharifi-Mood, N., Koplik, J. & Maldarelli, C. 2013b Molecular dynamics simulation of the motion of colloidal nanoparticles in a solute concentration gradient and a comparison to the continuum limit. Phys. Rev. Lett. 111, 184501.Google Scholar
Shklyaev, S., Brady, J. & Córdova-Figueroa, U. 2014 Non-spherical osmotic motor: chemical sailing. J. Fluid Mech. 748, 488–520.Google Scholar
Spielman, L. 1970 Viscous interactions in brownian coagulation. J. Colloid Interface Sci. 33, 562–571.Google Scholar
Stimson, M. & Jeffery, G. 1926 The motion of two spheres in a viscous fluid. Proc. Lond. Math. Soc. 111, 110–116.Google Scholar
Stone, H. & Samuel, A. 1996 Propulsion of microorganisms by surface distortions. Phys. Rev. Lett. 77, 4102–4104.Google Scholar
Sundararajan, S., Lammert, P., Zudans, A., Crespi, V. & Sen, A. 2008 Catalytic motors for transport of colloidal cargo. Nano Lett. 8 (5), 1271–1276.Google Scholar
Teubner, M. 1982 The motion of charged colloidal particles in electric fields. J. Chem. Phys. 76 (11), 5564–5573.Google Scholar
Thakur, S. & Kapral, R. 2010 Self-propelled nanodimer bound state pairs. J. Chem. Phys. 133, 204505.CrossRefGoogle ScholarPubMed
Theurkauff, I., Cottin-Bizonne, C., Palacci, J., Ybert, C. & Bocquet, L. 2012 Dynamic clustering in active colloidal suspensions with chemical signaling. Phys. Rev. Lett. 108, 268303.Google Scholar
Uspal, W., Popescu, M., Dietrich, S. & Tasinkevych, M. 2015 Self-propulsion of a catalytically active particle near a planar wall: from reflection to sliding and hovering. Soft Matt. 11, 434–438.Google Scholar
Wakiya, S. 1967 Slow motions of a viscous fluid around two spheres. J. Phys. Soc. Japan 22, 1101–1109.Google Scholar
Wang, J. 2012 Cargo-towing synthetic nanomachines: towards active transport in microchip devices. Lab on a Chip 12, 1944–1950.Google Scholar
Yan, W. & Brady, J. 2015 The swim force as a body force. Soft Matt. 11, 6235–6244.Google Scholar
Yariv, E. & Brenner, H. 2003 Near-contact electrophoretic motion of a sphere parallel to a planar wall. J. Fluid Mech. 484, 85–111.Google Scholar
Zeng, S., Zinchenko, A. & Davis, R. 1999 Electrophoretic motion of two interacting particles. J. Colloid Interface Sci. 209, 282–301.Google Scholar
Zöttl, A. & Stark, H. 2014 Hydrodynamics determines collective motion and phase behavior of active colloids in quasi-two-dimensional confinement. Phys. Rev. Lett. 112, 118101.Google Scholar