Published online by Cambridge University Press: 25 October 2018
The dynamics of a small rigid spherical particle in an unbounded pulsating vortex is considered, keeping constant the particle Stokes number 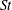 $St$ and varying the particle-to-fluid density ratio
$St$ and varying the particle-to-fluid density ratio 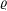 $\unicode[STIX]{x1D71A}$ and the pulsation frequency of the vortex
$\unicode[STIX]{x1D71A}$ and the pulsation frequency of the vortex 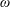 $\unicode[STIX]{x1D714}$. We show that the asymptotic dynamics of a particle of given
$\unicode[STIX]{x1D714}$. We show that the asymptotic dynamics of a particle of given  $St$ and
$St$ and 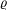 $\unicode[STIX]{x1D71A}$ can be controlled by varying
$\unicode[STIX]{x1D71A}$ can be controlled by varying 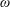 $\unicode[STIX]{x1D714}$, turning the vortex core either into an attractor or a repellor. The creation of non-trivial particle limit cycles characterizes the boundaries between centrifugal and centripetal regions in parameter space. The discovered phenomenon is termed oscillatory switching centrifugation and its implications for particle demixing processes, biological protocols, lab-on-a-chip devices and dynamical systems theory are discussed at the end.
$\unicode[STIX]{x1D714}$, turning the vortex core either into an attractor or a repellor. The creation of non-trivial particle limit cycles characterizes the boundaries between centrifugal and centripetal regions in parameter space. The discovered phenomenon is termed oscillatory switching centrifugation and its implications for particle demixing processes, biological protocols, lab-on-a-chip devices and dynamical systems theory are discussed at the end.