Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-30T23:27:00.516Z Has data issue: false hasContentIssue false
  • English
  • Français

Open capillary siphons

Published online by Cambridge University Press:  09 December 2021

Kaizhe Wang Open the ORCID record for Kaizhe Wang [Opens in a new window] ,
Pejman Sanaei Open the ORCID record for Pejman Sanaei [Opens in a new window] ,
Jun Zhang Open the ORCID record for Jun Zhang [Opens in a new window]  and
Leif Ristroph Open the ORCID record for Leif Ristroph [Opens in a new window]
Kaizhe Wang
Affiliation:
Applied Mathematics Laboratory, Courant Institute, New York University, New York, NY 10012, USA Department of Physics, New York University, New York, NY 10003, USA NYU–ECNU Joint Research Institute of Physics at NYU Shanghai, Shanghai 200062, PR China
Pejman Sanaei
Affiliation:
Department of Mathematics, New York Institute of Technology, New York, NY 10023-7692, USA
Jun Zhang
Affiliation:
Applied Mathematics Laboratory, Courant Institute, New York University, New York, NY 10012, USA Department of Physics, New York University, New York, NY 10003, USA NYU–ECNU Joint Research Institute of Physics at NYU Shanghai, Shanghai 200062, PR China
Leif Ristroph*
Affiliation:
Applied Mathematics Laboratory, Courant Institute, New York University, New York, NY 10012, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

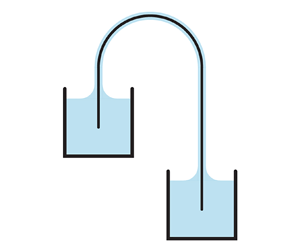
Flow in the inverted U-shaped tube of a conventional siphon can be established and maintained only if the tube is filled and closed, so that air does not enter. We report on siphons that operate entirely open to the atmosphere by exploiting surface tension effects. Such capillary siphoning is demonstrated by paper tissue that bridges two containers and conveys water from the upper to the lower. We introduce a more controlled system consisting of grooves in a wetting solid, formed here by pressing together hook-shaped metallic rods. The dependence of flux on siphon geometry is systematically measured, revealing behaviour different from the conventional siphon. The flux saturates when the height difference between the two container's free surfaces is large; it also has a strong dependence on the climbing height from the source container's free surface to the apex. A one-dimensional theoretical model is developed, taking into account the capillary pressure due to surface tension, pressure loss due to viscous friction, and driving by gravity. Numerical solutions are in good agreement with experiments, and the model suggests hydraulic interpretations for the observed flux dependence on geometrical parameters. The operating principle and characteristics of capillary siphoning revealed here can inform biological phenomena and engineering applications related to directional fluid transport.

JFM classification

Interfacial Flows (free surface): Capillary flows
Type
JFM Rapids
Information
Journal of Fluid Mechanics , Volume 932 , 10 February 2022 , R1
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Ayyaswamy, P.S., Catton, I. & Edwards, D.K. 1974 Capillary flow in triangular grooves. J. Appl. Mech. 332–336.CrossRefGoogle Scholar
Boatwright, A., Hughes, S. & Barry, J. 2015 The height limit of a siphon. Sci. Rep. 5 (1), 16790.CrossRefGoogle ScholarPubMed
Chen, H., Zhang, P., Zhang, L., Liu, H., Jiang, Y., Zhang, D., Han, Z. & Jiang, L. 2016 Continuous directional water transport on the peristome surface of Nepenthes alata. Nature 532 (7597), 85–89.CrossRefGoogle ScholarPubMed
Concus, P. & Finn, R. 1969 On the behavior of a capillary surface in a wedge. Proc. Natl Acad. Sci. USA 63 (2), 292–299.CrossRefGoogle Scholar
Cooray, H., Cicuta, P. & Vella, D. 2012 The capillary interaction between two vertical cylinders. J. Phys.: Condens. Matter 24 (28), 284104.Google ScholarPubMed
De Gennes, P.-G., Brochard-Wyart, F. & Quéré, D. 2013 Capillarity and Wetting Phenomena: Drops, Bubbles, Pearls, Waves. Springer Science & Business Media.Google Scholar
Dudukovic, N.A., Fong, E.J., Gemeda, H.B., DeOtte, J.R., Cerón, M.R., Moran, B.D., Davis, J.T., Baker, S.E. & Duoss, E.B. 2021 Cellular fluidics. Nature 595 (7865), 58–65.CrossRefGoogle ScholarPubMed
Fairbank, H.A. & Lane, C.T. 1949 Rollin film rates in liquid helium. Phys. Rev. 76 (8), 1209.CrossRefGoogle Scholar
Feng, S., Zhu, P., Zheng, H., Zhan, H., Chen, C., Li, J., Wang, L., Yao, X., Liu, Y. & Wang, Z. 2021 Three-dimensional capillary ratchet-induced liquid directional steering. Science 373 (6561), 1344–1348.CrossRefGoogle ScholarPubMed
Ganci, S. & Yegorenkov, V. 2008 Historical and pedagogical aspects of a humble instrument. Eur. J. Phys. 29 (3), 421.CrossRefGoogle Scholar
Guo, Z. & Cao, Y. 2005 Experimental studies of biliquid capillary siphons. Chem. Engng Sci. 60 (10), 2621–2626.CrossRefGoogle Scholar
Higuera, F.J., Medina, A. & Linan, A. 2008 Capillary rise of a liquid between two vertical plates making a small angle. Phys. Fluids 20 (10), 102102.CrossRefGoogle Scholar
Hughes, S.W. 2010 A practical example of a siphon at work. Phys. Education 45 (2), 162.CrossRefGoogle Scholar
James, D.F. 1966 Open channel siphon with viscoelastic fluids. Nature 212 (5063), 754–756.CrossRefGoogle Scholar
Kim, W., Peaudecerf, F., Baldwin, M.W. & Bush, J.W.M. 2012 The hummingbird's tongue: a self-assembling capillary syphon. Proc. R. Soc. B: Biol. Sci. 279 (1749), 4990–4996.CrossRefGoogle ScholarPubMed
Kingsolver, J.G. & Daniel, T.L. 1983 Mechanical determinants of nectar feeding strategy in hummingbirds: energetics, tongue morphology, and licking behavior. Oecologia 60 (2), 214–226.CrossRefGoogle ScholarPubMed
Ponomarenko, A., Quéré, D. & Clanet, C. 2011 A universal law for capillary rise in corners. J. Fluid Mech. 666, 146–154.CrossRefGoogle Scholar
Potter, A. & Barnes, F.H. 1971 The siphon. Phys. Education 6 (5), 362.CrossRefGoogle Scholar
Pozrikidis, C. 2010 Computation of three-dimensional hydrostatic menisci. IMA J. Appl. Maths 75 (3), 418–438.CrossRefGoogle Scholar
Prajitno, D.H., Maulana, A. & Syarif, D.G. 2016 Effect of surface roughness on contact angle measurement of nanofluid on surface of stainless steel 304 by sessile drop method. In Journal of Physics: Conference Series, vol. 739, p. 012029. IOP Publishing.CrossRefGoogle Scholar
Prokunin, A.N. 1983 A model of elastic deformation for the description of withdrawal of polymer solutions. Rheol. Acta 22 (4), 374–379.CrossRefGoogle Scholar
Ramette, J.J. & Ramette, R.W. 2011 Siphonic concepts examined: a carbon dioxide gas siphon and siphons in vacuum. Phys. Education 46 (4), 412–416.CrossRefGoogle Scholar
Richert, A. & Binder, P.-M. 2011 Siphons, revisited. Phys. Teacher 49 (2), 78–80.CrossRefGoogle Scholar
Rollin, B.V. & Simon, F. 1939 On the ‘film’ phenomenon of liquid helium $\text {II}$. Physica 6 (2), 219–230.CrossRefGoogle Scholar
Sloane, T.O. 1886 The capillary siphon. Sci. Am. 55 (22), 345–345.CrossRefGoogle Scholar
Suh, J.-S., Greif, R. & Grigoropoulos, C.P. 2001 Friction in micro-channel flows of a liquid and vapor in trapezoidal and sinusoidal grooves. Intl J. Heat Mass Transfer 44 (16), 3103–3109.CrossRefGoogle Scholar
Tanner, R.I. 1966 Pressure losses in viscometric capillary tubes of slowly varying diameter. Brit. J. Appl. Phys. 17 (5), 663.CrossRefGoogle Scholar
Terpiłowski, K., Hołysz, L., Rymuszka, D. & Banach, R. 2016 Comparison of contact angle measurement methods of liquids on metal alloys. Ann. Univ. Mariae Curie-Skłodowska, AA–Chemia 71 (1), 89–104.Google Scholar
White, F.M. 2015 Fluid Mechanics. McGraw-Hill Education.Google Scholar