Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-12-02T21:30:21.578Z Has data issue: false hasContentIssue false
  • English
  • Français

On the nature of fluctuations in turbulent Rayleigh–Bénard convection at large Prandtl numbers

Published online by Cambridge University Press:  03 August 2016

Ping Wei  and
Guenter Ahlers
Ping Wei
Affiliation:
Department of Physics, University of California, Santa Barbara, CA 93106, USA
Guenter Ahlers*
Affiliation:
Department of Physics, University of California, Santa Barbara, CA 93106, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We report experimental results for the power spectra, variance, skewness and kurtosis of temperature fluctuations in turbulent Rayleigh–Bénard convection (RBC) of a fluid with Prandtl number $Pr=12.3$ in cylindrical samples with aspect ratios 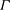$\unicode[STIX]{x1D6E4}$ (diameter 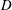$D$ over height 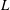$L$) of 0.50 and 1.00. The measurements were primarily for the radial positions 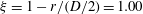$\unicode[STIX]{x1D709}=1-r/(D/2)=1.00$ and 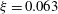$\unicode[STIX]{x1D709}=0.063$. In both cases, data were obtained at several vertical locations 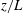$z/L$. For all locations, there is a frequency range of about a decade over which the spectra can be described well by the power law $P(f)\sim f^{-\unicode[STIX]{x1D6FC}}$. For all 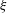$\unicode[STIX]{x1D709}$ and 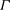$\unicode[STIX]{x1D6E4}$, the $\unicode[STIX]{x1D6FC}$ value is less than one near the top and bottom plates and increases as 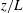$z/L$ or 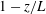$1-z/L$ increase from 0.01 to 0.5. This differs from the finding for$Pr=0.8$ (He et al., Phys. Rev. Lett., vol. 112, 2014, 174501) and the expectation for the downstream velocity of turbulent wall-bounded shear flow (Rosenberg, J. Fluid Mech., vol. 731, 2013, pp. 46–63), where 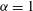$\unicode[STIX]{x1D6FC}=1$ is found or expected in an inner layer (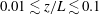$0.01\lesssim z/L\lesssim 0.1$) near the wall but in the bulk. The variance is described better by a power law 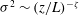$\unicode[STIX]{x1D70E}^{2}\sim (z/L)^{-\unicode[STIX]{x1D701}}$ than by the logarithmic dependence found or expected for 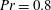$Pr=0.8$ and for turbulent shear flow. For both 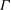$\unicode[STIX]{x1D6E4}$, we found that, independent of Rayleigh number, $\unicode[STIX]{x1D701}\simeq 2/3$ near the sidewall (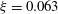$\unicode[STIX]{x1D709}=0.063$), where plumes primarily rise or fall and the large-scale circulation (LSC) dynamics is most influential. This result agrees with a model due to Priestley (Turbulent Transfer in the Lower Atmosphere, 1959, University of Chicago Press) for convection over a horizontal heated surface. However, we found 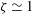$\unicode[STIX]{x1D701}\simeq 1$ along the sample centreline (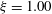$\unicode[STIX]{x1D709}=1.00$), where there are relatively few plumes moving vertically and the LSC dynamics is expected to be less important; that result is consistent with one of two possible interpretations by Adrian (Intl J. Heat Mass Transfer, vol. 39, 1996, pp. 2303–2310) of a model due to Libchaber et al. (J. Fluid Mech., vol. 204, 1989, pp. 1–30). We discuss the composite nature of fluctuations in turbulent RBC, with contributions from intrinsic background fluctuations, plumes, the stochastic dynamics of the LSC, and the sloshing and torsional mode of the LSC. None of the models advanced so far explicitly consider all of these contributions.

JFM classification

Turbulent Flows: Turbulent convection Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 802 , 10 September 2016 , pp. 203 - 244
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adrian, R. 1996 Variation of temperature and velocity fluctuations in turbulent thermal convection over horizontal surfaces. Intl J. Heat Mass Transfer 39, 2303–2310.Google Scholar
Adrian, R., Ferreira, R. & Boberg, T. 1986 Turbulent thermal convection in wide horizontal fluid layers. Exp. Fluids 4, 121–141.CrossRefGoogle Scholar
Ahlers, G. 1972 Convective heat transport between horizontal parallel plates. Bull. Am. Phys. Soc. 17, 59–60.Google Scholar
Ahlers, G. 1974 Low-temperature studies of the Rayleigh–Bénard instability and turbulence. Phys. Rev. Lett. 33, 1185–1188.Google Scholar
Ahlers, G. 1975 The Rayleigh–Bénard instability at helium temperatures. In Fluctuations, Instabilities and Phase Transitions (ed. Riste, T.), pp. 181–193. Plenum.Google Scholar
Ahlers, G. 1995 Over two decades of pattern formation, a personal perspective. In 25 Years of Nonequilibrium Statistical Mechanics (ed. Brey, J. J., Marro, J., Rubi, J. M. & San Miguel, M.), Lecture Notes in Physics, vol. 445, pp. 91–124. Springer.CrossRefGoogle Scholar
Ahlers, G. 2009 Turbulent convection. Physics 2, 74.CrossRefGoogle Scholar
Ahlers, G. & Behringer, R. P. 1978 Evolution of turbulence from the Rayleigh–Bénard instability. Phys. Rev. Lett. 40, 712–715.CrossRefGoogle Scholar
Ahlers, G., Bodenschatz, E., Funfschilling, D., Grossmann, S., He, X., Lohse, D., Stevens, R. & Verzicco, R. 2012 Logarithmic temperature profiles in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 109, 114501.Google Scholar
Ahlers, G., Bodenschatz, E. & He, X. 2014 Logarithmic temperature profiles of turbulent Rayleigh–Bénard convection in the classical and ultimate state for a Prandtl number of 0.8. J. Fluid Mech. 758, 436–467.Google Scholar
Ahlers, G. & Graebner, J. E. 1972 Time dependence in convective heat transport between horizontal parallel plates. Bull. Am. Phys. Soc. 17, 61.Google Scholar
Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81, 503–538.CrossRefGoogle Scholar
Ahlers, G. & Walden, R. W. 1980 Turbulence near onset of convection. Phys. Rev. Lett. 44, 445–448.Google Scholar
Ahlers, G. & Xu, X. 2001 Prandtl-number dependence of heat transport in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 86, 3320–3323.Google Scholar
Aubin, D. & Dalmedico, A. 2002 Writing the history of dynamical systems and chaos: longue durée and revolution, disciplines and cultures. Historia Math. 29, 273–339.Google Scholar
Bailon-Cuba, J., Emran, M. S. & Schumacher, J. 2010 Aspect ratio dependence of heat transfer and large-scale flow in turbulent convection. J. Fluid Mech. 655, 152–173.CrossRefGoogle Scholar
Batchelor, G. 1959 Small-scale variation of convected quantities like temperature in turbulent fluid. Part 1. General discussion and the case of small conductivity. J. Fluid Mech. 5, 113–133.Google Scholar
Belmonte, A. & Libchaber, A. 1996 Thermal signature of plumes in turbulent convection: the skewness of the derivative. Phys. Rev. E 53, 4893–4898.Google Scholar
Bolgiano, R. 1959 Turbulent spectra in a stably stratified atmosphere. J. Geophys. Res. 64, 2226–2229.Google Scholar
Bosbach, J., Weiss, S. & Ahlers, G. 2012 Plume fragmentation by bulk interactions in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 108, 054501.Google Scholar
Boussinesq, J. 1903 Theorie Analytique de la Chaleur. vol. 2. Gauthier-Villars.Google Scholar
Brown, E. & Ahlers, G. 2006a Effect of the Earth’s Coriolis force on turbulent Rayleigh–Bénard convection in the laboratory. Phys. Fluids 18, 125108.Google Scholar
Brown, E. & Ahlers, G. 2006b Rotations and cessations of the large-scale circulation in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 568, 351–386.Google Scholar
Brown, E. & Ahlers, G. 2007a Large-scale circulation model of turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 98, 134501.Google Scholar
Brown, E. & Ahlers, G. 2007b Temperature gradients, and search for non-Boussinesq effects, in the interior of turbulent Rayleigh–Bénard convection. Europhys. Lett. 80, 14001.CrossRefGoogle Scholar
Brown, E. & Ahlers, G. 2008a Azimuthal asymmetries of the large-scale circulation in turbulent Rayleigh–Bénard convection. Phys. Fluids 20, 105105.Google Scholar
Brown, E. & Ahlers, G. 2008b A model of diffusion in a potential well for the dynamics of the large-scale circulation in turbulent Rayleigh–Bénard convection. Phys. Fluids 20, 075101.Google Scholar
Brown, E. & Ahlers, G. 2009 The origin of oscillations of the large-scale circulation of turbulent Rayleigh–Bénard convection. J. Fluid Mech. 638, 383–400.Google Scholar
Brown, E., Nikolaenko, A. & Ahlers, G. 2005 Reorientation of the large-scale circulation in turbulent Rayleigh-Bénard convection. Phys. Rev. Lett. 95, 084503.Google Scholar
Castaing, B., Gunaratne, G., Heslot, F., Kadanoff, L., Libchaber, A., Thomae, S., Wu, X. Z., Zaleski, S. & Zanetti, G. 1989 Scaling of hard thermal turbulence in Rayleigh–Bénard convection. J. Fluid Mech. 204, 1–30.Google Scholar
Chillà, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh–Bénard convection. Eur. Phys. J. E 35, 58.Google Scholar
Chung, M., Yun, H. & Adrian, R. 1992 Scale analysis and wall-layer model for the temperature profile in a turbulent thermal convection. Intl J. Heat Mass Transfer 35, 43–51.CrossRefGoogle Scholar
Cioni, S., Ciliberto, S. & Sommeria, J. 1997 Strongly turbulent Rayleigh–Bénard convection in mercury: comparison with results at moderate Prandtl number. J. Fluid Mech. 335, 111–140.Google Scholar
Cooley, J. & Tukey, J. 1965 An algorithm for the machine calculation of complex Fourier series. Math. Comput. 19, 297–301.Google Scholar
Corrsin, S. 1951 On the spectrum of isotropic temperature fluctuations in an isotropic turbulence. J. Appl. Phys. 22, 469–473.Google Scholar
Du, Y. B. & Tong, P. 2001 Temperature fluctuations in a convection cell with rough upper and lower surfaces. Phys. Rev. E 63, 046303.Google Scholar
Fernandez, R. & Adrian, R. 2002 Scaling of velocity and temperature fluctuations in turbulent thermal convection. Exp. Therm. Fluid Sci. 26, 355–360.Google Scholar
Frisch, U. 1995 Turbulence. Cambridge University Press.Google Scholar
Frish, U. & Morph, R. 1981 Intermittency in nonlinear dynamics and singularities at complex times. Phys. Rev. A 23, 2673–2705.CrossRefGoogle Scholar
Funfschilling, D. & Ahlers, G. 2004 Plume motion and large scale circulation in a cylindrical Rayleigh–Bénard cell. Phys. Rev. Lett. 92, 194502.CrossRefGoogle Scholar
Funfschilling, D., Brown, E. & Ahlers, G. 2008 Torsional oscillations of the large-scale circulation in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 607, 119–139.Google Scholar
Greenside, H. S., Ahlers, G., Hohenberg, P. C. & Walden, R. W. 1982 A simple stochastic model for the onset of turbulence in Rayleigh–Bénard convection. Physica D 5, 322–334.Google Scholar
Grossmann, S. & Lohse, D. 1992 Scaling in hard turbulent Rayleigh–Bénard flow. Phys. Rev. A 46, 903–917.Google Scholar
Grossmann, S. & Lohse, D. 1993 Characteristic scales in Rayleigh–Bénard turbulence. Phys. Lett. A 173, 58–62.Google Scholar
Grossmann, S. & Lohse, D. 1997 Fractal-dimension crossovers in turbulent passive scalar signals. Europhys. Lett. 27, 347–352.Google Scholar
Grossmann, S. & Lohse, D. 2000 Scaling in thermal convection: a unifying view. J. Fluid. Mech. 407, 27–56.Google Scholar
Grossmann, S. & Lohse, D. 2001 Thermal convection for large Prandtl number. Phys. Rev. Lett. 86, 3316–3319.Google Scholar
Grossmann, S. & Lohse, D. 2004 Fluctuations in turbulent Rayleigh–Bénard convection: the role of plumes. Phys. Fluids 16, 4462–4472.CrossRefGoogle Scholar
Grossmann, S. & Lohse, D. 2012 Logarithmic temperature profiles in the ultimate regime of thermal convection. Phys. Fluids 24, 125103.Google Scholar
He, G.-W. & Zhang, J.-B. 2006 Elliptic model for space–time correlations in turbulent shear flows. Phys. Rev. E 73, 055303.Google Scholar
He, X., Bodenschatz, E. & Ahlers, G.2016a Fluctuations in turbulent Rayleigh–Bénard convection at large Rayleigh numbers, to be published.Google Scholar
He, X., Bodenschatz, E. & Ahlers, G. 2016b Azimuthal diffusion of the large-scale-circulation plane, and absence of significant non-Boussinesq effects, in turbulent convection near the ultimate-state transition. J. Fluid Mech. 791, R3.Google Scholar
He, X. & Tong, P. 2009 Measurements of the thermal dissipation field in turbulent Rayleigh–Benard convection. Phys. Rev. E 79, 026306.Google Scholar
He, X., van Gils, D., Bodenschatz, E. & Ahlers, G. 2014 Logarithmic spatial variations and universal f -1 power spectra of temperature fluctuations in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 112, 174501.CrossRefGoogle ScholarPubMed
He, X., van Gils, D., Bodenschatz, E. & Ahlers, G. 2015 Reynolds numbers and the elliptic approximation near the ultimate state of turbulent Rayleigh–Bénard convection. New J. Phys. 17, 063028.Google Scholar
Heideman, M., Johnson, D. & Burrus, C. 1984 Gauss and the history of the fast Fourier transform. IEEE ASSP Mag. 1, 14–21.CrossRefGoogle Scholar
Heslot, F., Castaing, B. & Libchaber, A. 1987 Transition to turbulence in helium gas. Phys. Rev. A 36, 5870–5873.Google Scholar
Hogg, J. & Ahlers, G. 2013 Reynolds-number measurements for low-Prandtl-number turbulent convection of large aspect-ratio samples. J. Fluid Mech. 725, 664–680.Google Scholar
Hultmark, M., Vallikivi, M., Bailey, S. & Smits, A. 2012 Turbulent pipe flow at extreme Reynolds numbers. Phys. Rev. Lett. 108, 094501.Google Scholar
Kadanoff, L. P. 2001 Turbulent heat flow: structures and scaling. Phys. Today 54 (8), 34–39.Google Scholar
Kraichnan, R. 1968 Small-scale structure of a scalar field convected by turbulence. Phys. Fluids 11, 945–953.CrossRefGoogle Scholar
Kraichnan, R. 1974 Convection of a passive scalar by a quasi-uniform random straining field. J. Fluid Mech. 64, 737–762.Google Scholar
Kunnen, R. P. J., Clercx, H. J. H. & Geurts, B. J. 2008 Breakdown of large-scale circulation in turbulent rotating convection. Europhys. Lett. 84, 24001.Google Scholar
Lam, S., Shang, X. D., Zhou, S. Q. & Xia, K.-Q. 2002 Prandtl-number dependence of the viscous boundary layer and the Reynolds-number in Rayleigh–Bénard convection. Phys. Rev. E 65, 066306.Google ScholarPubMed
Landau, L. D. & Lifshitz, E. M. 1963 Fluid Mechanics. Pergamon Press.Google Scholar
Li, L., Shi, N., du Puits, R., Resagk, C., Schumacher, J. & Thess, A. 2012 Boundary layer analysis in turbulent Rayleigh–Bénard convection in air: experiment versus simulation. Phys. Rev. E 86, 026315.Google Scholar
Libchaber, L. & Maurer, J. 1978 Local probe in a Rayleigh–Bénard experiment in liquid helium. J. Phys. Lett. 39, 369–372.Google Scholar
Lohse, D. & Xia, K.-Q. 2010 Small-scale properties of turbulent Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 42, 335–364.Google Scholar
Lorenz, E. N. 1963 Deterministic nonperiodic flow. J. Atmos. Sci. 20, 130–141.Google Scholar
Lui, S. L. & Xia, K.-Q. 1998 Spatial structure of the thermal boundary layer in turbulent convection. Phys. Rev. E 57, 5494–5503.Google Scholar
Malkus, M. V. R. 1954 The heat transport and spectrum of thermal turbulence. Proc. R. Soc. Lond. A 225, 196–212.Google Scholar
Maurer, J. & Libchaber, L. 1979 Rayleigh–Bénard experiment in liquid helium; frequency locking and the onset of turbulence. J. Physique Lett. 40, 419–423.Google Scholar
Maurer, J. & Libchaber, L. 1980 Effect of the Prandtl number on the onset of turbulence in liquid 4He. J. Physique Lett. 41, 515–518.Google Scholar
Niemela, J. J., Skrbek, L., Sreenivasan, K. R. & Donnelly, R. 2000 Turbulent convection at very high Rayleigh numbers. Nature 404, 837–840.Google Scholar
Nikolaenko, A., Brown, E., Funfschilling, D. & Ahlers, G. 2005 Heat transport by turbulent Rayleigh–Bénard convection in cylindrical cells with aspect ratio one and less. J. Fluid Mech. 523, 251–260.CrossRefGoogle Scholar
Oberbeck, A. 1879 Über die Wärmeleitung der Flüssigkeiten bei Berücksichtigung der Strömungen infolge von Temperaturdifferenzen. Ann. Phys. Chem. 7, 271–292.Google Scholar
Obukhov, A. M. 1949 Structure of the temperature field in a turbulent flow. Izv. Akad. Nauk SSSR Geogr. Geofiz 13, 58–69.Google Scholar
Obukhov, A. M. 1959 On the influence of Archimedean forces on the structure of the temperature field in a turbulent flow. Dokl. Akad. Nauk SSSR 125, 1246–1248.Google Scholar
Perry, A. E. & Abel, C. 1975 Scaling laws for pipe-flow turbulence. J. Fluid Mech. 67, 257–271.Google Scholar
Perry, A. E. & Abel, C. 1977 Asymptotic similarity of turbulence structures in smooth- and rough-walled pipes. J. Fluid Mech. 79, 785–799.Google Scholar
Perry, A. E. & Chong, M. S. 1982 On the mechanism of wall turbulence. J. Fluid Mech. 119, 173–217.Google Scholar
Perry, A. E., Henbest, S. & Chong, M. S. 1986 A theoretical and experimental study of wall turbulence. J. Fluid Mech. 165, 163–199.Google Scholar
Pope, S. B. 2000 Turbulent Flow. Cambridge University Press.Google Scholar
Priestley, C. H. B. 1954 Convection from a large horizontal surface. Austral. J. Phys. 7, 176–201.Google Scholar
Priestley, C. H. B. 1959 Turbulent Transfer in the Lower Atmosphere. University of Chicago Press.Google Scholar
Procaccia, I., Ching, E. S. C., Constantin, P., Kadanoff, L. P., Libchaber, A. & Wu, X. Z. 1991 Transition to convective turbulence: the role of thermal plumes. Phys. Rev. A 44, 8091–8102.Google Scholar
du Puits, R., Resagk, C. & Thess, A. 2009 Structure of viscous boundary layers in turbulent Rayleigh–Bénard convection. Phys. Rev. E 80, 036381.Google Scholar
Qiu, X. L., Shang, X. D., Tong, P. & Xia, K.-Q. 2004 Velocity oscillations in turbulent Rayleigh–Bénard convection. Phys. Fluids 16, 412–423.Google Scholar
Qiu, X. L. & Tong, P. 2000 Large-scale coherent rotation and oscillation in turbulent thermal convection. Phys. Rev. E 61, R6075–R6078.Google Scholar
Qiu, X. L. & Tong, P. 2001 Large scale velocity structures in turbulent thermal convection. Phys. Rev. E 64, 036304.Google Scholar
Rosenberg, B. J., Hultmark, M., Vallikivi, M., Bailey, S. C. C. & Smits, A. J. 2013 Turbulence spectra in smooth- and rough-wall pipe flow at extreme Reynolds numbers. J. Fluid Mech. 731, 46–63.Google Scholar
Sano, M., Wu, X. Z. & Libchaber, A. 1989 Turbulence in helium-gas free convection. Phys. Rev. A 40, 6421–6430.Google Scholar
She, Z. & Jackson, E. 1993 On the universal form of energy spectra in fully developed turbulence. Phys. Fluids A 5, 1526–1528.Google Scholar
Shishkina, O., Stevens, R., Grossmann, S. & Lohse, D. 2010 Boundary layer structure in turbulent thermal convection and its consequences for the required numerical resolution. New J. Phys. 12, 075022.Google Scholar
Song, H., Brown, E., Hawkins, R. & Tong, P. 2014 Dynamics of large-scale circulation of turbulent thermal convection in a horizontal cylinder. J. Fluid Mech. 740, 136–167.Google Scholar
Spiegel, E. A. 1971 Convection in stars. Annu. Rev. Astron. Astrophys. 9, 323–352.Google Scholar
Sreenivasan, K. R. 1996 The passive scalar spectrum and the Obukhov–Corrsin constant. Phys. Fluids 8, 189–196.CrossRefGoogle Scholar
Stevens, R. J., Clercx, H. J. & Lohse, D. 2011 Effect of plumes on measuring the large scale circulation. Phys. Fluids 23, 095110.Google Scholar
Sun, C., Cheung, Y. & Xia, K. 2008 Experimental studies of the viscous boundary layer properties in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 605, 79–113.Google Scholar
Sun, C., Xi, H. D. & Xia, K. Q. 2005a Azimuthal symmetry, flow dynamics, and heat transport in turbulent thermal convection in a cylinder with an aspect ratio of 0.5. Phys. Rev. Lett. 95, 074502.Google Scholar
Sun, C., Xia, K. Q. & Tong, P. 2005b Three-dimensional flow structures and dynamics of turbulent thermal convection in a cylindrical cell. Phys. Rev. E 72, 026302.Google Scholar
Threlfall, D. C. 1975 Free convection in low temperature gaseous helium. J. Fluid Mech. 67, 17–28.Google Scholar
Tilgner, A., Belmonte, A. & Libchaber, A. 1993 Temperature and velocity profiles of turbulence convection in water. Phys. Rev. E 47, R2253–R2256.Google Scholar
Townsend, A. A. 1976 The Structure of Turbulent Shear Flow. Cambridge University Press.Google Scholar
Verzicco, R. & Camussi, R. 1999 Prandtl number effects in convective turbulence. J. Fluid Mech. 383, 55–73.Google Scholar
Wang, J. & Xia, K.-Q. 2003 Spatial variations of the mean and statistical quantities in the thermal boundary layers of turbulent convection. Eur. Phys. J. B 32, 127–136.Google Scholar
Wei, P. & Ahlers, G. 2014 Logarithmic temperature profiles in the bulk of turbulent Rayleigh–Bénard convection for a Prandtl number of 12.3. J. Fluid Mech. 758, 809–830.Google Scholar
Weiss, S. & Ahlers, G. 2011a Heat transport by turbulent rotating Rayleigh–Bénard convection. J. Fluid Mech. 684, 407–426.Google Scholar
Weiss, S. & Ahlers, G. 2011b The large-scale flow structure in turbulent rotating Rayleigh–Bénard convection. J. Fluid Mech. 688, 461–492.Google Scholar
Weiss, S. & Ahlers, G. 2011c Turbulent Rayleigh–Bénard convection in a cylindrical container with aspect ratio 𝛤 = 0. 50 and Prandtl number Pr = 4. 38. J. Fluid Mech. 676, 5–40.Google Scholar
Weiss, S. & Ahlers, G. 2013 Effect of tilting on turbulent convection: cylindrical samples with aspect ratio 𝛾 = 0. 50. J. Fluid Mech. 715, 314–334.CrossRefGoogle Scholar
Wonsiewicz, B., Storm, A. & Sieber, J. 1978 UNIX time-sharing system: microcomputer control of apparatus, machinery, and experiments. Bell Syst. Tech. J. 57, 2209–2232.Google Scholar
Wu, X. Z., Kadanoff, L., Libchaber, A. & Sano, M. 1990 Frequency power spectrum of temperature-fluctuation in free-convection. Phys. Rev. Lett. 64, 2140.Google Scholar
Xi, H.-D. & Xia, K.-Q. 2008 Flow mode transition in turbulent thermal convection. Phys. Fluids 20, 055104.Google Scholar
Xi, H.-D., Zhou, S.-Q., Zhou, Q., Chan, T.-S. & Xia, K.-Q. 2009 Origin of the temperature oscillation in turbulent thermal convection. Phys. Rev. Lett. 102, 044503.Google Scholar
Xia, K.-Q., Lam, S. & Zhou, S. Q. 2002 Heat-flux measurement in high-Prandtl-number turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 88, 064501.Google Scholar
Xu, X., Bajaj, K. M. S. & Ahlers, G. 2000 Heat transport in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 84, 4357–4360.Google Scholar
Zhong, J.-Q. & Ahlers, G. 2010 Heat transport and the large-scale circulation in rotating turbulent Rayleigh–Bénard convection. J. Fluid Mech. 665, 300–333.Google Scholar
Zhou, Q., Xi, H.-D., Zhou, S.-Q., Sun, C. & Xia, K.-Q. 2009 Oscillations of the large-scale circulation in turbulent Rayleigh–Bénard convection: the sloshing mode and its relationship with the torsional mode. J. Fluid Mech. 630, 367–390.Google Scholar
Zhou, S. Q. & Xia, K.-Q. 2001 Scaling properties of the temperature field in convective turbulence. Phys. Rev. Lett. 87, 064501.Google Scholar