Hostname: page-component-745bb68f8f-mzp66 Total loading time: 0 Render date: 2025-02-11T00:00:15.728Z Has data issue: false hasContentIssue false
  • English
  • Français

On the interaction between planar incident shock with finite width and cylindrical boundary layer at Mach 2.0

Published online by Cambridge University Press:  06 February 2025

Fangbo Li Open the ORCID record for Fangbo Li [Opens in a new window] ,
Hexia Huang Open the ORCID record for Hexia Huang [Opens in a new window] ,
Huijun Tan Open the ORCID record for Huijun Tan [Opens in a new window] ,
Xin Li Open the ORCID record for Xin Li [Opens in a new window] ,
Hang Yu  and
Yuan Qin Open the ORCID record for Yuan Qin [Opens in a new window]
Fangbo Li
Affiliation:
College of Energy and Power Engineering, Nanjing University of Aeronautics and Astronautics, Nanjing 210016, PR China
Hexia Huang*
Affiliation:
College of Energy and Power Engineering, Nanjing University of Aeronautics and Astronautics, Nanjing 210016, PR China
Huijun Tan
Affiliation:
College of Energy and Power Engineering, Nanjing University of Aeronautics and Astronautics, Nanjing 210016, PR China
Xin Li
Affiliation:
College of Energy and Power Engineering, Nanjing University of Aeronautics and Astronautics, Nanjing 210016, PR China
Hang Yu
Affiliation:
College of Energy and Power Engineering, Nanjing University of Aeronautics and Astronautics, Nanjing 210016, PR China
Yuan Qin
Affiliation:
College of Energy and Power Engineering, Nanjing University of Aeronautics and Astronautics, Nanjing 210016, PR China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

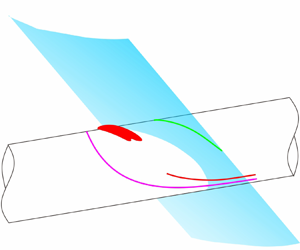
The interaction between planar incident shocks and cylindrical boundary layers is prevalent in missiles equipped with inverted inlets, which typically leads to substantial three-dimensional flow separation and the formation of vortical flow. This study utilizes wind-tunnel experiments and theoretical analysis to elucidate the shock structure, surface topology and pressure distributions induced by a planar shock with finite width impinging on a cylinder wall at Mach 2.0. In the central region, a refraction phenomenon occurs as the transmitted shock bends within the boundary layer, generating a series of compression waves that coalesce into a shock, forming a ‘shock triangle’ structure. As the incident shock propagates backward along both sides, it gradually evolves into a Mach stem, where the transmitted shock refracts the expansion wave. The incident shock interacts with the boundary layer, resulting in the formation of a highly swept separation region that yields a pair of counter-rotating horseshoe-like vortices above the separation lines. These vortices facilitate the accumulation of low-energy fluid on both sides. Although the interaction of the symmetry plane aligns with free-interaction-theory, the separation shock angle away from the centre significantly deviates from the predicted value owing to the accumulation of low-energy fluids. The primary separation line and pressure distribution jointly exhibit an elliptical similarity on the cylindrical surface. Furthermore, the potential unsteady behaviour is assessed, and the Strouhal number of the low-frequency oscillation is found to be 0.0094, which is insufficient to trigger significant alterations in the flow field structure.

JFM classification

Compressible Flows: Supersonic flow Compressible Flows: Shock waves Boundary Layers: Boundary layer separation
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 1002 , 10 January 2025 , A41
Check for updates
Copyright
© The Author(s), 2025. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alvi, F.S. & Settles, G.S. 1992 Physical model of the swept shock wave/boundary-layer interaction flowfield. AIAA J. 30 (9), 2252–2258.CrossRefGoogle Scholar
Anderson, J.D. 2010 Fundamentals of Aerodynamics. McGraw-Hill Education.Google Scholar
Babinsky, H. & Harvey, J.K. 2011 Shock Wave-Boundary-Layer Interactions. Cambridge University Press.CrossRefGoogle Scholar
Babinsky, H. & Ogawa, H. 2008 SBLI control for wings and inlets. Shock Waves 18 (2), 89–96.CrossRefGoogle Scholar
Barry, F.W., Shapiro, A.H. & Neumann, E.P. 1951 Numerical study on wall temperature effects on shock wave/turbulent boundary-layer interaction. J. Aeronaut. Sci. 18 (4), 229–238.CrossRefGoogle Scholar
Bhardwaj, S., Hemanth Chandra Vamsi, K. & Sriram, R. 2022 On the scaling of three-dimensional shock-induced separated flow due to protuberances. Phys. Fluids 34 (7), 076115.CrossRefGoogle Scholar
Brosh, A., Kussoy, M.I. & Hung, C.M. 1985 Experimental and numerical investigation of a shock wave impingementon a cylinder. AIAA J. 23 (6), 840–846.CrossRefGoogle Scholar
Chang, J.T., Li, N., Xu, K.J., Bao, W. & Yu, D.R. 2017 Recent research progress on unstart mechanism, detection and control of hypersonic inlet. Prog. Aeosp. Sci. 89, 1–22.CrossRefGoogle Scholar
Chapman, D.R., Kuehn, D.M. & Larson, H.K. 1958 Investigation of separated flows in supersonic and subsonic streams with emphasis on the effect of transition. NACA Tech. Rep. NACA-TR-1356.Google Scholar
Clemens, N.T. & Narayanaswamy, V. 2014 Low-frequency unsteadiness of shock wave/turbulent boundary layer interactions. Annu. Rev. Fluid Mech. 46 (1), 469–492.CrossRefGoogle Scholar
Combs, C.S., Lash, E.L., Kreth, P.A. & Schmisseur, J.D. 2018 Investigating unsteady dynamics of cylinder-induced shock-wave/transitional boundary-layer interactions. AIAA J. 56 (4), 1588–1599.CrossRefGoogle Scholar
Délery, J. 1985 Shock wave/turbulent boundary layer interaction and its control. Prog. Aeosp. Sci. 22, 209–280.CrossRefGoogle Scholar
Délery, J. 1993 Contribution of laser doppler velocimetry to the physical description of shock wave/turbulent boundary layer interaction with incidence on turbulence modeling. Tech. Rep. 792. AGARD/FDP-VKI Special Course on Shock Wave $\tilde{\rm B}$oundary Layer Interactions in Supersonic and Hypersonic Flows.Google Scholar
Délery, J. & Marvin, J.G. 1986 Shock-wave boundary layer interactions. Tech. Rep. 280. AGARDograph.Google Scholar
Dolling, D.S. 2001 Fifty years of shock-wave/boundary-layer interaction research: what next? AIAA J. 39 (8), 1517–1531.CrossRefGoogle Scholar
van Driest, E.R. 1951 Turbulent boundary layer in compressible fluids. J. Aeronaut. Sci. 18 (3), 145–160.CrossRefGoogle Scholar
Dupont, P., Haddad, C. & Debiève, J.F. 2006 Space and time organization in a shock-induced separated boundary layer. J. Fluid Mech. 559, 255–277.CrossRefGoogle Scholar
Fage, A. & Sargent, R.H. 1947 Shock-wave and boundary-layer phenomena near a flat surface. In Proceedings of the Royal Society of London, vol. 190, pp. 1–20. Royal Society of London.CrossRefGoogle Scholar
Fang, J., Zheltovodov, A.A., Yao, Y., Moulinec, C. & Emerson, D.R. 2020 Comparative study on single-incident and 1 dual-incident shock wave / turbulent boundary layer 2 interactions with identical total deflection angle. J. Fluid Mech. 940 (A7), 1–37.Google Scholar
Ferri, A. 1940 Experimental results with airfoils tested in the high-speed tunnel at Guidonia. NACA Tech. Mem. NACA-TM-946.Google Scholar
Gai, S.L. & Teh, S.L. 2000 Interaction between a conical shock wave and a plane turbulent boundary layer. AIAA J. 38 (5), 804–811.CrossRefGoogle Scholar
Gaitonde, D.V. 2015 Progress in shock wave/boundary layer interactions. Prog. Aeosp. Sci. 72, 80–99.CrossRefGoogle Scholar
Gaitonde, D. & Knight, D. 1991 Numerical investigation of bleed on three-dimensional turbulent interactions due to sharp fins. AIAA J. 29 (11), 1878–1885.CrossRefGoogle Scholar
Garcia, M., Hoffman, E.N.A., LaLonde, E.J., Combs, C.S., Pohlman, M., Smith, C., Gragston, M.T. & Schmisseur, J.D. 2018 Effects of surface roughness on shock-wave/turbulent boundary-layer interaction at mach 4 over a hollow cylinder flare model. Fluids 7 (9), 286.CrossRefGoogle Scholar
Green, J.E. 1970 Interactions between shock waves and turbulent boundary layers. Prog. Aeosp. Sci. 11, 235–340.CrossRefGoogle Scholar
Grossman, I.J. & Bruce, P.J.K. 2018 Confinement effects on regular–irregular transition in shock-wave–boundary-layer interactions. J. Fluid Mech. 853, 171–204.CrossRefGoogle Scholar
Henderson, L.F. 1966 The refraction of a plane shock wave at a gas interface. J. Fluid Mech. 26, 607–637.CrossRefGoogle Scholar
Henderson, L.F. 1967 The reflexion of a shock wave at a rigid wall in the presence of a boundary layer. J. Fluid Mech. 30, 699–722.CrossRefGoogle Scholar
Herrmann, C. & Koschel, W. 2002 Experimental investigation of the internal compression inside a hypersonic intake. AIAA Paper 2002-4130.CrossRefGoogle Scholar
Hu, Z.M., Myong, R.S, Kimand, M.S. & Cho, T.H. 2009 Downstream flow condition effects on the rr $\to$ mr transition of asymmetric shock waves in steady flows. J. Fluid Mech. 620, 43–62.CrossRefGoogle Scholar
Huang, H.X., et al. 2023 A review of the shock-dominated flow in a hypersonic inlet/isolator. Prog. Aeosp. Sci. 143, 376–421.Google Scholar
Huang, H.X., Tan, H.J., Sun, S. & Wang, Z.Y. 2018 behavior of shock train in curved isolators with complex background waves. AIAA J. 56 (1), 329–341.CrossRefGoogle Scholar
Kendall, A. & Koochesfahani, M. 2008 A method for estimating wall friction in turbulent wall-bounded flows. Exp. Fluids 44 (5), 773–780.CrossRefGoogle Scholar
Kiriakos, R.M., Pournadali Khamseh, A., Gianoukakis, G. & DeMauro, E.P. 2022 PIV investigation of the effects of shock generator wedge angle and trailing-edge expansion waves on impinging shock/turbulent boundary layer interactions over a cylinder. AIAA Paper 2022-1328.CrossRefGoogle Scholar
Krishnan, L., Sandham, N.D. & Steelant, J. 2009 Shock-wave/boundary-layer interactions in a model scramjet intake. AIAA J. 47 (7), 1680–1691.CrossRefGoogle Scholar
Kubota, H. & Stollery, J.L. 1982 An experimental study of the interaction between a glancing shock wave and a turbulent boundary layer. J. Fluid Mech. 116, 431–458.CrossRefGoogle Scholar
Kumar, P. & Mahesh, K. 2018 Analysis of axisymmetric boundary layers. J. Fluid Mech. 849, 927–941.CrossRefGoogle Scholar
Kussoy, M.I., Viegas, J.R. & Horstman, C.C. 1980 Investigation of a three-dimensional shock wave separated turbulent boundary layer. AIAA J. 18 (12), 1477–1484.CrossRefGoogle Scholar
Li, H., Chpoun, A. & Ben-dor, G. 1999 Analytical and experimental investigations of the reflection of asymmetric shock waves in steady flows. J. Fluid Mech. 390, 25–43.CrossRefGoogle Scholar
Li, X., Zhang, Y., Tan, H.J., Jin, Y. & Li, C. 2022 Comparative study on single-incident and 1 dual-incident shock wave / turbulent boundary layer 2 interactions with identical total deflection angle. J. Fluid Mech. 940 (A7), 1–34.CrossRefGoogle Scholar
Li, X., Zhang, Y., Tan, H.J., Sun, S., Yu, H. & Jin, Y. 2023 Separation length scaling for dual-incident shock 1 wave/turbulent boundary layer interactions with 2 different shock wave distances. J. Fluid Mech. 940 (A7), 1–34.Google Scholar
Liepmann, H.W., Roshko, A. & Dhawan, S. 1952 On Reflection of Shock Waves from Boundary Layers. NACA Tech. Rep. NACA-TR-1100.Google Scholar
Lindörfer, S.A., Combs, C.S., Kreth, P.A., Bond, R.B. & Schmisseur, J.D. 2020 Scaling of cylinder-generated shock-wave/turbulent boundary-layer interactions. Shock Waves 30 (4), 395–407.CrossRefGoogle Scholar
Lueptow, R.M. 1990 Turbulent boundary layer on a cylinder in axial flow. AIAA J. 28 (10), 1705–1706.CrossRefGoogle Scholar
Mason, F., Natarajan, K. & Kumar, R. 2021 Shock-wave/boundary-layer interactions on an axisymmetric body at Mach 2. AIAA J. 59 (11), 4530–4543.CrossRefGoogle Scholar
Monkewitz, P.A. & Nagib, H.M. 2023 The hunt for the Kármán ‘constant’ revisited. J. Fluid Mech. 967 (A15), 1–19.CrossRefGoogle Scholar
Morkovin, M.V., Migotsky, E., Bailey, H.E. & Phinney, R.E. 1952 Experiments on interaction of shock waves and cylindrical bodies at supersonic speeds. J. Aeronaut. Sci. 19 (4), 237–248.CrossRefGoogle Scholar
Nimura, K., Tsutsui, F., Ktamura, K. & Nonaka, S. 2023 Aerodynamic effects of surface protuberance sizes on slender-bodied supersonic vehicle. AIAA Paper 2023-0241.CrossRefGoogle Scholar
Nompelis, I., Candler, G.V. & Holden, M.S. 2003 Effect of vibrational nonequilibrium on hypersonic double-cone experiments. AIAA J. 41 (11), 2162–2169.CrossRefGoogle Scholar
Panaras, A.G. 1996 Review of the physics of swept-shock/boundary layer interactions. Prog. Aeosp. Sci. 32, 173–244.CrossRefGoogle Scholar
Panov, Y.A. 1971 Interaction of incident three-dimensional shock with a turbulent boundary layer. Fluid Dyn. 3 (3), 108–110.CrossRefGoogle Scholar
Pickles, J.D., Mettu, B.R., Subbareddy, P.K. & Narayanaswamy, V. 2019 On the mean structure of sharp-fin-induced shock wave/turbulent boundary layer interactions over a cylindrical surface. J. Fluid Mech. 865, 212–246.CrossRefGoogle Scholar
Ramesh, M.D. & Tannehill, J.C. 2004 Correlations to predict the streamwise influence regions in supersonic turbulent flows. J. Aircraft 41 (2), 274–283.CrossRefGoogle Scholar
Robertson, G., Kumar, R., Eymann, A.T. & Morton, S.A. 2015 Experimental and numerical study of shock-wave boundary layer interactions on an axisymmetric body. AIAA Paper 2015-2935.CrossRefGoogle Scholar
Sabnis, K. & Babinsky, H. 2023 A review of three-dimensional shock wave–boundary-layer interactions. Prog. Aeosp. Sci. 89, 1–22.Google Scholar
Schmisseur, J.D. & Dolling, D.S. 1994 Fluctuating wall pressures near separation in highly swept turbulent interactions. AIAA J. 32 (6), 1151–1157.CrossRefGoogle Scholar
Sedney, R. 1973 A survey of the effects of small protuberances on boundary-layer flows. AIAA J. 11 (6), 782–792.CrossRefGoogle Scholar
Settles, G.S. & Kimmel, R.L. 1986 Similarity of quasi conical shock wave/turbulent boundary-layer interactions. AIAA J. 24 (1), 47–53.CrossRefGoogle Scholar
Settles, G.S. & Lu, F.K. 1985 Conical similarity of shock/boundary-layer interactions generated byswept and unswept fins. AIAA J. 23 (7), 1021–1027.CrossRefGoogle Scholar
Sheng, F.J., Tan, H.J., Zhuang, Y., Huang, H.X., Chen, H. & Wang, W.X. 2018 Visualization of conical vortex and shock in swept shock/turbulent boundary layer interaction flow. J. Vis. 21 (6), 909–914.CrossRefGoogle Scholar
Shutts, W.H., Hartwig, W.H. & Weiler, J.E. 1955 Final report on turbulent boundary layer and skin friction measurements on a smooth, thermally insulated flat plate at supersonic speeds. Tech. Rep. Defence Research Laboratory, the University of Texas.Google Scholar
Souverein, L.J., Bakker, P.G. & Dupont, P. 2013 A scaling analysis for turbulen shock-wave/boundary-layer interactions. J. Fluid Mech. 714, 505–535.CrossRefGoogle Scholar
Squire, L.C. 1961 The motion of a thin oil sheet under the steady boundary layer on a body. J. Fluid Mech. 11 (2), 167–179.CrossRefGoogle Scholar
Sriram, R., Srinath, L., Devaraj, M.K.K. & Jagadeesh, G. 2016 On the length scales of hypersonic shock-induced large separation bubbles near leading edges. J. Fluid Mech. 806, 304–355.CrossRefGoogle Scholar
Stephen, E.J., Farnsworth, J.A., Porter, C.O., Decker, R., McLaughlin, T.E. & Dudley, J.G. 2013 Impinging shock-wave boundary-layer interactions on a three-dimensional body. AIAA Paper 2013-2733.CrossRefGoogle Scholar
Voitenko, D.M., Zubkov, A.I. & Panov, Y.A. 1966 Supersonic gas flow past a cylindrical obstacle on a plate. Fluid Dyn. 1 (1), 84–88.CrossRefGoogle Scholar
Volpiani, P.S., Bernardini, M. & Larsson, J. 2018 Effects of a nonadiabatic wall on supersonic shock/boundary-layer interactions. Phys. Rev. Fluids 3 (8), 083401.CrossRefGoogle Scholar
White, F.M. & Majdalani, J. 2006 Viscous Fluid Flow. McGraw-Hill Education.Google Scholar
Wright, M.J., Sinha, K., Olejniczak, J., Candler, G.V., Magruder, T.D. & Smits, A.J. 2000 Numerical and experimental investigation of double-cone shock interactions. AIAA J. 38 (12), 2268–2276.CrossRefGoogle Scholar
Zhu, X.K., Yu, C.P., Tong, F.L. & Li, X.L. 2017 Numerical study on wall temperature effects on shock wave/turbulent boundary-layer interaction. AIAA J. 55 (1), 131–140.CrossRefGoogle Scholar
Zuo, F.Y., Memmolo, A., Huang, G.P. & Pirozzoli, S. 2019 Direct numerical simulation of conical shock wave–turbulent boundary layer interaction. J. Fluid Mech. 877, 167–195.CrossRefGoogle Scholar