Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Ueno, Ichiro
2021.
Experimental Study on Coherent Structures by Particles Suspended in Half-Zone Thermocapillary Liquid Bridges: Review.
Fluids,
Vol. 6,
Issue. 3,
p.
105.
Visone, M.
Lanzetta, M.
Lappa, M.
Lanzaro, C.
and
Polizio, L.
2021.
Three-dimensional simulation of clouds of multi-disperse evaporating saliva droplets in a train cabin.
Physics of Fluids,
Vol. 33,
Issue. 8,
Capobianchi, Paolo
and
Lappa, Marcello
2021.
Particle Accumulation Structures in a 5 cSt Silicone Oil Liquid Bridge: New Data for the Preparation of the JEREMI Experiment.
Microgravity Science and Technology,
Vol. 33,
Issue. 2,
Lappa, Marcello
Burel, Thomas
Kerr, Monica
Crewdson, Georgie
Boaro, Alessio
Capobianchi, Paolo
Bonnieu, Sebastien Vincent
Murphy, Lesley
Randall, Peter
and
Hens, Steven
2022.
Particle Vibration, an Instrument to Study Particle Accumulation Structures On Board the International Space Station.
Microgravity Science and Technology,
Vol. 34,
Issue. 3,
Sakata, Tomoki
Terasaki, Sayo
Saito, Hiroki
Fujimoto, Sorachi
Ueno, Ichiro
Yano, Taishi
Nishino, Koichi
Kamotani, Yasuhiro
and
Matsumoto, Satoshi
2022.
Coherent structures of
m=1
by low-Stokes-number particles suspended in a half-zone liquid bridge of high aspect ratio: Microgravity and terrestrial experiments.
Physical Review Fluids,
Vol. 7,
Issue. 1,
Crewdson, Georgie
and
Lappa, Marcello
2022.
An Investigation into the Behavior of Non-Isodense Particles in Chaotic Thermovibrational Flow.
Fluid Dynamics & Materials Processing,
Vol. 18,
Issue. 3,
p.
497.
Lappa, Marcello
2022.
On the Propagation of Hydrothermal Waves in a Fluid Layer with Two-Way Coupled Dispersed Solid Particles.
Fluids,
Vol. 7,
Issue. 7,
p.
215.
Lappa, Marcello
2022.
Characterization of two-way coupled thermovibrationally driven particle attractee.
Physics of Fluids,
Vol. 34,
Issue. 5,
Santhosh, Balagopal Manayil
and
Lappa, Marcello
2023.
On the relationship between solid particle attractors and thermal inhomogeneities in vibrationally driven fluid-particle systems.
Physics of Fluids,
Vol. 35,
Issue. 10,
Noguchi, Shin
and
Ueno, Ichiro
2023.
Spatial-temporal behaviors of low-Stokes-number particles forming coherent structures in high-aspect-ratio liquid bridges by thermocapillary effect.
Physical Review Fluids,
Vol. 8,
Issue. 11,
Crewdson, Georgie
and
Lappa, Marcello
2023.
Three-dimensional solid particle self-assembly in thermovibrational flow: The case with unidirectional temperature gradient and concurrent vibrations.
Physics of Fluids,
Vol. 35,
Issue. 2,
Terasaki, Sayo
Sensui, Shogo
and
Ueno, Ichiro
2023.
Thermocapillary-driven coherent structures by low-Stokes-number particles and their morphology in high-aspect-ratio liquid bridges.
International Journal of Heat and Mass Transfer,
Vol. 203,
Issue. ,
p.
123772.
Parker, Robert
Capobianchi, Paolo
and
Lappa, Marcello
2023.
Competing particle attractee in liquid bridges.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 381,
Issue. 2245,
Tanaka, Yohsuke
Iwaguchi, Tatsuki
Yamamoto, Yasufumi
Otomo, Ryoko
and
Harada, Shusaku
2024.
VISUALIZATION OF MIXING UPPER AND LOWER STRATIFIED SUSPENSIONS USING RED AND BLUE FLUORESCENT PARTICLES
.
Multiphase Science and Technology,
Vol. 36,
Issue. 2,
p.
29.
Sensui, Shogo
Noguchi, Shin
Kato, Keiichiro
and
Ueno, Ichiro
2024.
Coherent structures formed by small particles in traveling-wave flows.
Physical Review E,
Vol. 110,
Issue. 1,
Santhosh, Balagopal Manayil
and
Lappa, Marcello
2024.
Vibrationally driven particle formations in fluid systems with bimodal thermal inhomogeneities.
Physics of Fluids,
Vol. 36,
Issue. 5,
Kato, Keiichiro
Sensui, Shogo
Noguchi, Shin
Kurose, Kizuku
and
Ueno, Ichiro
2024.
Experimental study on coherent structures by small particles suspended in high aspect-ratio ($$\Gamma =$$ 2.5) thermocapillary liquid bridges of high Prandtl number.
The European Physical Journal Special Topics,
Vol. 233,
Issue. 8-9,
p.
1673.
Noguchi, Shin
and
Ueno, Ichiro
2025.
Quantitative analysis of particle behavior constituting multiple coherent structures in liquid bridges.
Journal of Colloid and Interface Science,
Vol. 684,
Issue. ,
p.
29.
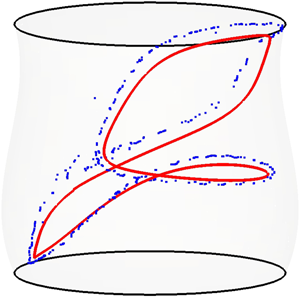
 $m=1$, for which PAS seem to be possible only in a very restricted range of Marangoni numbers. Two distinct families of particle attractors are identified, which coexist in the space of parameters as multiple solutions. We show that the key ingredient needed to unravel the related basins of attraction is the effect of gravity on particles, which may therefore be regarded as an additional mechanism responsible for the formation of these structures in terrestrial liquid bridges.
$m=1$, for which PAS seem to be possible only in a very restricted range of Marangoni numbers. Two distinct families of particle attractors are identified, which coexist in the space of parameters as multiple solutions. We show that the key ingredient needed to unravel the related basins of attraction is the effect of gravity on particles, which may therefore be regarded as an additional mechanism responsible for the formation of these structures in terrestrial liquid bridges.


