Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-24T16:55:04.107Z Has data issue: false hasContentIssue false
  • English
  • Français

Oblique collisions of internal wave beams and associated resonances

Published online by Cambridge University Press:  19 September 2012

T. R. Akylas  and
H. H. Karimi
T. R. Akylas*
Affiliation:
Department of Mechanical Engineering, Massachusetts Institute of Technology, Cambridge, MA 02139, USA
H. H. Karimi
Affiliation:
Department of Mechanical Engineering, Massachusetts Institute of Technology, Cambridge, MA 02139, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Quadratic nonlinear interactions between two colliding internal gravity wave beams in a uniformly stratified fluid, and the resulting radiation of secondary beams with frequencies equal to the sum and difference of those of the primary beams, are discussed. The analysis centres on oblique collisions, involving beams that propagate in different vertical planes. The propagation directions of generated secondary beams are deduced from kinematic considerations and the use of radiation conditions, thus extending to oblique collisions previously derived selection rules for plane collisions. Using small-amplitude expansions, radiated-beam profiles at steady state are also computed in terms of the characteristics of the colliding beams. It is pointed out that, for certain oblique collision configurations, radiated beams with frequency equal to the difference of the primary frequencies have unbounded steady-state amplitude. This resonance, which has no counterpart for plane collisions, is further analysed via the solution of an initial-value problem; ignoring dissipation, the transient resonant response grows in time like 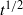, a behaviour akin to that of forced waves at cut-off frequencies.

JFM classification

Geophysical and Geological Flows: Internal waves Geophysical and Geological Flows: Ocean processes Geophysical and Geological Flows: Stratified flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 711 , 25 November 2012 , pp. 337 - 363
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Akylas, T. R. 1984 On the excitation of nonlinear water waves by a moving pressure distribution oscillating at resonant frequency. Phys. Fluids 27, 2803–2807.CrossRefGoogle Scholar
2. Aranha, J. A., Yue, D. K. P. & Mei, C. C. 1982 Nonlinear waves near a cut-off frequency in an acoustic duct: a numerical study. J. Fluid Mech. 121, 465–478.CrossRefGoogle Scholar
3. Bell, T. H. 1975 Lee waves in stratified flows with simple harmonic time dependence. J. Fluid Mech. 67, 705–722.CrossRefGoogle Scholar
4. Gerkema, T., Staquet, C. & Bouruet-Aubertot, P. 2006 Decay of semi-diurnal internal-tide beams due to subharmonic resonance. Geophys. Res. Lett. 33, L08604.CrossRefGoogle Scholar
5. Jiang, C.-H. & Marcus, P. S. 2009 Selection rules for the nonlinear interaction of internal gravity waves. Phys. Rev. Lett. 102, 124502.CrossRefGoogle ScholarPubMed
6. Khatiwala, S. 2003 Generation of internal tides in an ocean of finite depth: analytical and numerical calculations. Deep-Sea Res. 50, 3–21.Google Scholar
7. King, B., Zhang, H. P. & Swinney, H. L. 2010 Tidal flow over three-dimensional topography generates out-of-forcing-plane harmonics. Geophys. Res. Lett. 37, L14606.CrossRefGoogle Scholar
8. Lamb, K. G. 2004 Nonlinear interaction among internal wave beams generated by tidal flow over supercritical topography. Geophys. Res. Lett. 31, L09313.CrossRefGoogle Scholar
9. Lighthill, M. J. 1978 Waves in Fluids. Cambridge University Press.Google Scholar
10. McEwan, A. D. 1973 Interactions between internal gravity waves and their traumatic effect on a continuous stratification. Boundary-Layer Meteorol. 5, 159–175.CrossRefGoogle Scholar
11. Mowbray, D. E. & Rarity, B. S. 1967 A theoretical and experimental investigation of the phase configuration of internal waves of small amplitude in a density stratified fluid. J. Fluid Mech. 28, 1–16.CrossRefGoogle Scholar
12. Peacock, T. & Tabaei, A. 2005 Visualization of nonlinear effects in reflecting internal wave beams. Phys. Fluids 17, 061702.CrossRefGoogle Scholar
13. Stashchuk, N. & Vlasenko, V. 2005 Topographic generation of internal waves by nonlinear superposition of tidal harmonics. Deep-Sea Res. I50, 605–620.CrossRefGoogle Scholar
14. Tabaei, A. & Akylas, T. R. 2003 Nonlinear internal gravity wave beams. J. Fluid Mech. 482, 141–161.CrossRefGoogle Scholar
15. Tabaei, A., Akylas, T. R. & Lamb, K. G. 2005 Nonlinear effects in reflecting and colliding internal wave beams. J. Fluid Mech. 526, 217–243.CrossRefGoogle Scholar
16. Zhang, H. P., King, B. & Swinney, H. L. 2007 Experimental study of internal gravity waves generated by supercritical topography. Phys. Fluids 19, 096602.CrossRefGoogle Scholar