Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-28T12:16:43.279Z Has data issue: false hasContentIssue false
  • English
  • Français

Moffatt-drift-driven large-scale dynamo due to ${\it\alpha}$ fluctuations with non-zero correlation times

Published online by Cambridge University Press:  09 June 2016

Nishant K. Singh
Nishant K. Singh*
Affiliation:
Nordita, KTH Royal Institute of Technology and Stockholm University, Roslagstullsbacken 23, SE-106 91 Stockholm, Sweden
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We present a theory of large-scale dynamo action in a turbulent flow that has stochastic, zero-mean fluctuations of the ${\it\alpha}$ parameter. Particularly interesting is the possibility of the growth of the mean magnetic field due to Moffatt drift, which is expected to be finite in a statistically anisotropic turbulence. We extend the Kraichnan–Moffatt model to explore effects of finite memory of ${\it\alpha}$ fluctuations, in a spirit similar to that of Sridhar & Singh (Mon. Not. R. Astron. Soc., vol. 445, 2014, pp. 3770–3787). Using the first-order smoothing approximation, we derive a linear integro-differential equation governing the dynamics of the large-scale magnetic field, which is non-perturbative in the ${\it\alpha}$-correlation time 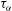${\it\tau}_{{\it\alpha}}$. We recover earlier results in the exactly solvable white-noise limit where the Moffatt drift does not contribute to the dynamo growth/decay. To study finite-memory effects, we reduce the integro-differential equation to a partial differential equation by assuming that 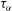${\it\tau}_{{\it\alpha}}$ be small but non-zero and the large-scale magnetic field is slowly varying. We derive the dispersion relation and provide an explicit expression for the growth rate as a function of four independent parameters. When 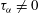${\it\tau}_{{\it\alpha}}\neq 0$, we find that: (i) in the absence of the Moffatt drift, but with finite Kraichnan diffusivity, only strong ${\it\alpha}$ fluctuations can enable a mean-field dynamo (this is qualitatively similar to the white-noise case); (ii) in the general case when also the Moffatt drift is non-zero, both weak and strong ${\it\alpha}$ fluctuations can lead to a large-scale dynamo; and (iii) there always exists a wavenumber (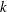$k$) cutoff at some large 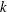$k$ beyond which the growth rate turns negative, irrespective of weak or strong ${\it\alpha}$ fluctuations. Thus we show that a finite Moffatt drift can always facilitate large-scale dynamo action if sufficiently strong, even in the case of weak ${\it\alpha}$ fluctuations, and the maximum growth occurs at intermediate wavenumbers.

JFM classification

MHD and Electrohydrodynamics: Dynamo theory Turbulent Flows: Turbulence theory
Type
Papers
Information
Journal of Fluid Mechanics , Volume 798 , 10 July 2016 , pp. 696 - 716
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Beck, R. 2012 Magnetic fields in galaxies. Space Sci. Rev. 166, 215–230.CrossRefGoogle Scholar
Bhat, P. & Subramanian, K. 2013 Fluctuation dynamos and their Faraday rotation signatures. Mon. Not. R. Astron. Soc. 429, 2469–2481.CrossRefGoogle Scholar
Brandenburg, A., Rädler, K.-H., Rheinhardt, M. & Käpylä, P. J. 2008 Magnetic diffusivity tensor and dynamo effects in rotating and shearing turbulence. Astrophys. J. 676, 740–751.CrossRefGoogle Scholar
Brandenburg, A. & Subramanian, K. 2005 Astrophysical magnetic fields and nonlinear dynamo theory. Phys. Rep. 417, 1–209.CrossRefGoogle Scholar
Chamandy, L., Shukurov, A., Subramanian, K. & Stoker, K. 2014 Non-linear galactic dynamos: a toolbox. Mon. Not. R. Astron. Soc. 443, 1867–1880.CrossRefGoogle Scholar
Chamandy, L., Subramanian, K. & Shukurov, A. 2013a Galactic spiral patterns and dynamo action: I. A new twist on magnetic arms. Mon. Not. R. Astron. Soc. 428, 3569–3589.CrossRefGoogle Scholar
Chamandy, L., Subramanian, K. & Shukurov, A. 2013b Galactic spiral patterns and dynamo action: II. Asymptotic solutions. Mon. Not. R. Astron. Soc. 433, 3274–3289.CrossRefGoogle Scholar
Charbonneau, P. 2010 Dynamo models of the solar cycle. Living Rev. Solar Phys. 7 (3).CrossRefGoogle Scholar
Courvoisier, A., Hughes, D. A. & Tobias, S. M. 2006 𝛼 effect in a family of chaotic flows. Phys. Rev. Lett. 96, 034503.CrossRefGoogle Scholar
Heinemann, T., McWilliams, J. C. & Schekochihin, A. A. 2011 Large-scale magnetic field generation by randomly forced shearing waves. Phys. Rev. Lett. 107, 255004.CrossRefGoogle ScholarPubMed
Hubbard, A. & Brandenburg, A. 2009 Memory effects in turbulent transport. Astrophys. J. 706, 712–726.CrossRefGoogle Scholar
Jones, C. A. 2011 Planetary magnetic fields and fluid dynamos. Annu. Rev. Fluid Mech. 43, 583–614.CrossRefGoogle Scholar
Kleeorin, N. & Rogachevskii, I. 2008 Mean-field dynamo in a turbulence with shear and kinetic helicity fluctuations. Phys. Rev. E 77, 036307.Google Scholar
Kraichnan, R. H. 1976 Diffusion of weak magnetic fields by isotropic turbulence. J. Fluid Mech. 75, 657–676.CrossRefGoogle Scholar
Krause, F. & Rädler, K.-H. 1980 Mean-field Magnetohydrodynamics and Dynamo Theory. Pergamon.Google Scholar
Kucher, P. & Enßlin, T. A. 2011 Magnetic power spectra from Faraday rotation maps. REALMAF and its use on Hydra A. Astron. Astrophys. 529, A13.CrossRefGoogle Scholar
Kulsrud, R. M. 2004 Plasma Physics for Astrophysics. Princeton University Press.Google Scholar
McWilliams, J. C. 2012 The elemental shear dynamo. J. Fluid Mech. 699, 414–452.CrossRefGoogle Scholar
Mitra, D. & Brandenburg, A. 2012 Scaling and intermittency in incoherent 𝛼-shear dynamo. Mon. Not. R. Astron. Soc. 420, 2170–2177.CrossRefGoogle Scholar
Moffatt, H. K. 1978 Magnetic Field Generation in Electrically Conducting Fluids. Cambridge University Press.Google Scholar
Moffatt, H. K. 1983 Transport effects associated with turbulence with particular attention to the influence of helicity. Rep. Prog. Phys. 46, 621–623, 625–664.CrossRefGoogle Scholar
Murgia, M., Govoni, F., Feretti, L., Giovannini, G., Dallacasa, D., Fanti, R., Taylor, G. B. & Dolag, K. 2004 Magnetic fields and Faraday rotation in clusters of galaxies. Astron. Astrophys. 424, 429–446.CrossRefGoogle Scholar
Parker, E. N. 1979 Cosmical Magnetic Fields: Their Origin and Their Activity. Clarendon.Google Scholar
Proctor, M. R. E. 2007 Effects of fluctuation on 𝛼𝛺 dynamo models. Mon. Not. R. Astron. Soc. 382, L39–L42.CrossRefGoogle Scholar
Proctor, M. R. E. 2012 Bounds for growth rates for dynamos with shear. J. Fluid Mech. 697, 504–510.CrossRefGoogle Scholar
Rheinhardt, M., Devlen, E., Rädler, K.-H. & Brandenburg, A. 2014 Mean-field dynamo action from delayed transport. Mon. Not. R. Astron. Soc. 441, 116–126.CrossRefGoogle Scholar
Richardson, K. J. & Proctor, M. R. E. 2012 Fluctuating 𝛼𝛺 dynamos by iterated matrices. Mon. Not. R. Astron. Soc. 422, 53–56.CrossRefGoogle Scholar
Rogachevskii, I. & Kleeorin, N. 2008 Nonhelical mean-field dynamos in a sheared turbulence. Astron. Nachr. 329, 732–736.CrossRefGoogle Scholar
Ruzmaikin, A. A., Shukurov, A. M. & Sokoloff, D. D. 1988 Magnetic Fields of Galaxies. Kluwer.CrossRefGoogle Scholar
Silant’ev, N. A. 2000 Magnetic dynamo due to turbulent helicity fluctuations. Astron. Astrophys. 364, 339–347.Google Scholar
Singh, N. K. & Jingade, N. 2015 Numerical studies of dynamo action in a turbulent shear flow. I. Astrophys. J. 806, 118.CrossRefGoogle Scholar
Singh, N. K. & Sridhar, S. 2011 Transport coefficients for the shear dynamo problem at small Reynolds numbers. Phys. Rev. E 83, 056309.Google ScholarPubMed
Sokolov, D. D. 1997 The disk dynamo with fluctuating spirality. Astron. Rep. 41, 68–72.Google Scholar
Squire, J. & Bhattacharjee, A. 2015 Coherent nonhelical shear dynamos driven by magnetic fluctuations at low Reynolds numbers. Astrophys. J. 813, 52.CrossRefGoogle Scholar
Sridhar, S. & Singh, N. K. 2010 The shear dynamo problem for small magnetic Reynolds numbers. J. Fluid Mech. 664, 265–285.CrossRefGoogle Scholar
Sridhar, S. & Singh, N. K. 2014 Large-scale dynamo action due to 𝛼 fluctuations in a linear shear flow. Mon. Not. R. Astron. Soc. 445, 3770–3787; (SS14).CrossRefGoogle Scholar
Sridhar, S. & Subramanian, K. 2009a Shear dynamo problem: quasilinear kinematic theory. Phys. Rev. E 79, 045305.Google ScholarPubMed
Sridhar, S. & Subramanian, K. 2009b Nonperturbative quasilinear approach to the shear dynamo problem. Phys. Rev. E 80, 066315.Google Scholar
Sur, S. & Subramanian, K. 2009 Galactic dynamo action in presence of stochastic 𝛼 and shear. Mon. Not. R. Astron. Soc. 392, L6–L10.CrossRefGoogle Scholar
Vishniac, E. T. & Brandenburg, A. 1997 An incoherent 𝛼–𝛺 dynamo in accretion disks. Astrophys. J. 475, 263–274.CrossRefGoogle Scholar
Vogt, C. & Enßlin, T. A. 2005 A Bayesian view on Faraday rotation maps. Seeing the magnetic power spectra in galaxy clusters. Astron. Astrophys. 434, 67–76.CrossRefGoogle Scholar
Yousef, T. A., Heinemann, T., Schekochihin, A. A., Kleeorin, N., Rogachevskii, I., Iskakov, A. B., Cowley, S. C. & McWilliams, J. C. 2008 Generation of magnetic field by combined action of turbulence and shear. Phys. Rev. Lett. 100, 184501.CrossRefGoogle ScholarPubMed