Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-24T18:56:42.675Z Has data issue: false hasContentIssue false
  • English
  • Français

Modes of synchronisation around a near-wall oscillating cylinder in streamwise directions

Published online by Cambridge University Press:  17 April 2020

Xiaoying Ju Open the ORCID record for Xiaoying Ju [Opens in a new window] ,
Hongwei An Open the ORCID record for Hongwei An [Opens in a new window] ,
Liang Cheng  and
Feifei Tong Open the ORCID record for Feifei Tong [Opens in a new window]
Xiaoying Ju
Affiliation:
School of Engineering, University of Western Australia, 35 Stirling Highway,Crawley, WA 6009, Australia
Hongwei An
Affiliation:
School of Engineering, University of Western Australia, 35 Stirling Highway,Crawley, WA 6009, Australia
Liang Cheng*
Affiliation:
School of Engineering, University of Western Australia, 35 Stirling Highway,Crawley, WA 6009, Australia DUT–UWA Joint Research Centre, State Key Laboratory of Coastal and Offshore Engineering,Dalian University of Technology, No. 2 Linggong Road, 116024Dalian, PR China
Feifei Tong*
Affiliation:
School of Engineering, University of Western Australia, 35 Stirling Highway,Crawley, WA 6009, Australia
*
†Email addresses for correspondence: [email protected], [email protected]
†Email addresses for correspondence: [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

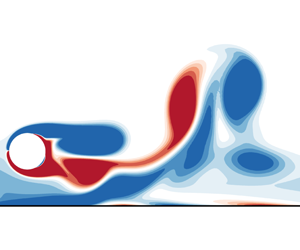
Two-dimensional direct numerical simulations of a cylinder undergoing forced streamwise oscillations in steady approaching flow are conducted over ranges of oscillation amplitude, oscillation frequency and gap distance between the cylinder and the wall at a Reynolds number of 175. The flow characteristics are found to be strongly affected by the gap distance, compared to those observed around an isolated cylinder (Tang et al., J. Fluid Mech., vol. 832, 2017, pp. 146–169). The synchronisation modes are mapped out in the parameter ranges. The existence of the plane wall leads to an increased chance of occurrence of high-order modes with the denominator being an odd number. Two new flow phenomena, namely the period doubling and transition to quasi-periodic states through cascade of period doubling within the primary synchronisation region, are observed. The interaction of the plane-wall boundary layer with vortices shed from the cylinder and the asymmetry of the flow through the gap and around the top side of the cylinder are identified as the primary physical mechanisms responsible for the observed behaviours. The influence of velocity gradient in the plane-wall boundary layer on the two new phenomena is quantified through a numerical test involving linear shear flow around an isolated cylinder. The period-doubling phenomenon occurs only when the velocity gradient is larger than a critical value. The results obtained through three-dimensional simulations suggest that the synchronisation modes identified through two-dimensional simulations are not significantly affected by the three-dimensionality of the flow over the parameter ranges covered in the present study.

JFM classification

Vortex Flows: Vortex dynamics Wakes/Jets: Shear layers
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 893 , 25 June 2020 , A8
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Al-Mdallal, Q. M., Lawrence, K. P. & Kocabiyik, S. 2007 Forced streamwise oscillations of a circular cylinder: locked-on modes and resulting fluid forces. J. Fluids Struct. 23, 681–701.CrossRefGoogle Scholar
Bearman, P. W. & Zdravkovich, M. M. 1978 Flow around a circular cylinder near a plane boundary. J. Fluid Mech. 89, 33–47.CrossRefGoogle Scholar
Blackburn, H. M. & Henderson, R. D. 1999 A study of two-dimensional flow past an oscillating cylinder. J. Fluid Mech. 385, 255–286.CrossRefGoogle Scholar
Bridge, C., Laver, K., Clukey, E. & Evans, T. 2004 Steel catenary riser touchdown point vertical interaction models. In Offshore Technology Conference. Offshore Technology Conference.Google Scholar
Cantwell, C. D., Moxey, D., Comerford, A., Bolis, A., Rocco, G., Mengaldo, G., De Grazia, D., Yakovlev, S., Lombard, J. E., Ekelschot, D. et al. 2015 Nektar + +: an open-source spectral/hp element framework. Comput. Phys. Commun. 192, 205–219.CrossRefGoogle Scholar
Farey, J. 1816 LXXIX. On a curious property of vulgar fractions. Phil. Mag. 47 (217), 385–386.CrossRefGoogle Scholar
Griffin, O. M. & Ramberg, S. E. 1976 Vortex shedding from a cylinder vibrating in line with an incident uniform flow. J. Fluid Mech. 75 (2), 257–271.CrossRefGoogle Scholar
Jiang, H., Cheng, L., Draper, S. & An, H. 2017a Two- and three-dimensional instabilities in the wake of a circular cylinder near a moving wall. J. Fluid Mech. 812, 435–462.CrossRefGoogle Scholar
Jiang, H., Cheng, L., Draper, S. & An, H. 2017b Three-dimensional wake transition for a circular cylinder near a moving wall. J. Fluid Mech. 818, 260–287.CrossRefGoogle Scholar
Jiang, H., Cheng, L., Draper, S., An, H. & Tong, F. 2016 Three-dimensional direct numerical simulation of wake transitions of a circular cylinder. J. Fluid Mech. 801, 353–391.CrossRefGoogle Scholar
Hunt, J. C. R., Wray, A. & Moin, P.1988 Eddies, stream, and convergence zones in turbulent flows. Center for Turbulence Research Report CTR-S88, pp. 193–208.Google Scholar
Karniadakis, G. E., Israeli, M. & Orszag, S. A. 1991 High-order splitting methods for the incompressible Navier–Stokes equations. J. Comput. Phys. 97, 414–443.CrossRefGoogle Scholar
Lei, C., Cheng, L., Armfield, S. W. & Kavanagh, K. 2000b Vortex shedding suppression for flow over a circular cylinder near a plane boundary. Ocean Engng 10 (27), 1109–1127.CrossRefGoogle Scholar
Lei, C., Cheng, L. & Kavanagh, K. 1999 Re-examination of the effect of a plane boundary on force and vortex shedding of a circular cylinder. J. Wind Engng Ind. Aerodyn. 80, 263–286.CrossRefGoogle Scholar
Lei, C., Cheng, L. & Kavanagh, K. 2000a A finite difference solution of the shear flow over a circular cylinder. Ocean Engng 27 (3), 271–290.CrossRefGoogle Scholar
Leontini, J. S., Lo Jacono, D. & Thompson, M. C. 2011 A numerical study of an inline oscillating cylinder in a free stream. J. Fluid Mech. 688, 551–568.CrossRefGoogle Scholar
Leontini, J. S., Lo Jacono, D. & Thompson, M. C. 2013 Wake states and frequency selection of a streamwise oscillating cylinder. J. Fluid Mech. 730, 162–192.CrossRefGoogle Scholar
Li, Z., Jaiman, R. K. & Khoo, B. C. 2017 Coupled dynamics of vortex-induced vibration and stationary wall at low Reynolds number. Phys. Fluids 29 (9), 093601.CrossRefGoogle Scholar
Li, Z., Yao, W., Yang, K., Jaiman, R. K. & Khoo, B. C. 2016 On the vortex-induced oscillations of a freely vibrating cylinder in the vicinity of a stationary plane wall. J. Fluids Struct. 65, 495–526.CrossRefGoogle Scholar
Newman, D. J. & Karniadakis, G. E. 1997 A direct numerical simulation study of flow past a freely vibrating cable. J. Fluid Mech. 344, 95–136.CrossRefGoogle Scholar
Olinger, D. & Sreenivasan, K. 1988 Nonlinear dynamics of the wake of an oscillating cylinder. Phys. Rev. Lett. 60, 797–800.CrossRefGoogle ScholarPubMed
Ongoren, A. & Rockwell, D. 1988a Flow structure from an oscillating cylinder. Part 1. Mechanisms of phase shift and recovery in the near wake. J. Fluid Mech. 191, 197–223.CrossRefGoogle Scholar
Ongoren, A. & Rockwell, D. 1988b Flow structure from an oscillating cylinder. Part 2. Mode competition in the near wake. J. Fluid Mech. 191, 225–245.CrossRefGoogle Scholar
Pikovsky, A., Rosenblum, M. & Kurths, J. 2001 Synchronization: A Universal Concept in Nonlinear Sciences. Cambridge University Press.CrossRefGoogle Scholar
Randolph, M. F., Gaudin, C., Gourvenec, S. M., White, D. J., Boylan, N. & Cassidy, M. J. 2011 Recent advances in offshore geotechnics for deep water oil and gas developments. Ocean Engng 38 (7), 818–834.CrossRefGoogle Scholar
Rao, A., Stewart, B. E., Thompson, M. C., Leweke, T. & Hourigan, K. 2011 Flows past rotating cylinders next to a wall. J. Fluids Struct. 27 (5–6), 668–679.CrossRefGoogle Scholar
Rao, A., Thompson, M. C., Leweke, T. & Hourigan, K. 2013 The flow past a circular cylinder translating at different heights above a wall. J. Fluids Struct. 41, 9–21.CrossRefGoogle Scholar
Rao, A., Thompson, M. C., Leweke, T. & Hourigan, K. 2015 Flow past a rotating cylinder translating at different gap heights along a wall. J. Fluids Struct. 57, 314–330.CrossRefGoogle Scholar
Ren, C., Cheng, L., Tong, F., Xiong, C. & Chen, T. 2019 Oscillatory flow regimes around four cylinders in a diamond arrangement. J. Fluid Mech. 877, 955–1006.CrossRefGoogle Scholar
Saffman, P. G. 1992 Vortex Dynamics. Cambridge University Press.Google Scholar
Sarpkaya, T. 2004 A critical review of the intrinsic nature of vortex-induced vibrations. J. Fluids Struct. 19 (4), 389–447.CrossRefGoogle Scholar
Stewart, B. E., Thompson, M. C., Leweke, T. & Hourigan, K. 2010 The wake behind a cylinder rolling on a wall at varying rotation rates. J. Fluid Mech. 648, 225–256.CrossRefGoogle Scholar
Tang, G., Cheng, L., Tong, F., Lu, L. & Zhao, M. 2017 Modes of synchronisation in the wake of a streamwise oscillatory cylinder. J. Fluid Mech. 832, 146–169.CrossRefGoogle Scholar
Tham, D. M. Y., Gurugubelli, P. S., Li, Z. & Jaiman, R. K. 2015 Freely vibrating circular cylinder in the vicinity of a stationary wall. J. Fluids Struct. 59, 103–128.CrossRefGoogle Scholar
Thompson, M. C., Radi, A., Rao, A., Sheridan, J. & Hourigan, K. 2014 Low-Reynolds-number wakes of elliptical cylinders: from the circular cylinder to the normal flat plate. J. Fluid Mech. 751, 570–600.CrossRefGoogle Scholar
Wang, X. K. & Tan, S. K. 2008 Near-wake flow characteristics of a circular cylinder close to a wall. J. Fluids Struct. 24, 605–627.CrossRefGoogle Scholar
Williamson, C. H. 1995 Vortex dynamics in the wake of a cylinder. In Fluid Vortices. Springer.Google Scholar
Williamson, C. H. K. 1996 Three-dimensional wake transition. J. Fluid Mech. 328, 345–407.CrossRefGoogle Scholar
Williamson, C. H. K. & Govardhan, R. 2004 Vortex-induced vibrations. Annu. Rev. Fluid Mech. 36, 413–455.CrossRefGoogle Scholar
Williamson, C. H. K. & Roshko, A. 1988 Vortex formation in the wake of an oscillating cylinder. J. Fluids Struct. 2, 355–381.CrossRefGoogle Scholar
Woo, H. 1999 A note on phase-locked states at low Reynolds numbers. J. Fluids Struct. 13, 153–158.CrossRefGoogle Scholar
Wu, J. Z., Lu, X. Y., Denny, A. G., Fan, M. & Wu, J. M. 1998 Post-stall flow control on an airfoil by local unsteady forcing. J. Fluid Mech. 371, 21–58.CrossRefGoogle Scholar
Xu, S. J., Zhou, Y. & Wang, M. H. 2006 A symmetric binary-vortex street behind a longitudinally oscillating cylinder. J. Fluid Mech. 556, 27–43.CrossRefGoogle Scholar