Hostname: page-component-586b7cd67f-l7hp2 Total loading time: 0 Render date: 2024-11-28T13:16:37.966Z Has data issue: false hasContentIssue false
  • English
  • Français

Modal stability analysis of a helical vortex tube with axial flow

Published online by Cambridge University Press:  05 December 2013

Yuji Hattori  and
Yasuhide Fukumoto
Yuji Hattori*
Affiliation:
Institute of Fluid Science, Tohoku University, Sendai 980–8577, Japan
Yasuhide Fukumoto
Affiliation:
Institute of Mathematics for Industry, Kyushu University, Fukuoka 819–0395, Japan
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The linear stability of a helical vortex tube with axial flow, which is a model of helical vortices emanating from rotating wings, is studied by modal stability analysis. At the leading order the base flow is set to the Rankine vortex with uniform velocity along the helical tube whose centreline is a helix of constant curvature and torsion. The helical vortex tube in an infinite domain, in which the free boundary condition is imposed at the surface of the tube, is our major target although the case of the rigid boundary condition is also considered in order to elucidate the effects of torsion and the combined effects of torsion and axial flow. The analysis is based on the linearized incompressible Euler equations expanded in $\epsilon $ which is the ratio of the core to curvature radius of the tube. The unstable growth rate can be evaluated using the leading-order neutral modes called the Kelvin waves with the expanded equations. At 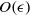$O(\epsilon )$ the instability is a linear combination of the curvature instability due to the curvature of the tube and the precessional instability due to the axial flow, both parametric instabilities appearing at the same resonance condition. At the next order 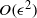$O({\epsilon }^{2} )$ not only the effects of torsion but also the combined effects of torsion and axial flow appear, a fact which has been shown only for the short-wave limit. The maximum growth rate increases for the right-handed/left-handed helix with positive/negative helicity, in which the torsion makes the period of particle motion increase. All results converge to the previous local stability results in the short-wave limit. The differences between the two cases of different boundary conditions are due to the isolated mode of the free boundary case, whose dispersion curve depends strongly on the axial flow.

JFM classification

Mathematical Foundations: General fluid mechanics Instability: Parametric instability Vortex Flows: Vortex instability
Type
Papers
Information
Journal of Fluid Mechanics , Volume 738 , 10 January 2014 , pp. 222 - 249
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Betchov, R. 1965 On the curvature and torsion of an isolated vortex filament. J. Fluid Mech. 22, 471–479.Google Scholar
Bhagwat, M. J. & Leishman, J. G. 2000 Stability analysis of helicopter rotor wakes in axial flight. J. Am. Helicopter Assoc. 45, 165–178.CrossRefGoogle Scholar
Delbende, I., Rossi, M. & Daube, O. 2012 DNS of flows with helical symmetry. Theor. Comput. Fluid Dyn. 26, 141–160.CrossRefGoogle Scholar
del Pino, C., Parras, L., Felli, M. & Fernandez-Feria, R. 2011 Structure of trailing vortices: Comparison between particle image velocimetry measurements and theoretical models. Phys. Fluids 23, 013602.Google Scholar
Eloy, C. & Le Dizès, S. 2001 Stability of the Rankine vortex in a multipolar strain field. Phys. Fluids 13, 660–676.Google Scholar
Felli, M., Camussi, R. & Di Felice, F. 2011 Mechanisms of evolution of the propeller wake in the transition and far fields. J. Fluid Mech. 682, 5–53.Google Scholar
Fukumoto, Y. & Hattori, Y. 2005 Curvature instability of a vortex ring. J. Fluid Mech. 526, 77–115.CrossRefGoogle Scholar
Fukumoto, Y. & Miyazaki, T. 1991 Three-dimensional distortions of a vortex filament with axial velocity. J. Fluid Mech. 222, 369–416.Google Scholar
Fukumoto, Y. & Okulov, V. L. 2005 The velocity field induced by a helical vortex tube. Phys. Fluids 17, 107101.Google Scholar
Gupta, B. P. & Loewy, R. G. 1974 Theoretical analysis of the aerodynamic stability of multiple, interdigitated helical vortices. AIAA J. 12, 1381–1387.Google Scholar
Hattori, Y. & Fukumoto, Y. 2003 Short-wavelength stability analysis of thin vortex rings. Phys. Fluids 15, 3151–3163.Google Scholar
Hattori, Y. & Fukumoto, Y. 2009 Short-wavelength stability analysis of a helical vortex tube. Phys. Fluids 21, 014104.Google Scholar
Hattori, Y. & Fukumoto, Y. 2010 Short-wave stability of a helical vortex tube: the effect of torsion on the curvature instability. Theor. Comput. Fluid Dyn. 24, 363–368.Google Scholar
Hattori, Y. & Fukumoto, Y. 2012 Effects of axial flow on the stability of a helical vortex tube. Phys. Fluids 24, 054102.CrossRefGoogle Scholar
Kida, S. 1981 Stability of a Steady Vortex Filament. J. Phys. Soc. Japan 51, 1655–1662.Google Scholar
Knobloch, E., Mahalov, A & Marsden, J. E. 1994 Normal forms for three-dimensional parametric instabilities in ideal hydrodynamics. Physica D 73, 49–81.CrossRefGoogle Scholar
Lacaze, L., Birbaud, A.-L. & Le Dizès, S. 2005 Elliptic instability in a Rankine vortex with axial flow. Phys. Fluids 17, 017101.Google Scholar
Lacaze, L., Ryan, K. & Le Dizès, S. 2007 Elliptic instability in a strained Batchelor vortex. J. Fluid Mech. 577, 341–361.Google Scholar
Lessen, M., Deshpande, N. V. & Hadji-Ohanes, B. 1973 Stability of a potential vortex with a non-rotating and rigid-body rotating top-hat jet core. J. Fluid Mech. 60, 459–465.Google Scholar
Loiseleux, T, Chomaz, J. M. & Huerre, P. 1998 The effect of swirl on jets and wakes: linear instability of the Rankine vortex with axial flow. Phys. Fluids 10, 375–394.Google Scholar
Lucas, D. & Dritschel, D. G. 2009 A family of helically symmetric vortex equilibria. J. Fluid Mech. 634, 245–268.Google Scholar
Moore, D. W. & Saffman, P. G. 1972 The motion of a vortex filament with axial flow. Phil. Trans. R. Soc. Lond. A 272, 403–429.Google Scholar
Okulov, V. L. 1995 The velocity field induced by vortex filaments with cylindrical and conic supporting surface. Russ. J. Eng. Thermophys. 5, 63–75.Google Scholar
Okulov, V. L. 2004 On the stability of multiple helical vortices. J. Fluid Mech. 521, 319–342.Google Scholar
Okulov, V. L. & Sørensen, J. N. 2007 Stability of helical tip vortices in a rotor far wake. J. Fluid Mech. 576, 1–25.Google Scholar
Spalart, P. R. 1998 Airplane trailing vortices. Annu. Rev. Fluid Mech. 30, 107–138.Google Scholar
Widnall, S. E. 1972 The stability of a helical vortex filament. J. Fluid Mech. 54, 641–663.CrossRefGoogle Scholar
Widnall, S. E. & Tsai, C.-Y. 1977 The instability of the thin vortex ring of constant vorticity. Phil. Trans. R. Soc. Lond. A 287, 273–305.Google Scholar