Hostname: page-component-745bb68f8f-d8cs5 Total loading time: 0 Render date: 2025-01-11T08:57:39.076Z Has data issue: false hasContentIssue false
  • English
  • Français

Mixing by bubble-induced turbulence

Published online by Cambridge University Press:  10 July 2015

Elise Alméras ,
Frédéric Risso ,
Véronique Roig ,
Sébastien Cazin ,
Cécile Plais  and
Frédéric Augier
Elise Alméras
Affiliation:
Institut de Mécanique des Fluides de Toulouse, Université de Toulouse and CNRS, Allée C. Soula, 31400 Toulouse, France IFP Energies nouvelles, Rond-point de l’échangeur de Solaize, BP 3, 69360 Solaize, France
Frédéric Risso*
Affiliation:
Institut de Mécanique des Fluides de Toulouse, Université de Toulouse and CNRS, Allée C. Soula, 31400 Toulouse, France
Véronique Roig
Affiliation:
Institut de Mécanique des Fluides de Toulouse, Université de Toulouse and CNRS, Allée C. Soula, 31400 Toulouse, France
Sébastien Cazin
Affiliation:
Institut de Mécanique des Fluides de Toulouse, Université de Toulouse and CNRS, Allée C. Soula, 31400 Toulouse, France
Cécile Plais
Affiliation:
IFP Energies nouvelles, Rond-point de l’échangeur de Solaize, BP 3, 69360 Solaize, France
Frédéric Augier
Affiliation:
IFP Energies nouvelles, Rond-point de l’échangeur de Solaize, BP 3, 69360 Solaize, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

This work reports an experimental investigation of the dispersion of a low-diffusive dye within a homogeneous swarm of high-Reynolds-number rising bubbles at gas volume fractions ${\it\alpha}$ ranging from 1 % to 13 %. The capture and transport of dye within bubble wakes is found to be negligible and the mixing turns out to result from the bubble-induced turbulence. It is described well by a regular diffusion process. The diffusion coefficient corresponding to the vertical direction is larger than that corresponding to the horizontal direction, owing to the larger intensity of the liquid fluctuations in the vertical direction. Two regimes of diffusion have been identified. At low gas volume fraction, the diffusion time scale is given by the correlation time of the bubble-induced turbulence and the diffusion coefficients increase roughly as 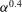${\it\alpha}^{0.4}$. At large gas volume fraction, the diffusion time scale is imposed by the time interval between two bubbles and the diffusion coefficients become almost independent of ${\it\alpha}$. The transition between the two regimes occurs sooner in the horizontal direction (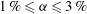$1\,\%\leqslant {\it\alpha}\leqslant 3\,\%$) than in the vertical direction (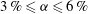$3\,\%\leqslant {\it\alpha}\leqslant 6\,\%$). Physical models based on the hydrodynamic properties of the bubble swarm are introduced and guidelines for practical applications are suggested.

JFM classification

Drops and Bubbles: Bubble dynamics Multiphase and Particle-laden Flows: Gas/liquid flow
Type
Papers
Information
Journal of Fluid Mechanics , Volume 776 , 10 August 2015 , pp. 458 - 474
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abbas, M., Billet, A. M. & Roig, V.2009 Experiments on mass transfer and mixing in a homogeneous bubbly flow. In Proceedings of the 6th International Symposium on Turbulence, Heat and Mass Transfer, Rome, Italy, pp. 815–817.Google Scholar
Alméras, E.2014 Étude des propriétés de transport et de mélange dans les écoulements à bulles, PhD thesis, Université de Toulouse.Google Scholar
Bouche, E., Cazin, S., Roig, V. & Risso, F. 2013 Mixing in a swarm of bubbles rising in a confined cell measured by mean of PLIF with two different dyes. Exp. Fluids 54:1552.CrossRefGoogle Scholar
Bouche, E., Roig, V., Risso, F. & Billet, A.-M. 2014 Homogeneous swarm of high-Reynolds-number bubbles rising within a thin gap. Part 2. Liquid dynamics. J. Fluid Mech. 758, 508–521.CrossRefGoogle Scholar
Colombet, D., Legendre, D., Cockx, A., Guiraud, G., Risso, F., Daniel, C. & Galinat, S. 2011 Experimental study of mass transfer in a dense bubble swarm. Chem. Engng Sci. 66, 3432–3440.CrossRefGoogle Scholar
Colombet, D., Legendre, D., Risso, F., Cockx, A. & Guiraud, P. 2014 Dynamics and mass transfer of rising bubbles in an homogeneous swarm at large gas volume fraction. J. Fluid Mech. 763, 254–285.CrossRefGoogle Scholar
Combest, D. P., Ramachandran, A. & Dudukovic, M. P. 2011 On the gradient diffusion hypothesis and passive scalar transport in turbulent flows. Ind. Engng Chem. Res. 50, 8817–8823.CrossRefGoogle Scholar
Corrsin, S. 1963 Estimates of the relations between Eulerian and Lagrangian scales in large Reynolds number turbulence. J. Atmos. Sci. 20, 115–119.2.0.CO;2>CrossRefGoogle Scholar
Lance, M. & Bataille, J. 1991 Turbulence in the liquid phase of a uniform bubbly air–water flow. J. Fluid Mech. 222, 95–118.CrossRefGoogle Scholar
Mareuge, I. & Lance, M.1995 Bubble-induced dispersion of a passive scalar in bubbly flows. In Proceedings of the 2nd International Conference on Multiphase Flow, Kyoto, Japan, PT1-3–8.Google Scholar
Martínez-Mercado, J., Gómez, D. C., van Gils, D., Sun, C. & Lohse, D. 2010 On bubble clustering and energy spectra in pseudo-turbulence. J. Fluid Mech. 650, 287–306.CrossRefGoogle Scholar
Martínez-Mercado, J., Palacios-Morales, C. A. & Zenit, R. 2007 Measurement of pseudoturbulence intensity in monodispersed bubbly liquids for $10<Re<500$ . Phys. Fluids 19, 103302.CrossRefGoogle Scholar
Mendez-Diaz, J. C., Serrano-García, J. C. & Zenit, R. 2013 Power spectral distributions of pseudo-turbulent bubbly flows. Phys. Fluids 25, 043303.CrossRefGoogle Scholar
Radl, S. & Khinast, J. 2010 Multiphase flow and mixing in dilute bubble swarms. AIChE J. 56, 2421–2445.CrossRefGoogle Scholar
Riboux, G., Legendre, D. & Risso, F. 2013 A model of bubble-induced turbulence based on large-scale wake interactions. J. Fluid Mech. 719, 196–212.CrossRefGoogle Scholar
Riboux, G., Risso, F. & Legendre, D. 2010 Experimental characterization of the agitation generated by bubbles rising at high Reynolds number. J. Fluid Mech. 643, 509–559.CrossRefGoogle Scholar
Risso, F. & Ellingsen, K. 2002 Velocity fluctuations in a homogeneous dilute dispersion of high-Reynolds-number rising bubbles. J. Fluid Mech. 453, 395–410.CrossRefGoogle Scholar
Risso, F., Roig, V., Amoura, Z., Riboux, G. & Billet, A.-M. 2008 Wake attenuation in large Reynolds number dispersed two-phase flows. Phil. Trans. R. Soc. Lond. A 366, 2177–2190.Google ScholarPubMed
Socolofsky, S. A. & Jirka, G. H. 2005 CVEN 489-501: Special Topics in Mixing and Transport Processes in the Environment, 5th edn, pp. 1–172. Texas A&M University.Google Scholar
Taylor, G. I. 1922 Diffusion by continuous movements. Proc. Lond. Math. Soc. s2–20 (1), 362–387.Google Scholar