Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-28T14:18:40.143Z Has data issue: false hasContentIssue false
  • English
  • Français

Mechanisms of dynamic wetting failure in the presence of soluble surfactants

Published online by Cambridge University Press:  21 July 2017

Chen-Yu Liu ,
Marcio S. Carvalho Open the ORCID record for Marcio S. Carvalho [Opens in a new window]  and
Satish Kumar Open the ORCID record for Satish Kumar [Opens in a new window]
Chen-Yu Liu
Affiliation:
Department of Chemical Engineering and Materials Science, University of Minnesota, Minneapolis, MN 55455, USA
Marcio S. Carvalho*
Affiliation:
Department of Mechanical Engineering, Pontificia Universidade Católica do Rio de Janeiro, Rio de Janeiro, RJ 22451-041, Brazil
Satish Kumar*
Affiliation:
Department of Chemical Engineering and Materials Science, University of Minnesota, Minneapolis, MN 55455, USA
*
†Email addresses for correspondence: [email protected], [email protected]
†Email addresses for correspondence: [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

A hydrodynamic model and flow visualization experiments are used to understand the mechanisms through which soluble surfactants can influence the onset of dynamic wetting failure. In the model, a Newtonian liquid displaces air in a rectangular channel in the absence of inertia. A Navier-slip boundary condition and constant contact angle are used to describe the dynamic contact line, and surfactants are allowed to adsorb to the interface and moving channel wall (substrate). The Galerkin finite element method is used to calculate steady states and identify the critical capillary number 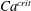$Ca^{crit}$ at which wetting failure occurs. It is found that surfactant solubility weakens the influence of Marangoni stresses, which tend to promote the onset of wetting failure. Adsorption of surfactants to the substrate can delay the onset of wetting failure due to the emergence of Marangoni stresses that thicken the air film near the dynamic contact line. The experiments indicate that 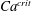$Ca^{crit}$ increases with surfactant concentration. For the more viscous solutions used, this behaviour can largely be explained by accounting for changes to the mean surface tension and static contact angle produced by surfactants. For the lowest-viscosity solution used, comparison between the model predictions and experimental observations suggests that other surfactant-induced phenomena such as Marangoni stresses may play a more important role.

JFM classification

Materials Processing Flows: Coating Interfacial Flows (free surface): Contact lines Interfacial Flows (free surface): Interfacial Flows (free surface)
Type
Papers
Information
Journal of Fluid Mechanics , Volume 825 , 25 August 2017 , pp. 677 - 703
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alvarez, N. J., Vogus, D. R., Walker, L. M. & Anna, S. L. 2012 Using bulk convection in a microtensiometer to approach kinetic-limited surfactant dynamics at fluid–fluid interfaces. J. Colloid Interface Sci. 372 (1), 183–191.Google Scholar
Beacham, D. R., Matar, O. K. & Craster, R. V. 2009 Surfactant-enhanced rapid spreading of drops on solid surfaces. Langmuir 25 (24), 14174–14181.Google Scholar
Benkreira, H. 2004 The effect of substrate roughness on air entrainment in dip coating. Chem. Engng Sci. 59 (13), 2745–2751.Google Scholar
Benkreira, H. & Ikin, J. B. 2010 Dynamic wetting and gas viscosity effects. Chem. Engng Sci. 65 (5), 1790–1796.Google Scholar
Benkreira, H. & Khan, M. I. 2008 Air entrainment in dip coating under reduced air pressures. Chem. Engng Sci. 63 (2), 448–459.Google Scholar
Bera, B., Duits, M. H. G., Cohen Stuart, M. A., van den Ende, D. & Mugele, F. 2016 Surfactant induced autophobing. Soft Matt. 12, 4562–4571.Google Scholar
Blake, T. D.1998 Coating processes. US patent 5792515.Google Scholar
Blake, T. D. 2006 The physics of moving wetting lines. J. Colloid Interface Sci. 299 (1), 1–13.Google Scholar
Blake, T. D., Fernandez-Toledano, J.-C., Doyen, G. & De Coninck, J. 2015 Forced wetting and hydrodynamic assist. Phys. Fluids 27 (11), 112101.Google Scholar
Bose, A. 1993 Wetting by solutions. In Wettability (ed. Berg, J. C.), pp. 149–181. Marcel Dekker.Google Scholar
Burley, R. & Jolly, R. P. S. 1984 Entrainment of air into liquids by a high speed continuous solid surface. Chem. Engng Sci. 39 (9), 1357–1372.Google Scholar
Burley, R. & Kennedy, B. S. 1976 An experimental study of air entrainment at a solid/liquid/gas interface. Chem. Engng Sci. 31, 901–911.Google Scholar
Campana, D. M., Di Paolo, J. & Saita, F. A. 2004 A 2-D model of Rayleigh instability in capillary tubes – surfactant effects. Intl J. Multiphase Flow 30, 431–454.Google Scholar
Campana, D. M. & Saita, F. A. 2006 Numerical analysis of the Rayleigh instability in capillary tubes: the influence of surfactant solubility. Phys. Fluids 18 (2), 022104.Google Scholar
Campana, D. M., Ubal, S., Giavedoni, M. D. & Saita, F. A. 2011 A deeper insight into the dip coating process in the presence of insoluble surfactants: a numerical analysis. Phys. Fluids 23 (5), 052102.CrossRefGoogle Scholar
Cantu, L., Corti, M., Degiorgio, V., Hoffmann, H. & Ulbricht, W. 1987 Nonionic micelles in mixed water–glycerol solvent. J. Colloid Interface Sci. 116 (2), 384–389.CrossRefGoogle Scholar
Chan, T. S., Srivastava, S., Marchand, A., Andreotti, B., Biferale, L., Toschi, F. & Snoeijer, J. H. 2013 Hydrodynamics of air entrainment by moving contact lines. Phys. Fluids 25 (7), 074105.Google Scholar
Chang, C.-H. & Franses, E. I. 1992 Modified Langmuir–Hinshelwood kinetics for dynamic adsorption of surfactants at the air/water interface. Colloids Surf. 69, 189–201.Google Scholar
Chang, C.-H. & Franses, E. I. 1995 Adsorption dynamics of surfactants at the air/water interface: a critical review of mathematical models, data, and mechanisms. Colloids Surf., A 100, 1–45.Google Scholar
Cheikh, C. & Koper, G. 2003 Stick–slip transition at the nanometer scale. Phys. Rev. Lett. 91 (15), 156102.Google Scholar
Cohu, O. & Benkreira, H. 1998 Entrainment of air by a solid surface plunging into a non-Newtonian liquid. AIChE J. 44 (11), 2360–2368.Google Scholar
Craster, R. V. & Matar, O. K. 2009 Dynamics and stability of thin liquid films. Rev. Mod. Phys. 81 (3), 1131–1198.Google Scholar
Deryagin, B. M. & Levi, S. M. 1964 Film Coating Theory: Physical Chemistry of Coating. Focal Press.Google Scholar
Dussan, V. E. B. 1976 The moving contact line: the slip boundary condition. J. Fluid Mech. 77 (4), 665–684.CrossRefGoogle Scholar
Eggers, J. 2005 Existence of receding and advancing contact lines. Phys. Fluids 17 (8), 082106.Google Scholar
Fainerman, V. B., Makievski, A. V. & Miller, R. 1993 The measurement of dynamic surface tensions of highly viscous liquids by the maximum bubble pressure method. Colloids Surf. A 75 (6), 229–235.Google Scholar
Fallest, D. W., Lichtenberger, A. M., Fox, C. J. & Daniels, K. E. 2010 Fluorescent visualization of a spreading surfactant. New J. Phys. 12, 073029.Google Scholar
Frank, B. & Garoff, S. 1995 Origins of the complex motion of advancing surfactant solutions. Langmuir 11 (18), 87–93.Google Scholar
Gutoff, E. B. & Kendrick, C. E. 1982 Dynamic contact angles. AlChE J. 28 (3), 459–466.Google Scholar
Henry, C. L., Neto, C., Evans, D. R., Biggs, S. & Craig, V. S. J. 2004 The effect of surfactant adsorption on liquid boundary slippage. Physica A 339 (1), 60–65.Google Scholar
Huh, C. & Scriven, L. E. 1971 Hydrodynamic model of steady movement of a solid/liquid/fluid contact line. J. Colloid Interface Sci. 35 (1), 85–101.CrossRefGoogle Scholar
Jacqmin, D. 2004 Onset of wetting failure in liquid–liquid systems. J. Fluid Mech. 517, 209–228.Google Scholar
Jensen, O. E. & Grotberg, J. B. 1993 The spreading of heat or soluble surfactant along a thin liquid film. Phys. Fluids A 5 (1), 58–68.Google Scholar
Karapetsas, G., Craster, R. V. & Matar, O. K. 2011 On surfactant-enhanced spreading and superspreading of liquid drops on solid surfaces. J. Fluid Mech. 670, 5–37.Google Scholar
Kistler, S. F. 1993 Hydrodynamics of wetting. In Wettability (ed. Berg, J. C.), pp. 311–429. Marcel Dekker.Google Scholar
Kumar, N., Maldarelli, C. & Couzis, A. 2006 An infrared spectroscopy study of the hydrogen bonding and water restructuring as a trisiloxane superspreading surfactant adsorbs onto an aqueous-hydrophobic surface. Colloids Surf. A 277, 98–106.Google Scholar
Kunert, C. & Harting, J. 2008 On the effect of surfactant adsorption and viscosity change on apparent slip in hydrophobic microchannels. Prog. Comput. Fluid Dyn. 8 (1–4), 197–205.Google Scholar
Liu, C.-Y.2017 Onset of dynamic wetting failure in the presence of surfactants. PhD thesis, University of Minnesota.Google Scholar
Liu, C.-Y., Vandre, E., Carvalho, M. S. & Kumar, S. 2016a Dynamic wetting failure and hydrodynamic assist in curtain coating. J. Fluid Mech. 808, 290–315.Google Scholar
Liu, C.-Y., Vandre, E., Carvalho, M. S. & Kumar, S. 2016b Dynamic wetting failure in surfactant solutions. J. Fluid Mech. 789, 285–309.Google Scholar
Lowndes, J. 1980 The numerical simulation of the steady movement of a fluid meniscus in a capillary tube. J. Fluid Mech. 101 (3), 631–646.Google Scholar
Marston, J. O., Hawkins, V., Decent, S. P. & Simmons, M. J. H. 2009 Influence of surfactant upon air entrainment hysteresis in curtain coating. Exp. Fluids 46, 549–558.Google Scholar
Matar, O. K. & Craster, R. V. 2009 Dynamics and stability of thin liquid films. Rev. Mod. Phys. 81 (3), 1131–1198.Google Scholar
Miyamoto, K. & Katagiri, Y. 1997 Curtain coating. In Liquid Film Coating (ed. Kistler, S. & Schweizer, P. M.), pp. 463–494. Chapman & Hall.Google Scholar
Ramé, E. 2001 The spreading of surfactant-laden liquids with surfactant transfer through the contact line. J. Fluid Mech. 440, 205–234.Google Scholar
Ruiz, C. C., Diaz-Lopez, L. & Aguiar, J. 2008 Micellization of sodium dodecyl sulfate in glycerol aqueous mixtures. J. Dispersion Sci. Technol. 29 (2), 266–273.Google Scholar
Sbragaglia, M., Sugiyama, K. & Biferale, L. 2008 Wetting failure and contact line dynamics in a Couette flow. J. Fluid Mech. 614, 471–493.Google Scholar
Schunk, P. R. & Scriven, L. E. 1997 Surfactant effects in coating processes. In Liquid Film Coating (ed. Kistler, S. & Schweizer, P. M.), pp. 495–536. Chapman & Hall.Google Scholar
Shikhmurzaev, Y. D. 1997 Moving contact lines in liquid/liquid/solid systems. J. Fluid Mech. 334, 211–249.Google Scholar
Sibley, D. N., Nold, A. & Kalliadasis, S. 2015 The asymptotics of the moving contact line: cracking an old nut. J. Fluid Mech. 764, 445–462.Google Scholar
Snoeijer, J. H. & Andreotti, B. 2013 Moving contact lines: scales, regimes, and dynamical transitions. Annu. Rev. Fluid Mech. 45, 269–292.Google Scholar
Sochi, T. 2011 Slip at fluid–solid interface. Polym. Rev. 51 (4), 309–340.Google Scholar
Sprittles, J. E. 2015 Air entrainment in dynamic wetting: Knudsen effects and the influence of ambient air pressure. J. Fluid Mech. 769, 444–481.Google Scholar
Sprittles, J. E. 2017 Kinetic effects in dynamic wetting. Phys. Rev. Lett. 118 (11), 114502.Google Scholar
Staggemeier, B. A., Collier, T. O., Prazen, B. J. & Synovec, R. E. 2005 Effect of solution viscosity on dynamic surface tension detection. Anal. Chim. Acta 534, 79–87.Google Scholar
Sui, Y., Ding, H. & Spelt, P. D. 2014 Numerical simulations of flows with moving contact lines. Annu. Rev. Fluid Mech. 46, 97–119.Google Scholar
Swanson, E. R., Strickland, S. L., Shearer, M. & Daniels, K. E. 2015 Surfactant spreading on a thin liquid film: reconciling models and experiments. J. Engng Maths 94, 63–79.Google Scholar
Takisawa, N., Thomason, M., Bloor, D. M. & Wyn-Jones, E. 1993 Ultrasonic relaxation and electrochemical studies of the micellization of sodium decyl sulfate and decyltrimethylammonium bromide in glycerol/water mixtures. J. Colloid Interface Sci. 157 (1), 77–81.Google Scholar
Tricot, Y.-M. 1997 Surfactants: static and dynamic surface tension. In Liquid Film Coating (ed. Kistler, S. & Schweizer, P. M.), pp. 99–136. Chapman & Hall.Google Scholar
Vandre, E. A.2013 Onset of dynamic wetting failure: the mechanics of high-speed fluid displacement. PhD thesis, University of Minnesota.Google Scholar
Vandre, E., Carvalho, M. S. & Kumar, S. 2012 Delaying the onset of dynamic wetting failure through meniscus confinement. J. Fluid Mech. 707, 496–520.Google Scholar
Vandre, E., Carvalho, M. S. & Kumar, S. 2013 On the mechanism of wetting failure during fluid displacement along a moving substrate. Phys. Fluids 25 (10), 102103.CrossRefGoogle Scholar
Vandre, E., Carvalho, M. S. & Kumar, S. 2014 Characteristics of air entrainment during dynamic wetting failure along a planar substrate. J. Fluid Mech. 747, 119–140.Google Scholar
Weinstein, S. J. & Ruschak, K. J. 2004 Coating flows. Annu. Rev. Fluid Mech. 36 (1), 29–53.Google Scholar
Zhu, Y. & Granick, S. 2002 No-slip boundary condition switches to partial slip when fluid contains surfactant. Langmuir 18 (26), 10058–10063.Google Scholar